Simulation investigation on spatial deflection of multiples fractures of multistage perforation clusters in hydraulic fracturing
-
摘要:
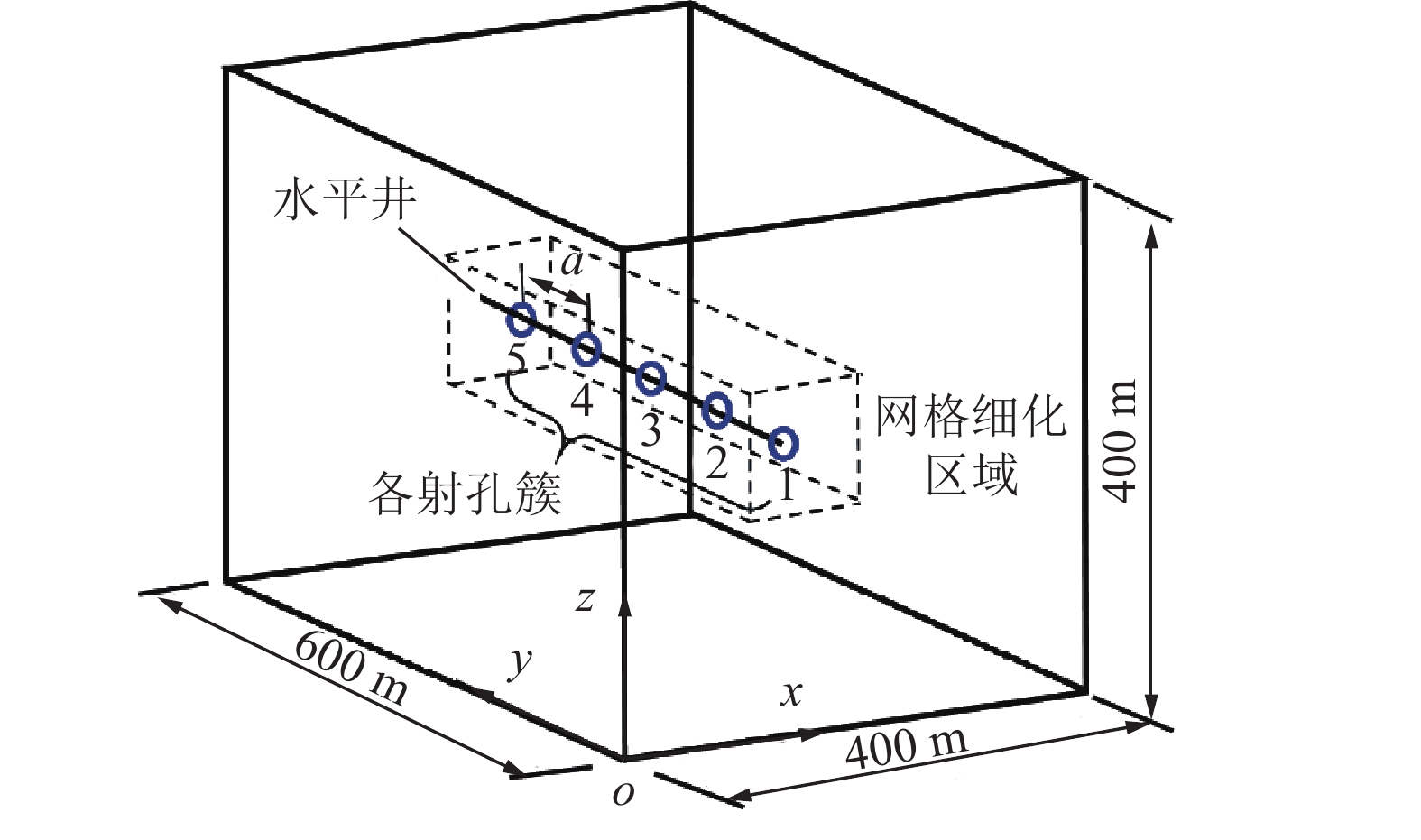
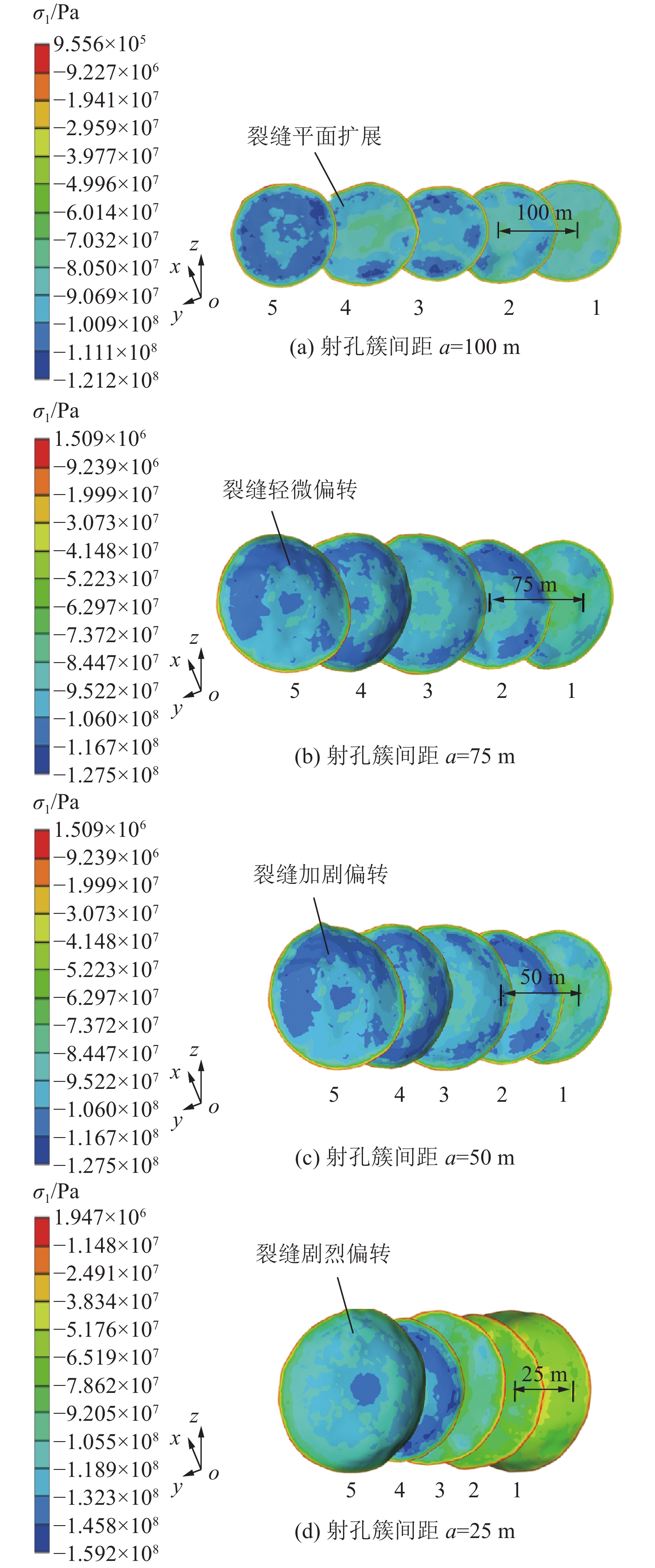
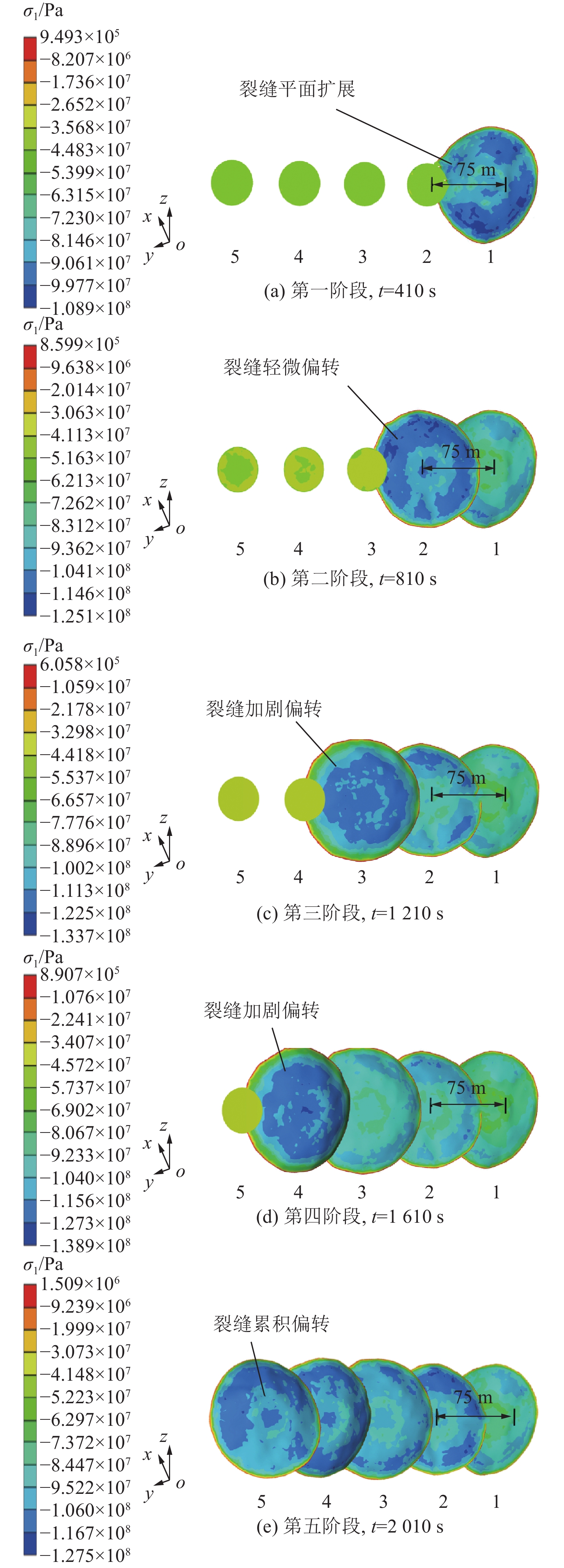
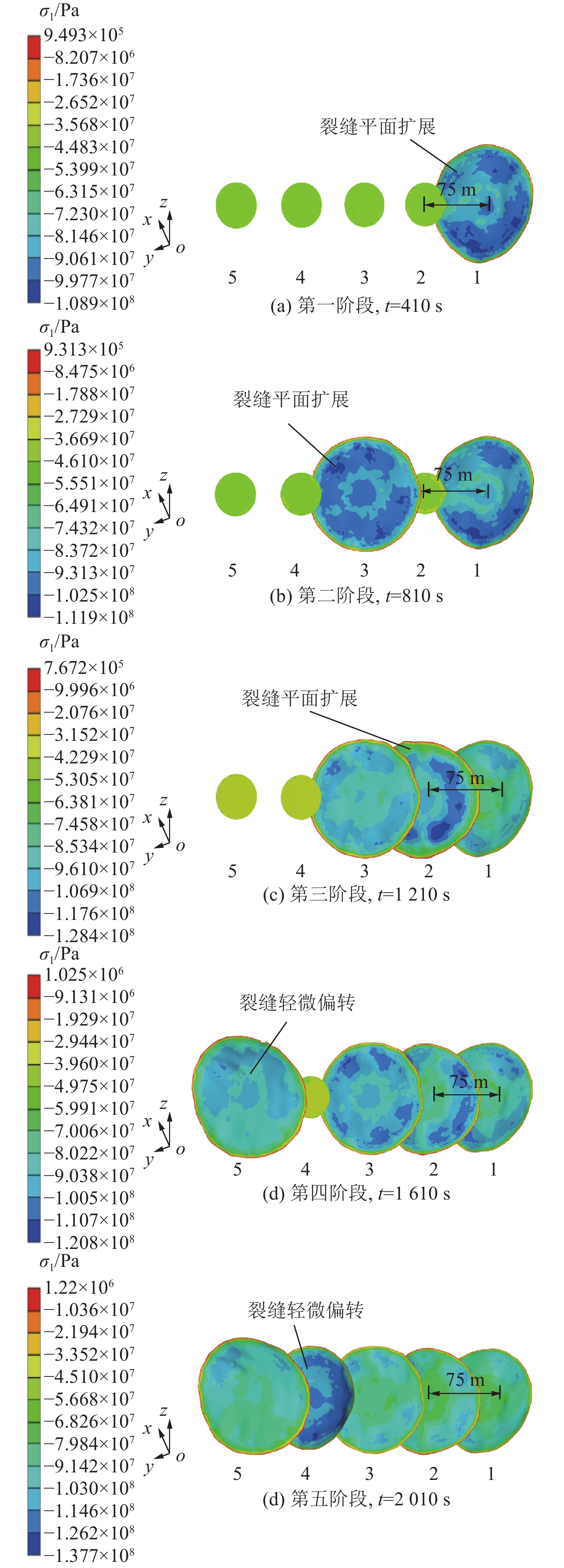
深部致密油气储层水力压裂工程形成复杂缝网形态是影响油气采收率的关键因素，需要准确评估和优化压裂裂缝扩展行为。水平井多射孔簇分段压裂涉及储层和孔隙−裂隙内流体之间的热扩散、流体流动与岩体基质变形，热扩散效应和多物理场耦合作用是深部致密岩体压裂的典型特征；同时，压裂缝网扩展与裂缝间的扰动作用有关，压裂工艺中的射孔簇间距、起裂顺序等造成平行裂缝发生不同程度的非稳定扩展。理解多物理场耦合、裂缝间扰动等内外因素的影响机制，对有效评估压裂缝网具有重要意义。综合考虑深部储层的热−流−固耦合效应，研究水力压裂缝网三维扩展之间的应力阴影效应和多裂缝扰动偏转行为。研究建立水平井分段压裂的工程尺度三维数值模型，利用典型工况计算分析了压裂裂缝三维扩展的热扩散效应影响、不同射孔簇间距以及不同压裂方案（顺序、同步、交替压裂）下裂缝网络的扩展扰动行为。结果表明：深部致密油气储层压裂裂缝扩展引起的应力扰动区域在多裂缝中存在叠加、覆盖行为，形成应力阴影效应、造成裂缝空间偏转；水平井多射孔簇间距的减小，将增大应力阴影区，加剧裂缝间相互干扰；相比多射孔簇顺序压裂，同步压裂将增大应力阴影区，交替压裂可减小应力阴影区，交替压裂成为缓解压裂缝网三维扩展扰动、优化空间缝网形态的有效方案；深部致密油气储层岩体裂缝内的压裂液与岩体基质进行热交换，各压裂方案下的裂缝扩展面积、体积均有提升，表明热效应对裂缝扩展有促进作用，成为影响压裂裂缝扩展的重要因素。
Abstract:The morphology of complex fracture network in hydraulic fracturing engineering in deep tight oil and gas reservoir is a crucial factor affecting oil and gas recovery, and it is necessary to accurately evaluate and optimize the fracture propagation behavior. Multistage fracturing of horizontal wells with multiple perforation clusters involves thermal diffusion, fluid flow and deformation of rock matrix between the reservoir and fluid in pores and fractures. Thermal diffusion effect and multi-physical field coupling are typical characteristics of fracturing in deep tight rock reservoirs. At the same time, the propagation of fracture network is related to the disturbance between adjacent fractures. The perforation clusters spacing and initiation sequence in fracturing process will lead to different degrees of unstable propagation of parallel fractures. It is of great significance to understand the influence mechanisms of internal and external factors for the effective evaluation of fracture networks, such as the coupling of multiple physical fields and fractures disturbance. The thermal-fluid-solid coupling effect in deep reservoir was considered comprehensively to investigate the stress shadow effect and the disturbance deflection behaviors of multiple fractures in three-dimensional (3D) propagation process of hydraulic fracture network. 3D engineering scale numerical model for multistage fracturing in horizontal wells was established. The influence of thermal diffusion effect on 3D fracture, and the propagation disturbance behaviors of 3D fracture network under different perforation cluster spaces and different fracturing scenarios (sequential, simultaneous and alternate fracturing) were analyzed in typical engineering conditions. The results shown that, the stress disturbance region caused by fracture propagation in deep tight oil and gas reservoirs had superposition and overlaying behaviors in multiple fractures, forming a stress shadow effect and spatial deflection of fractures. The decrease of space between multiple perforation clusters in horizontal wells would increase the stress shadow areas and aggravate the mutual interaction between fractures. Compared with the sequential fracturing of multiple perforation clusters, the simultaneous fracturing would increase the stress shadow areas, and the alternate fracturing may conversely reduce the stress shadow areas to alleviate 3D propagation disturbance of fracture network to form an effective scheme for optimizing the spatial morphology of fracturing fracture network. The heat transfers between the fracturing fluid and the rock matrix in deep tight rock reservoirs, and the fracture propagation area and volume under each fracturing scheme were significantly enhanced, indicating that the thermal effect promoted fracture propagation and became an important factor affecting the fracture propagation.
-
-
![]()
图 5 顺序压裂下裂缝区域局部网格重划分
Figure 5. Local mesh refinement around fracture domains in sequential fracturing
![]()
图 6 顺序压裂下最终缝网形态和应力场结果
Figure 6. Final morphology of fracture network and stress results in sequential fracturing
![]()
图 7 顺序压裂下裂缝扩展和应力场演化结果(射孔簇间距a=75 m)
Figure 7. Dynamic propagation of fracture network and evolution of stress results in sequential fracturing (Perforation cluster space a=75 m)
![]()
图 8 同步顺序压裂下裂缝扩展和应力场演化结果
Figure 8. Dynamic propagation of fracture network and evolution of stress results in simultaneous fracturing
![]()
图 9 交替压裂下裂缝扩展和应力场演化结果(射孔簇间距a=75 m)
Figure 9. Dynamic propagation of fracture network and evolution of stress results in alternate fracturing (Perforation cluster space a=75 m)
![]()
图 11 不同压裂方案下压裂裂缝面积对比
Figure 11. Comparison of fracture areas in different fracturing scenarios
![]()
图 12 不同压裂方案下压裂裂缝体积对比
Figure 12. Comparison of fracture volumes in different fracturing scenarios
表 1 模型基本物理参数
Table 1 Basic physical parameters of the model
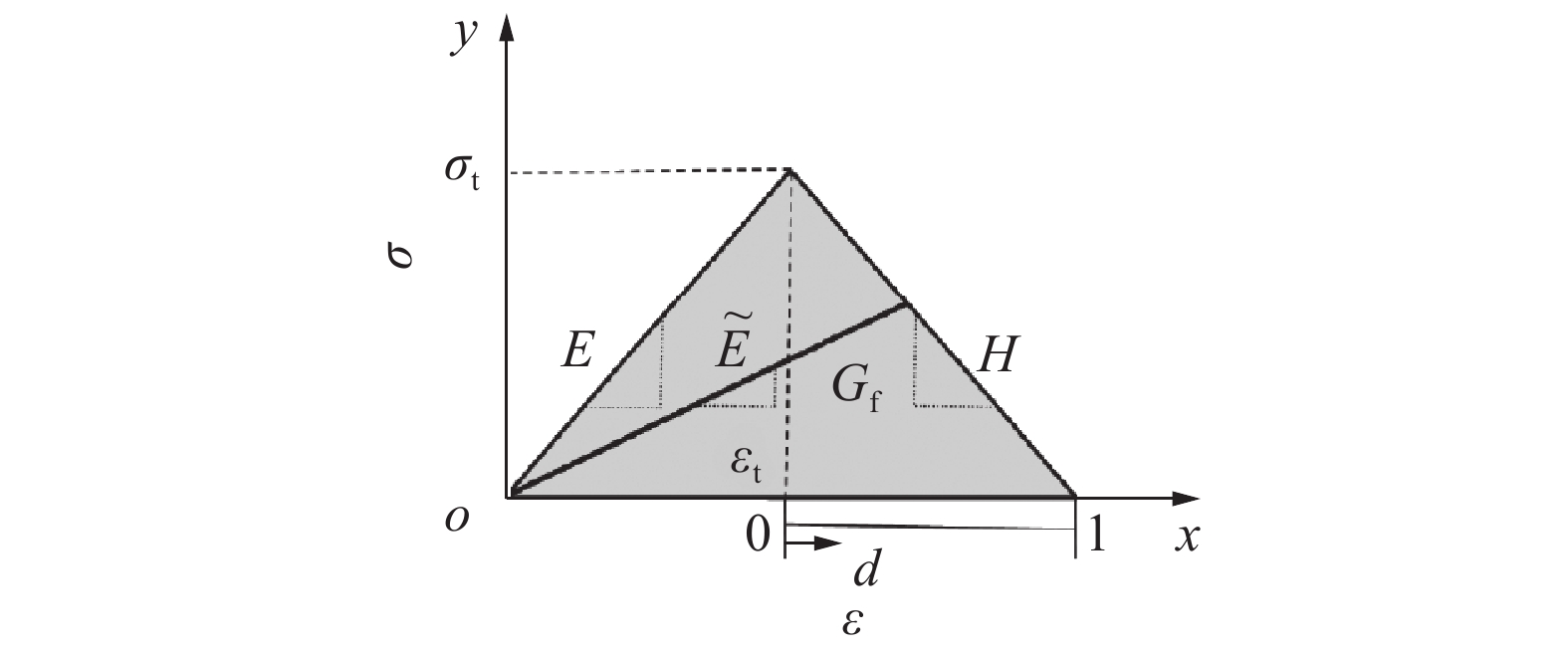
参数 取值 垂直地应力（z）Sv/MPa 40 水平最小地应力（x）Sh/MPa 46 水平最大地应力（y）SH/MPa 60 流体注入速率 Q/(m3·s-1) 0.5 滤失参数C/($ {{\rm{m}}^3} \cdot {{\rm{s}}^{ - \frac{1}{2}}}$) 1.0×10−16 孔隙压力 Ps/MPa 10 Biot系数 α 0.75 弹性模量 E/GPa 31 Poisson比 v 0.22 渗透率 k/m2 0.5×10−19 孔隙率 φ 0.05 运动黏性系数 μn/(Pa·s) 1.67×10−3 裂隙流体体积模量 $ K_{\rm f}^{\rm {f r}}$/MPa 2 000 拉伸强度 $\sigma_{\rm{t}} $/MPa 5.26 断裂能 $ G_{\rm{f}} $/(N·m) 165 
下载: 导出CSV
表 2 顺序和同步压裂的各阶段持续时间和总时间
Table 2 Duration and total time of multiple fracturing stages for sequential and alternate fracturing
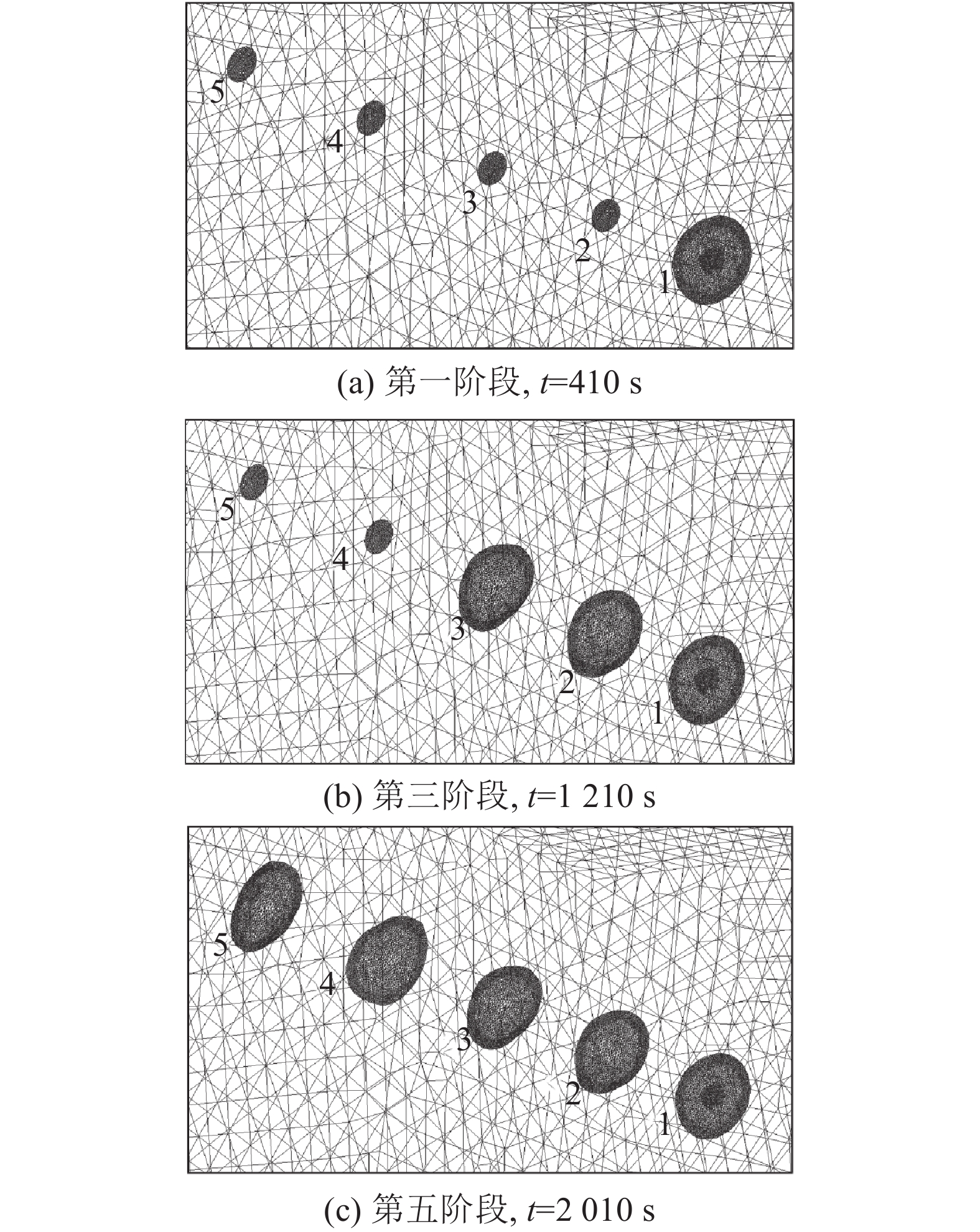
压裂阶段 持续时间$ \Delta t $/s 总时间$ t $/s 初始平衡阶段 10 10 第一阶段 400 410 第二阶段 400 810 第三阶段 400 1 210 第四阶段 400 1 610 第五阶段 400 2 010
下载: 导出CSV
表 3 各压裂方案射孔簇间距设置
Table 3 Perforation cluster spaces for fracturing scenarios
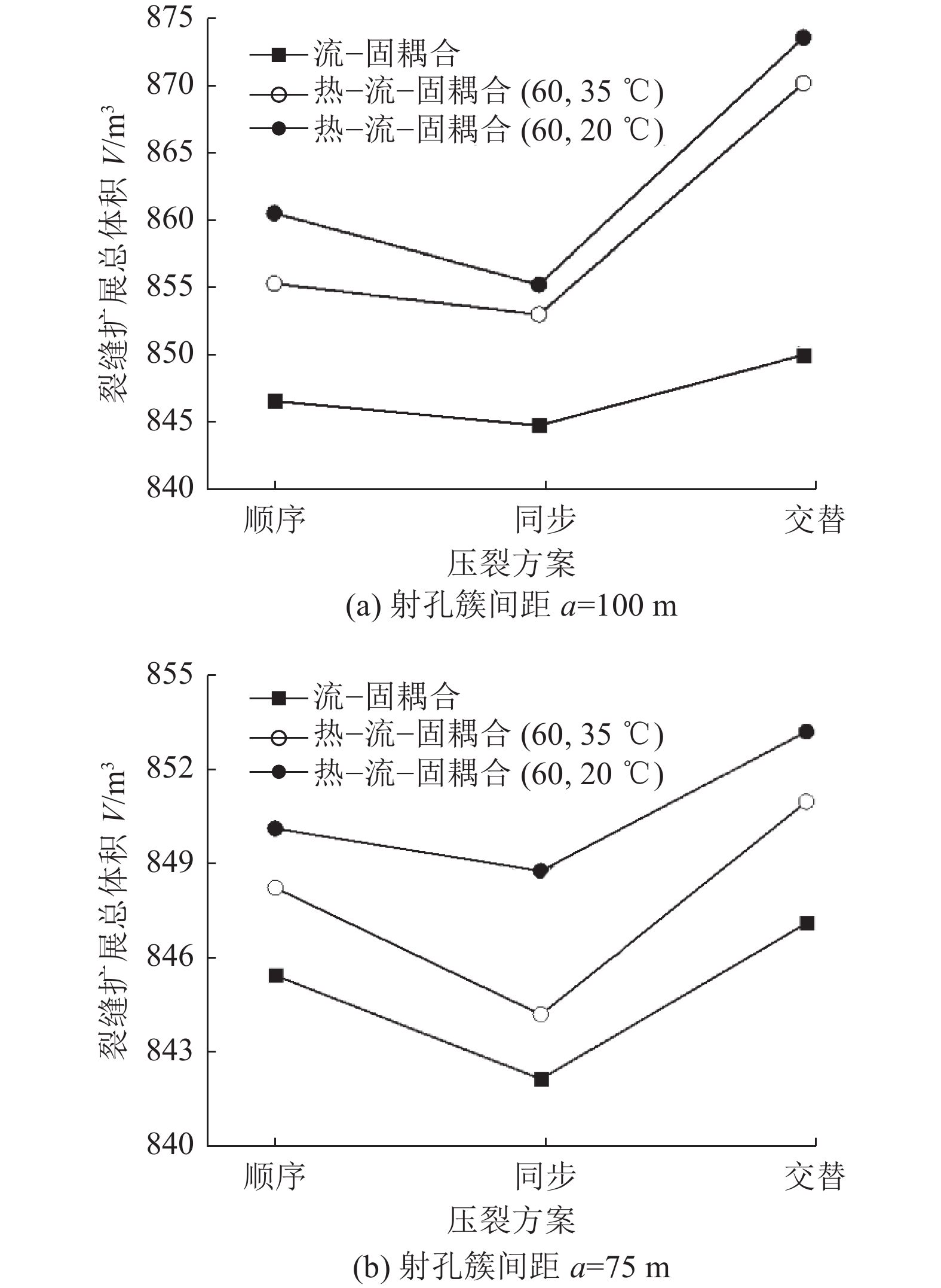
压裂方案 射孔簇间距 a/m 顺序 100 75 50 25 同步 100 75 — — 交替 100 75 — —
下载: 导出CSV
表 4 各压裂方案温度梯度设置
Table 4 Temperature gradients for fracturing scenarios
类别 温度/℃ 压裂液 20 35 岩石基质 60 60
下载: 导出CSV
表 5 各压裂方案下裂缝面积和体积结果
Table 5 Results of fracture areas and volumes in sequential fracturing
压裂方案 类别 a=100 m a=75 m 顺序 裂缝面积 S/m2 100.66 99.346 裂缝体积 V/m3 846.58 845.46 同步 裂缝面积 S/m2 100.29 98.523 裂缝体积 V/m3 844.76 842.15 交替 裂缝面积 S/m2 103.23 99.467 裂缝体积 V/m3 849.99 847.13
下载: 导出CSV
-
[1] HE Q,SUORINENI F T,MA T,et al. Effect of discontinuity stress shadows on hydraulic fracture re-orientation[J]. International Journal of Rock Mechanics and Mining Sciences,2017,91:179−194. doi: 10.1016/j.ijrmms.2016.11.021
[2] GUTIERREZ R,SANCHEZ E,ROEHL D,et al. XFEM modeling of stress shadowing in multiple hydraulic fractures in multi-layered formations[J]. Journal of Natural Gas Science and Engineering,2019,70:1−15.
[3] SOBHANIARAGH B,MANSUR W,PETERS F. The role of stress interference in hydraulic fracturing of horizontal wells[J]. International Journal of Rock Mechanics and Mining Sciences,2018,106:153−164. doi: 10.1016/j.ijrmms.2018.04.024
[4] YOON J S,ZIMMERMANN G,ZANG A. Numerical investigation on stress shadowing in fluid injection-induced fracture propagation in naturally fractured geothermal reservoirs[J]. Rock Mechanics and Rock Engineering,2015,48(4):1439−1454. doi: 10.1007/s00603-014-0695-5
[5] WONG S W, GEILIKMAN M, XU G. Interaction of multiple hydraulic fractures in horizontal wells[C]. SPE Middle East Unconventional Resources Conference and Exhibition, 2013.
[6] MANRIQUEZ A L,SEPEHRNOORI K,CORTES A. A novel approach to quantify reservoir pressure along the horizontal section and to optimize multistage treatments and spacing between hydraulic fractures[J]. Journal of Petroleum Science and Engineering,2017,149:579−590. doi: 10.1016/j.petrol.2016.10.068
[7] BAŽANT Z P,SALVIATO M,CHAU V T,et al. Why fracking works[J]. Journal of Applied Mechanics,2014,81(10):1−10.
[8] ZHANG X,JEFFREY R. Fluid-driven multiple fracture growth from a permeable bedding plane intersected by an ascending hydraulic fracture[J]. Journal of Geophysical Research:Solid Earth,2012,117:1−12.
[9] 康向涛,江明泉,黄 滚,等. 多煤层联合水压裂缝扩展规律应用研究[J]. 采矿与安全工程学报,2021,38(3):602−608. doi: 10.13545/j.cnki.jmse.2020.0586 KANG Xiangtao,JIANG Mingquan,HUANG Gun,et al. Application research on fracture propagation law of combined hydraulic pressure in multiple coal seams[J]. Journal of Mining & Safety Engineering,2021,38(3):602−608. doi: 10.13545/j.cnki.jmse.2020.0586
[10] LECAMPION B,DESROCHES J. Simultaneous initiation and growth of multiple radial hydraulic fractures from a horizontal wellbore[J]. Journal of the Mechanics and Physics of Solids,2015,82:235−258. doi: 10.1016/j.jmps.2015.05.010
[11] MANRÍQUEZ A L. Stress behavior in the near fracture region between adjacent horizontal wells during multistage fracturing using a coupled stress-displacement to hydraulic diffusivity model[J]. Journal of Petroleum Science and Engineering,2018,162:822−834. doi: 10.1016/j.petrol.2017.11.009
[12] GHADERI A,TAHERI-SHAKIB J,NIK M. The distinct element method (DEM) and the extended finite element method (XFEM) application for analysis of interaction between hydraulic and natural fractures[J]. Journal of Petroleum Science and Engineering,2018,171:422−430. doi: 10.1016/j.petrol.2018.06.083
[13] HOSSAIN M M,RAHMAN M K. Numerical simulation of complex fracture growth during tight reservoir stimulation by hydraulic fracturing[J]. Journal of Petroleum Science and Engineering,2008,60(2):86−104. doi: 10.1016/j.petrol.2007.05.007
[14] PALUSZNY A,TANG X H,ZIMMERMAN R W. Fracture and impulse based finite-discrete element modeling of fragmentation[J]. Computational Mechanics,2013,52(5):1071−1084. doi: 10.1007/s00466-013-0864-5
[15] TAGHICHIAN A,ZAMAN M,DEVEGOWDA D. Stress shadow size and aperture of hydraulic fractures in unconventional shales[J]. Journal of Petroleum Science and Engineering,2014,124:209−221. doi: 10.1016/j.petrol.2014.09.034
[16] KRESSE O,WENG X,GU H,et al. Numerical modeling of hydraulic fractures interaction in complex naturally fractured formations[J]. Rock Mechanics and Rock Engineering,2013,46(3):555−568. doi: 10.1007/s00603-012-0359-2
[17] KUMAR D,GHASSEMI A. A three-dimensional analysis of simultaneous and sequential fracturing of horizontal wells[J]. Journal of Petroleum Science and Engineering,2016,146:1006−1025. doi: 10.1016/j.petrol.2016.07.001
[18] WANG Y L. An h-version adaptive FEM for eigenproblems in system of second order ODEs: vector Sturm-Liouville problems and free vibration of curved beams[J]. Engineering Computations,2020,37(1):1210−1225.
[19] 袁 驷,王永亮,徐俊杰. 二维自由振动的有限元线法自适应分析新进展[J]. 工程力学,2014,31(1):15−22. doi: 10.6052/j.issn.1000-4750.2013.05.ST01 YUAN Si,WANG Yongliang,XU Junjie. New progress in self-adaptive FEMOL analysis of 2D free vibration problems[J]. Engineering Mechanics,2014,31(1):15−22. doi: 10.6052/j.issn.1000-4750.2013.05.ST01
[20] 王永亮. 含裂纹损伤圆弧曲梁弹性屈曲的有限元网格自适应分析[J]. 工程力学,2020,35(1):55−62. WANG Yongliang. Adaptive mesh refinement analysis of finite element method for elastic buckling of circularly cracked curved beams[J]. Engineering Mechanics,2020,35(1):55−62.
[21] WANG Y L,JU Y,ZHUANG Z,et al. Adaptive finite element analysis for damage detection of non–uniform Euler–Bernoulli beams with multiple cracks based on natural frequencies[J]. Engineering Computations,2017,35(3):1203−1229.
[22] AZADI H,KHOEI A R. Numerical simulation of multiple crack growth in brittle materials with adaptive remeshing[J]. International Journal for Numerical Methods in Engineering,2011,85(8):1017−1048. doi: 10.1002/nme.3002
[23] WANG Y L,JU Y,CHEN J L,et al. Adaptive finite element–discrete element analysis for the multistage supercritical CO2 fracturing of horizontal wells in tight reservoirs considering pre-existing fractures and thermal-hydro-mechanical coupling[J]. Journal of Natural Gas Science and Engineering,2019,61:251−269. doi: 10.1016/j.jngse.2018.11.022
[24] 王永亮,鞠 杨,陈佳亮,等. 自适应有限元−离散元算法、ELFEN软件及页岩体积压裂应用[J]. 工程力学,2018,35(9):17−25,36. doi: 10.6052/j.issn.1000-4750.2017.06.0421 WANG Yongliang,JU Yang,CHEN Jialiang,et al. Adaptive finite element-discrete element algorithm, software ELFEN and application in stimulated reservoir volume of shale[J]. Engineering Mechanics,2018,35(9):17−25,36. doi: 10.6052/j.issn.1000-4750.2017.06.0421
[25] PROFIT M,DUTKO M,YU J,et al. Complementary hydro-mechanical coupled finite/discrete element and microseismic modelling to predict hydraulic fracture propagation in tight shale reservoirs[J]. Computational Particle Mechanics,2016,3(2):229−248. doi: 10.1007/s40571-015-0081-4
[26] ZIENKIEWICZ O C,ZHU J Z. The superconvergent patch recovery (SPR) and adaptive finite element refinement[J]. Computer Methods in Applied Mechanics and Engineering,1992,101(1):207−224.
[27] WANG Y L, JU Y, ZHANG H M, et al. Adaptive finite element–discrete element analysis for the stress shadow effects and fracture interaction behaviours in three-dimensional multistage hydrofracturing considering varying perforation cluster spaces and fracturing scenarios of horizontal wells[J]. Rock Mechanics and Rock Engineering, 2020, 120(3): 1226–1252.
计量
- 文章访问数: 243
- HTML全文浏览量: 42
- PDF下载量: 79
