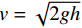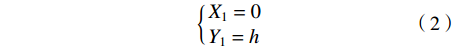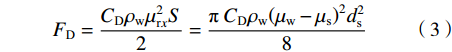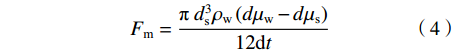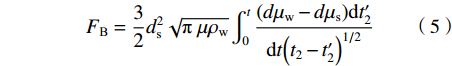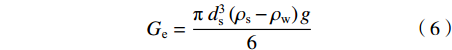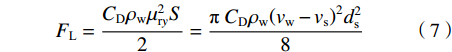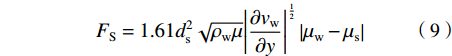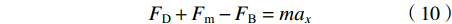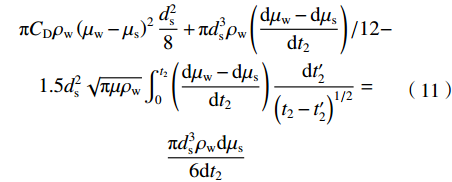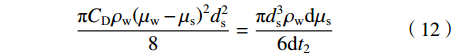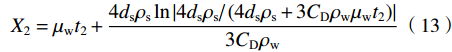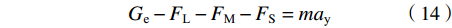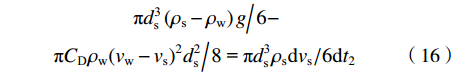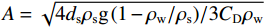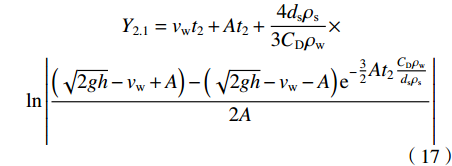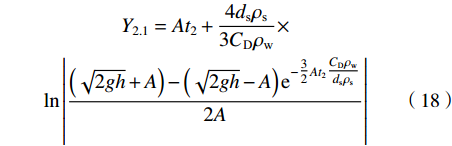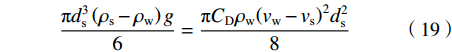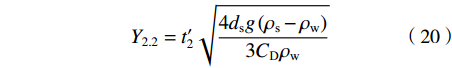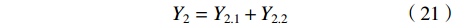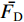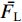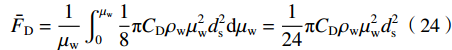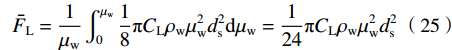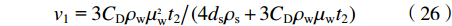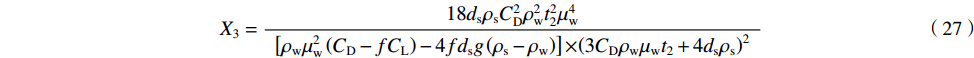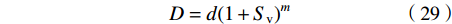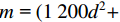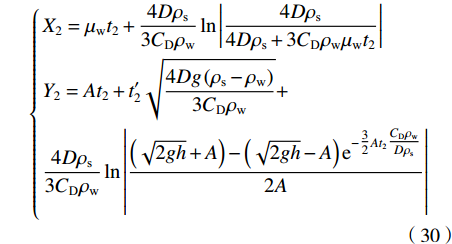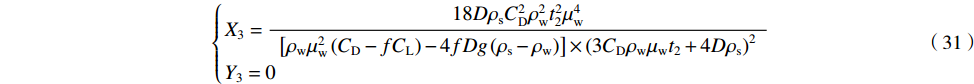Study on the migration law of aggregate sediment in the water inrush channel of coal rock mass
-
摘要:
先行灌注骨料后注浆可有效控制煤岩体突水。骨料在煤岩体突水通道中沉积运移规律的研究是确定骨料粒径、骨料灌注量以及骨料灌注孔间距的重要前提，但目前在该方面的理论和试验研究甚少，实际施工主要依赖经验，带有较大的盲目性。鉴于此，基于浆体管道输送理论和泥沙运动力学，结合牛顿第二定律，将骨料从灌注孔进入突水通道的全运移过程划分为：自由落体，类平抛以及滑移3个运动阶段，并分别对其进行受力分析，建立由单颗骨料到骨料颗粒群的沉积运移扩散模型，得出表征骨料颗粒群在水平方向上的沉积运移规律；自行设计煤岩体突水通道骨料沉积运移可视化的模型试验平台，借助该平台开展在不同动水流速、骨料粒径、灌注高度等多因素影响下的骨料沉积运移模型试验，分析骨料在突水通道内的沉积运移规律，得到在不同试验方案下骨料沉积运移区的总长度。试验结果表明：骨料实际运移过程与理论3个运动阶段的假设吻合，骨料沉积运移距离的试验值与理论计算值平均误差为7.34%；在控制投注高度与动水条件一致的前提下，相较细骨料（粒径≤5 mm）而言，理论公式对于粗骨料（粒径>5 mm）更具适用性；在工程中解决煤岩体突水问题时，若骨料粒径>5 mm，利用本文理论公式可计算得到骨料颗粒群在水平方向上的运移距离，从而确定骨料灌注孔的间距，降低实际施工过程中的盲目性。
Abstract:Grouting after pouring aggregate can effectively control water inrush from coal and rock mass. The study on the deposition and migration law of aggregate in the water inrush channel from coal and rock mass is an important prerequisite to determine the aggregate particle size, aggregate grouting volume and the spacing of aggregate grouting holes. However, there are few theoretical and experimental studies in this area at present, and the actual construction mainly depends on experience, which is blind. In view of this, based on the slurry pipeline transportation theory and sediment movement mechanics, combined with Newton’s second law, the whole movement process of aggregate from the pouring hole to the water inrush channel is divided into three movement stages: free fall, similar horizontal throwing and sliding, and their stress analysis is carried out respectively. A sedimentation, migration and diffusion model from single aggregate to aggregate particle group is established, and the sedimentation and migration rules characterizing the aggregate particle group in the horizontal direction are obtained; A model test platform for visualization of aggregate deposition and movement in water inrush channel of coal and rock mass is designed by ourselves. With the help of this platform, aggregate deposition and movement model tests are carried out under the influence of various factors such as different hydrodynamic velocity, aggregate particle size, pouring height, et al, to analyze the deposition and movement rules of aggregates in water inrush channel, and to obtain the total length of aggregate deposition and movement area under different test schemes. The experimental results show that the actual migration process of aggregate is consistent with the assumption of three movement stages in theory, and the average error between the experimental value and the theoretical calculation value of the sediment migration distance of aggregate is 7.34%; On the premise that the injection height is controlled to be consistent with the dynamic water conditions, the theoretical formula is more applicable to coarse aggregate (particle size > 5 mm) than to fine aggregate (particle size ≤ 5 mm); When solving the problem of water inrush from coal and rock mass in engineering, if the aggregate particle size is greater than 5 mm, the migration distance of aggregate particle group in the horizontal direction can be calculated by using the theoretical formula in this paper, so as to determine the spacing of aggregate grouting holes and reduce the blindness in the actual construction process.
-
0. 引 言
煤炭是我国主体能源，安全开采是生产工作的重中之重[1-2]。在我国，随着煤炭开采量的增加，各类型安全事故频发，突涌水害尤甚。其致害特点有：突水水源多样，突水通道不确定，突水位置随机，突水方式存在差异[3-4]。结合其致害特点，基于浆体管道输送理论与泥沙运动力学的动水截流技术：通过先灌注骨料后注浆的方式建立“阻水段”，截断突水水源，是目前堵水工程中最为有效的快速截流控制方法[5-6]。其中浆体管道输送理论的基础是水力输送固体颗粒的力学机制，此对于突水通道中骨料沉积运移规律的研究具有指导意义[7-9]：瓦莱斯.C.安德鲁斯（Walath.C.Andlus）[10]首次提出管道运输固体物料的技术；杜兰德（Durand）[11]、瓦斯普（Wasp）[12]、格拉夫（Graf）[13]以及费祥俊[14]等，完成了管道中固体颗粒由悬浮至沉底，临界速度经验计算公式的建立；随着科学技术条件的成熟，相关研究结合室内试验以及数值模拟更具针对性。MATOUSEK[15]通过室内模拟试验，对固体颗粒在不同流速状态下动力损失的情况进行了研究；许振良等[16]通过数值模拟，完成了对于固—液间动量交换前后速度变化关系的研究；李维欣[17]、惠爽[18]设计骨料灌注可视化模型试验，分析影响骨料截流的因素。突水通道中骨料颗粒的运移还可以运用泥沙运动力学进行分析。泥沙运动力学的研究始于19世纪末，DUBOYS[19]首次提出了泥沙颗粒在水流中运动时受水流拖曳力的概念。ROUSE[20]、BAGNOLD[21]以及ENGELUND[22]等通多室内水槽试验研究泥沙运动的规律，建立了泥沙携沙力、推移质输沙率以及悬移质输沙率等的计算公式，使学科初具体系。张瑞瑾[23]在“制紊假说”的指导下，结合我国长江、黄河以及部分水渠实际工程资料，建立了广泛使用的悬移质携沙力公式；窦国仁[24]利用平均流速推导出均匀泥沙推移质的输沙率公式，结合牛顿第二定律推导出新的携沙力公式；韩其为与何民明[25]总结出非均匀泥沙颗粒运动的规律。
综上所述，众多学者针对不同边界条件下的固液两相流问题进行了大量研究，但是由于受到突涌水致害特点的影响，对骨料在煤岩体突水通道中运动规律的研究仍存在不足。笔者基于浆体管道输送理论以及泥沙运动力学，建立了表征骨料颗粒在突水通道中沉积运移规律的理论模型，通过开展室内突水通道中骨料沉积运移模型试验验证其适用性，为优化骨料灌注工艺，预估骨料投注孔的位置提供理论依据。
1. 突水通道中骨料沉积运移模型
1.1 单颗骨料颗粒沉积运移规律
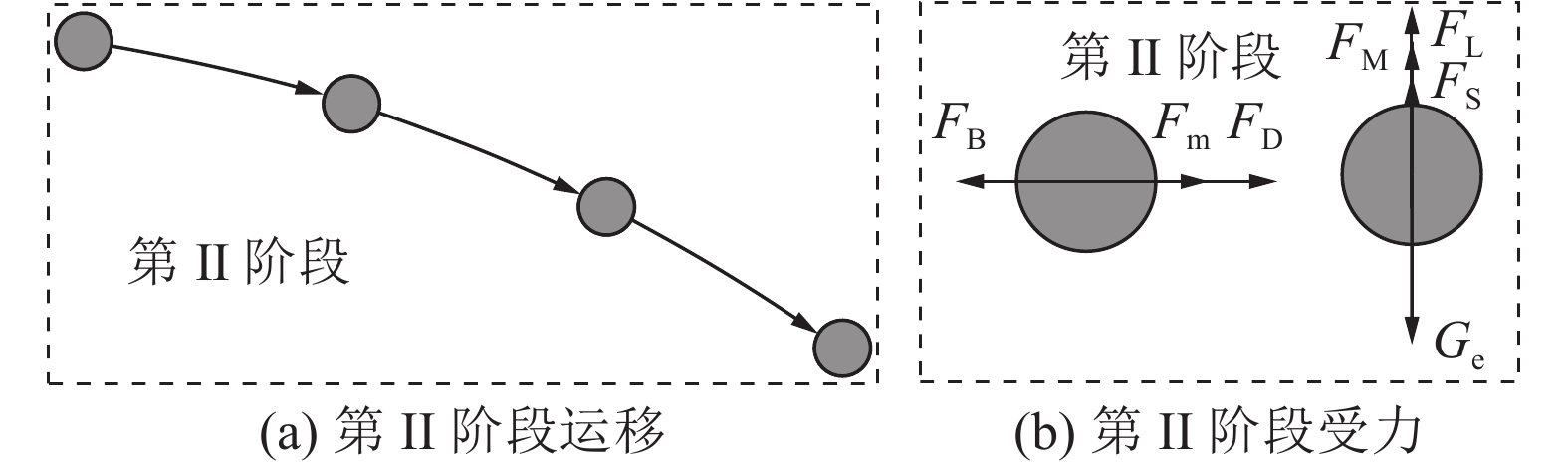
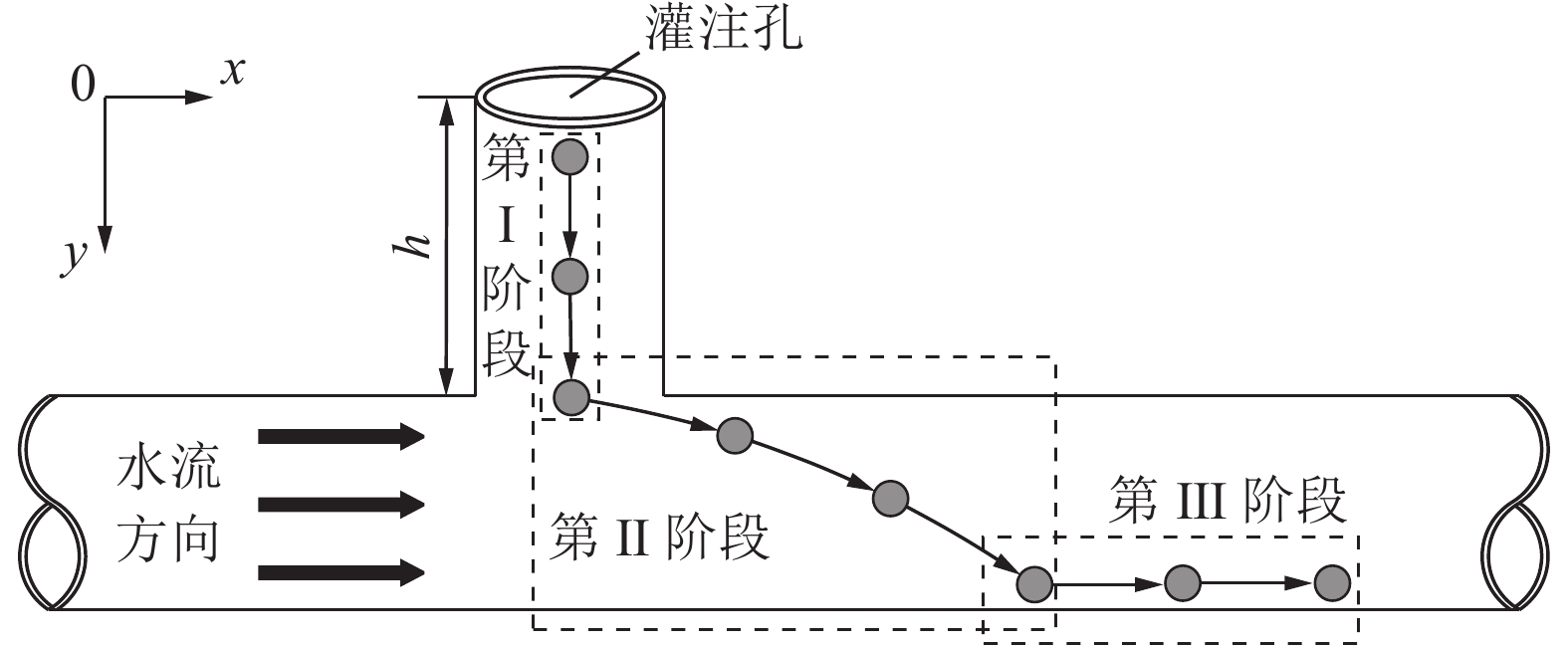
根据浆体管道输送理论、泥沙运动力学以及笔者对于初步试验结果的观察，将骨料在突水通道中的全运动过程分为3个阶段：第Ⅰ阶段，骨料进入灌注孔做自由落体运动直至与水流相接触；第Ⅱ阶段，骨料与水流相接触后，在动水条件作用下做类平抛运动，从涌水面下沉至突水通道底部；第Ⅲ阶段：骨料在突水通道底部，在水流推力和摩擦力的作用下，滑移一段距离后停止，做滑移运动。全运动过程如图1所示。
考虑到骨料颗粒在突水条件作用下受力的复杂性与随机性，为简化计算，做出如下假设：①所灌注的骨料颗粒为质量相等的均匀球体；②突水通道内水流向通道外的渗流作用可忽略不计；③突水通道内，水流各向同性且不可压缩，流态为湍流；④骨料颗粒进入灌注孔在与孔内水流接触前做自由落体运动，空气阻力可忽略不计。
第Ⅰ阶段：此阶段骨料在灌注孔内做自由落体运动，该阶段骨料颗粒的运移以及受力情况如图2所示。
球形骨料颗粒的重力G：
$$ G = {\rho _{\text{s}}}{V_{\text{s}}}g=\frac{{{{\text{π}}d_{\text{s}}^{\text{3}}{\rho _{\text{s}}}g}}}{{6}} $$ (1) 式中：ds为球形骨料颗粒的直径，m；Vs为颗粒体积，m3；ρs为颗粒的密度，kg/m3；g为重力加速度，9.8 m/s2。
第Ⅰ阶段的下落时间：
$ {t_1} = \sqrt {{{2h} \mathord{\left/ {\vphantom {{2h} {\text{g}}}} \right. } {\text{g}}}} $ ；骨料颗粒结束自由落体运动接触到水面时的瞬时速度：$ v = \sqrt {2gh} $ 。第Ⅰ阶段水平以及竖直方向上的运移距离分别为：$$ \left\{ \begin{gathered} {X_1} = 0 \\ {Y_1} = h \\ \end{gathered} \right. $$ (2) 第Ⅱ阶段：此阶段骨料与水流相接触，做类平抛运动，属固液两相管道流问题[26]。建立B.B.O方程[27]：单个颗粒的受力矢量和等于其质量乘以加速度。骨料颗粒的运移以及受力分解如图3所示。
其中，水流拖曳力：
$$ {F_{\text{D}}} = \frac{{{{C_{\text{D}}}{\rho _{\text{w}}}\mu _{{\text{r}}x}^2S}}}{{ 2}} = \frac{{{{\text{π }}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right)}^2}d_{\text{s}}^2}}}{{8}} $$ (3) 式中：μw为水流水平流动速度，m/s；S为距离，m；μs为骨料颗粒水平运动速度，m/s；CD为水流拖曳力系数，0.4；ρw为流体密度，kg/m3。
附加质量力Fm：
$$ {F_{\text{m}}} = \frac{{{\text{π }}d_{\text{s}}^3{\rho _{\text{w}}}\left( {d{\mu _{\text{w}}} - d{\mu _{\text{s}}}} \right)}} {12{\rm{d}}t} $$ (4) Basset力FB：
$$ {F_{\text{B}}} = \frac{3}{2}d_{\text{s}}^2\sqrt {{\text{π }}\mu {\rho _{\text{w}}}} \int_0^{{t}} \frac{{\left( {d{\mu _{\text{w}}} - d{\mu _{\text{s}}}} \right)}{\rm{d}}t_2'}{{{\rm{d}}t{{\left( {{t_2} - t_2'} \right)}^{{1 / 2}}}}}$$ (5) 有效重力Ge：
$$ {G_{\text{e}}} = \frac{{{{\text{π }}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g}}}{{6}} $$ (6) 上举力FL：
$$ {F_{\text{L}}} = \frac{{{{C_{\text{D}}}{\rho _{\text{w}}}\mu _{{\text{r}}y}^2S}}}{{ 2}} = \frac{{{{\text{π }}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} }}{{8}} $$ (7) 式中：t为时间，s；vw为水流竖直流动速度，m/s；vs为骨料颗粒竖直运动速度，m/s。
Magnus力FM：
$$ {F_{\text{M}}} =\frac{{{{\text{π }}d_{\text{s}}^3{\rho _{\text{w}}}\omega \left( {{\mu _{\rm{w}}} - {\mu _{\rm{s}}}} \right)}}}{{ 8}} $$ (8) Saffman剪切升力FS：
$$ {F_{\rm{S}}} = 1.61d_{\text{s}}^2\sqrt {{\rho _{\text{w}}}\mu } {\left| \frac{{{\partial {v_{\text{w}}}} }}{{{\partial y}}} \right|^{\frac{1}{2}}}\left| {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right| $$ (9) 式中：μ为水流的动力黏度，Pa·s；∂vw/∂y为水流在流过断面处的速度梯度。
建立水平方向上的力学平衡方程：
$$ {F_{\text{D}}} + {F_{\text{m}}} - {F_{\text{B}}} = m{a_x} $$ (10) 代入式(3)—(5)中各水平方向作用力得：
$$ \begin{gathered} \pi C_{\mathrm{D}} \rho_{\mathrm{w}}\left(\mu_{\mathrm{w}}-\mu_{\mathrm{s}}\right)^2 \frac{{d_{\mathrm{s}}^2}}{{8}} +\pi d_{\mathrm{s}}^3 \rho_{\mathrm{w}}\left(\frac{{\rm{d}} \mu_{\mathrm{w}}-{\rm{d}} \mu_{\mathrm{s}} }{{\rm{d}} t_2}\right) / 12- \\ 1.5 d_{\mathrm{s}}^2 \sqrt{\pi \mu \rho_{\mathrm{w}}} \int_0^{t_2}\left(\frac{{\rm{d}} \mu_{\mathrm{w}}-{\rm{d}} \mu_{\mathrm{s}}} { {\rm{d}} t_2}\right) \frac{{\rm{d}} t_2^{\prime} }{\left(t_2-t_2^{\prime}\right)^{1 / 2}}= \\ \frac{{\pi {{d}}_{\mathrm{s}}^3 \rho_{\mathrm{w}} {\rm{d}} \mu_{\mathrm{s}} }}{{6 {\rm{d}} t_2}} \end{gathered}$$ (11) 忽略骨料颗粒在水平方向上较小的作用力可得：
$$\frac{{{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right)}^2}d_{\text{s}}^2}}}{{8}} = \frac{{{{\text{π}}d_{\text{s}}^3{\rho _{\text{w}}}{\rm{d}}{\mu _{\text{s}}}}}}{{ 6{\rm{d}}{t_2}}} $$ (12) 将μs=dX2/dt2，边界条件μs|t2=0以及X2|t2=0代入式(12)中，积分并化解，得出骨料颗粒在第Ⅱ阶段的水平运移距离表达式：
$$ {X_2} = {\mu _{\text{w}}}{t_2} + \frac{{4{d_{\text{s}}}{\rho _{\text{s}}}\ln \left| {{{4{d_{\text{s}}}{\rho _{\text{s}}}} \mathord{\left/ {\vphantom {{4{d_{\text{s}}}{\rho _{\text{s}}}} {\left( {4{d_{\text{s}}}{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}} \right)}}} \right. } {\left( {4{d_{\text{s}}}{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}} \right)}}} \right|}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}} $$ (13) 建立竖直方向上的力学平衡方程：
$$ {G_{\text{e}}} - {F_{\text{L}}} - {F_{\text{M}}} - {F_{\rm{S}}} = m{a_{\text{y}}} $$ (14) 代入式(6)—式(9)中各竖直方向作用力可得：
$$ \begin{split}& \qquad \quad {{{\text{π}}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g} \mathord{\left/ {\vphantom {{{\text{π}}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g} 6}} \right. } 6} - {{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} \mathord{\left/ {\vphantom {{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} 8}} \right. } 8} -\\& {{{\text{π}}d_{\text{s}}^3{\rho _{\text{w}}}\omega \left( {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right)} \mathord{\left/ {\vphantom {{{\text{π}}d_{\text{s}}^3{\rho _{\text{w}}}\omega \left( {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right)} 8}} \right. } 8} - 1.61d_{\text{s}}^2\sqrt {{\rho _{\text{w}}}\mu } {\left| {{{\partial {v_{\text{w}}}} \mathord{\left/ {\vphantom {{\partial {v_{\text{w}}}} {\partial y}}} \right. } {\partial y}}} \right|^{{1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}}}\left| {{\mu _{\text{w}}} - {\mu _{\text{s}}}} \right| =\\ &\qquad \qquad \qquad \qquad {\text{ }}{{{\text{π}}d_{\text{s}}^{\text{3}}{\rho _{\text{s}}}{\rm{d}}{v_{\text{s}}}} /{6{\rm{d}}{t_2}}} \\[-12pt] \end{split} $$ (15) 忽略骨料颗粒竖直方向上较小的作用力可得：
$$ \begin{split}&\qquad \qquad {{{\text{π}}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g} \mathord{\left/ {\vphantom {{{\text{π }}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g} 6}} \right. } 6}- \\& {{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} \mathord{\left/ {\vphantom {{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} 8}} \right. } 8}{\text{ = }}{{{\text{π}}d_{\text{s}}^{\text{3}}{\rho _{\text{s}}}{\rm{d}}{v_{\text{s}}}} / 6}{\rm{d}}{t_2} \end{split}$$ (16) 令
$ A = \sqrt {{{4{d_{\text{s}}}{\rho _{\text{s}}}{\text{g}}\left( {1 - {{{\rho _{\text{w}}}} /{{\rho _{\text{s}}}}}} \right)} / {3{C_{\text{D}}}{\rho _{\text{w}}}}}} $ ，vs=dY2.1/dt2。将边界条件vs|t2=以及Y2.1|t2=0代入式(16)整理并积分可得：$$ \begin{gathered} {Y_{2.1}} = {v_{\text{w}}}{t_2} + A{t_2} + \frac{{4{d_{\text{s}}}{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}\times \\ \ln \left| {\frac{{\left( {\sqrt {2gh} - {v_{\text{w}}} + A} \right) - \left( {\sqrt {2gh} - {v_{\text{w}}} - A} \right){{\rm{e}}^{ - \textstyle\frac{3}{2}A{t_2}\textstyle\frac{{{C_{\text{D}}}{\rho _{\text{w}}}}}{{{d_{\text{s}}}{\rho _{\text{s}}}}}}}}}{{2A}}} \right| \\ \end{gathered} $$ (17) 考虑到竖直方向水流速度较小，可忽略不记，则：
$$ \begin{split}&\qquad \qquad {Y_{2.1}} = A{t_2} + \frac{{4{d_{\text{s}}}{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}} \times\\& \ln \left| {\frac{{\left( {\sqrt {2gh} + A} \right) - \left( {\sqrt {2gh} - A} \right){{\rm{e}}^{ - \tfrac{3}{2}A{t_2}\tfrac{{{C_{\text{D}}}{\rho _{\text{w}}}}}{{{d_{\text{s}}}{\rho _{\text{s}}}}}}}}}{{2A}}} \right| \end{split} $$ (18) 上式得出了单颗骨料颗粒在竖直方向上的运移距离Y与第Ⅱ阶段运动时间t2的表达式。若已知突水通道的边界宽度，即可代入式(18)中，反算出第Ⅱ阶段的运动时间t2，再将其代入式(13)中，计算得到单颗骨料在水平方向上的运移距离。但在实际情况中，骨料在第Ⅱ阶段的运动中伴有较多不确定的随机性，往往无法一次性直接降落并静止停留在突水通道底部，所以需要对t2进行修正，得出更为准确的第Ⅱ阶段竖直方向上的运移距离Y2。
骨料进入突水通道与水流相接触，经过一段时间的非匀速运动后，在竖直方向上，其有效重力等于上举力，加速度为0，匀速下沉至突水通道底部，即：
$$ \frac{{{{\text{π}}d_{\text{s}}^{\text{3}}\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)g}}}{{6}} =\frac{{{{\text{π}}{C_{\text{D}}}{\rho _{\text{w}}}{{\left( {{v_{\text{w}}} - {v_{\text{s}}}} \right)}^2}d_{\text{s}}^2} }}{{ 8}} $$ (19) 将vw=0，vs=dY2.2/dt'2代入式(19)整理化解可得：
$$ {Y_{2.2}} = t_2'\sqrt {{\frac{{{4{d_{\text{s}}}g\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)}}}{{{3{C_{\text{D}}}{\rho _{\text{w}}}}}} }} $$ (20) 故竖直方向上的总位移为：
$$ {Y_2} = {Y_{2.1}} + {Y_{2.2}} $$ (21) 第Ⅱ阶段水平以及竖直方向上的运移距离分别为：
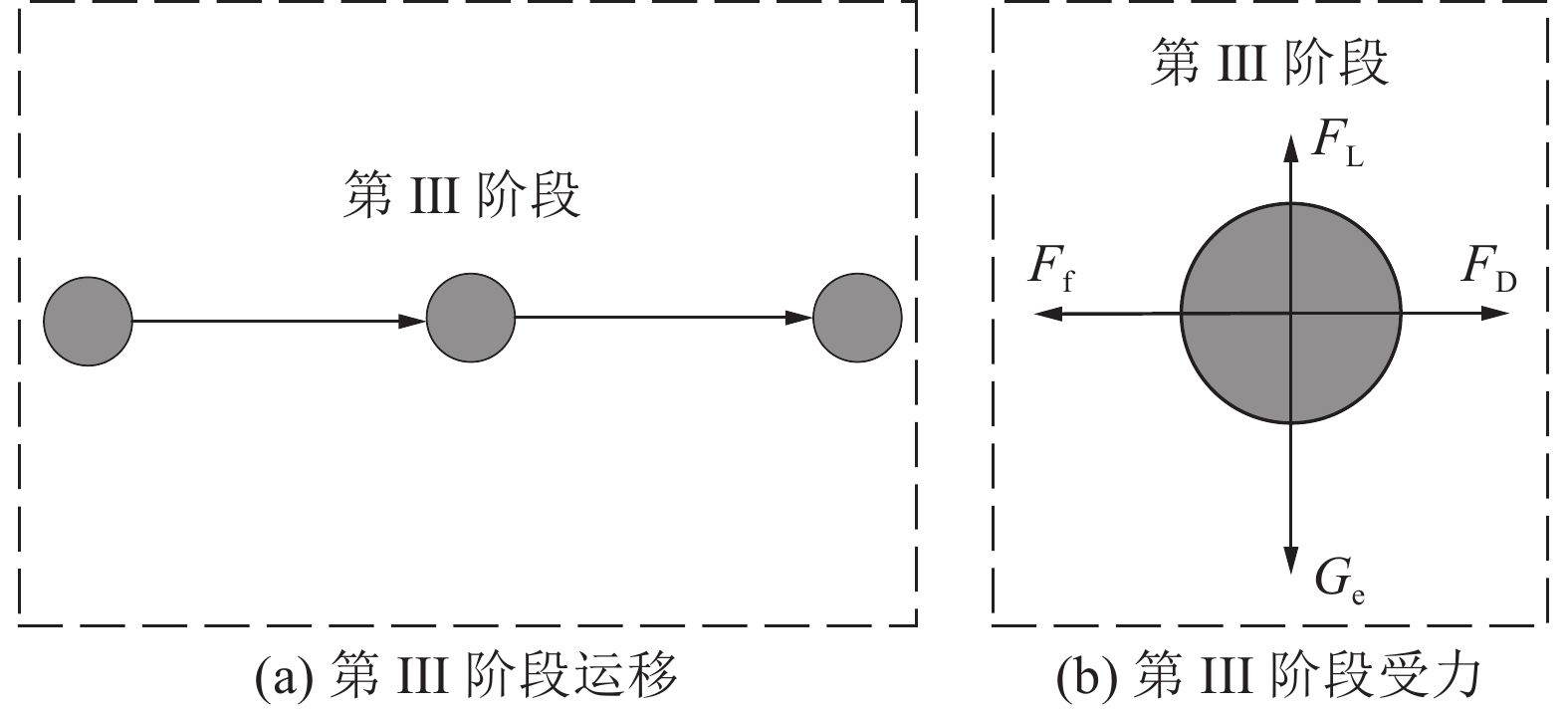
$$ \left\{ \begin{gathered} {X_2} = {\mu _{\text{w}}}{t_2} + \frac{{4{d_{\text{s}}}{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}\ln \left| {\frac{{4{d_{\text{s}}}{\rho _{\text{s}}}}}{{4{d_{\text{s}}}{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}}}} \right| \\ {Y_2} = A{t_2} + t_2'\sqrt {\frac{{4{d_{\text{s}}}g\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}}\times \\ \frac{{4{d_{\text{s}}}{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}\ln \left| {\frac{{\left( {\sqrt {2gh} + A} \right) - \left( {\sqrt {2gh} - A} \right){{\rm{e}}^{ - \tfrac{3}{2}A{t_2}\tfrac{{{C_{\text{D}}}{\rho _{\text{w}}}}}{{{d_{\text{s}}}{\rho _{\text{s}}}}}}}}}{{2A}}} \right| \\ \end{gathered} \right. $$ (22) 第Ⅲ阶段：骨料颗粒沉底后，进入到第Ⅲ阶段的运动，在摩擦阻力的作用下速度逐渐减小，直至静止，运动轨迹为直线。其运移以及受力情况如图4所示：
根据动能定理列出平衡方程式：
$$ \left[ { \bar {{F_{\text{D}}}} - f\left( {{G_{\text{e}}} - \bar {{F_{\text{L}}}} } \right)} \right]{X_3} = \left| {\frac{1}{2}mv_2^2 - \frac{1}{2}mv_1^2} \right| $$ (23) 式中：
$\bar {{F_{\text{D}}}} $ 和$\bar {{F_{\text{L}}}} $ 分别为水流拖曳力FD和上举力FL的均值，其中：$$ \bar{F}_{\mathrm{D}}=\frac{1}{\mu_{\mathrm{w}}} \int_0^{\mu_{\mathrm{w}}} \frac{1}{8} \pi C_{\mathrm{D}} \rho_{\mathrm{w}} \mu_{\mathrm{w}}^2 d_{\mathrm{s}}^2 {\rm{d}} \mu_{\mathrm{w}}=\frac{1}{24} \pi C_{\mathrm{D}} \rho_{\mathrm{w}} \mu_{\mathrm{w}}^2 d_{\mathrm{s}}^2$$ (24) $$ \bar{F}_{\mathrm{L}}=\frac{1}{\mu_{\mathrm{w}}} \int_0^{\mu_{\mathrm{w}}} \frac{1}{8} \pi C_{\mathrm{L}} \rho_{\mathrm{w}} \mu_{\mathrm{w}}^2 d_{\mathrm{s}}^2 {\rm{d}} \mu_{\mathrm{w}}=\frac{1}{24} \pi C_{\mathrm{L}} \rho_{\mathrm{w}} \mu_{\mathrm{w}}^2 d_{\mathrm{s}}^2$$ (25) 骨料在第Ⅱ阶段的运动时间为t2，计算得出其在第Ⅲ阶段水平方向上的初始速度v1为：
$$ {v_1}{\text{ = }}{{3{C_{\text{D}}}{\rho _{\text{w}}}\mu _{\text{w}}^{_{\text{2}}}{t_2}} \mathord{\left/ {\vphantom {{3{C_{\text{D}}}{\rho _{\text{w}}}\mu _{\text{w}}^{_{\text{2}}}{t_2}} {\left( {4{d_{\text{s}}}{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}} \right)}}} \right. } {\left( {4{d_{\text{s}}}{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}} \right)}} $$ (26) 将式(24)—(26)代入式(23)，得出其在第Ⅲ阶段水平方向上的位移：
$${X_3} = \frac{{18{d_{\rm{s}}}{\rho _{\rm{s}}}C_{\rm{D}}^2\rho _{\rm{w}}^2t_2^2\mu _{\rm{w}}^4}}{{\begin{array}{*{20}{c}} {\left[ {{\rho _{\rm{w}}}\mu _{\rm{w}}^2\left( {{C_{\rm{D}}} - f{C_{\rm{L}}}} \right) - 4f{d_{\rm{s}}}g\left( {{\rho _{\rm{s}}} - {\rho _{\rm{w}}}} \right)} \right] \times } {{{\left( {3{C_{\rm{D}}}{\rho _{\rm{w}}}{\mu _{\rm{w}}}{t_2} + 4{d_{\rm{s}}}{\rho _{\rm{s}}}} \right)}^2}} \end{array}}}$$ (27) 第Ⅲ阶段水平以及竖直方向的运移距离分别为：
$$\left\{ \begin{array}{l} {X_3} = \dfrac{{18{d_{\rm{s}}}{\rho _{\rm{s}}}C_{\rm{D}}^2\rho _{\rm{w}}^2t_2^2\mu _{\rm{w}}^4}}{\begin{array}{l} \left[ {{\rho _{\rm{w}}}\mu _{\rm{w}}^2\left( {{C_{\rm{D}}} - f{C_{\rm{L}}}} \right) - 4f{d_{\rm{s}}}g\left( {{\rho _{\rm{s}}} - {\rho _{\rm{w}}}} \right)} \right] \times {\left( {3{C_{\rm{D}}}{\rho _{\rm{w}}}{\mu _{\rm{w}}}{t_2} + 4{d_{\rm{s}}}{\rho _{\rm{s}}}} \right)^2} \end{array}}\\ {Y_3} = 0 \end{array} \right.$$ (28) 1.2 骨料颗粒群的沉积运移规律研究
由单颗骨料在各阶段中的沉积运移公式可知：骨料粒径通过影响单颗粒的运动，控制颗粒群的运移与沉降。在前文基础上引入颗粒的体积浓度Sv修正粒径，骨料颗粒的粒径与骨料浓度的关系可用下式表示：
$$ D = d{\left( {1 + {S_{\text{v}}}} \right)^{{m}}} $$ (29) 式中：D为骨料颗粒群的算术平均粒径，cm；Sv为流体中骨料颗粒的体积浓度，当骨料颗粒群沉积物的浓度趋近于无穷小时，D为单颗骨料颗粒的粒径，cm；m为待定系数：
$m{\text{ = }}( 1\;200{d^2} + 12.15 )S_{\text{v}}^{3.31d - 0.74}$ 。将式(29)中的D值代入式(22)和(28)中，即可得出修正后骨料颗粒群在第Ⅱ、Ⅲ阶段中水平以及竖直方向上的运移距离的计算公式，分别为：
$$ \left\{ \begin{gathered} {X_2} = {\mu _{\text{w}}}{t_2} + \frac{{4D{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}\ln \left| {\frac{{4D{\rho _{\text{s}}}}}{{4D{\rho _{\text{s}}} + 3{C_{\text{D}}}{\rho _{\text{w}}}{\mu _{\text{w}}}{t_2}}}} \right| \\ {Y_2} = A{t_2} + t_2'\sqrt {\frac{{4Dg\left( {{\rho _{\text{s}}} - {\rho _{\text{w}}}} \right)}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}} + \\ \frac{{4D{\rho _{\text{s}}}}}{{3{C_{\text{D}}}{\rho _{\text{w}}}}}\ln \left| {\frac{{\left( {\sqrt {2gh} + A} \right) - \left( {\sqrt {2gh} - A} \right){{\rm{e}}^{ - \tfrac{3}{2}A{t_2}\tfrac{{{C_{\text{D}}}{\rho _{\text{w}}}}}{{D{\rho _{\text{s}}}}}}}}}{{2A}}} \right| \\ \\ \end{gathered} \right. $$ (30) $$ \left\{ \begin{gathered} {X_3} = \frac{{18D{\rho _{\text{s}}}C_{\text{D}}^2\rho _{\text{w}}^2t_2^2\mu _{\text{w}}^4}}{\begin{array}{l} \left[ {{\rho _{\rm{w}}}\mu _{\rm{w}}^2\left( {{C_{\rm{D}}} - f{C_{\rm{L}}}} \right) - 4fDg\left( {{\rho _{\rm{s}}} - {\rho _{\rm{w}}}} \right)} \right] \times {\left( {3{C_{\rm{D}}}{\rho _{\rm{w}}}{\mu _{\rm{w}}}{t_2} + 4D{\rho _{\rm{s}}}} \right)^2} \end{array} }\\ {Y_3} = 0 \\ \end{gathered} \right. $$ (31) 2. 突水通道中骨料颗粒运移模型试验
本节通过建立煤岩体突水通道骨料沉积运移可视化的模型试验平台，开展室内试验，验证前文所建立的理论计算模型，揭示出具有实际指导意义的骨料沉积运移规律。
2.1 突水通道骨料截流模拟试验平台建立
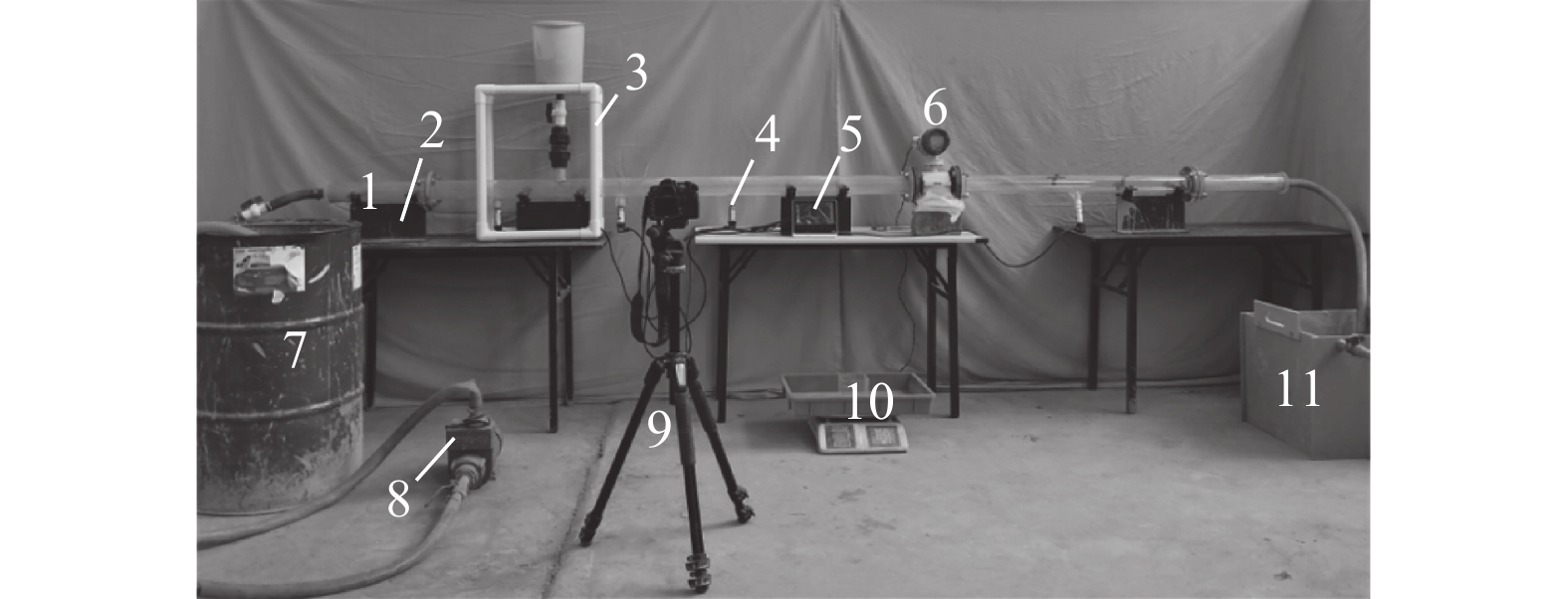
根据实际工程情况结合室内试验条件，设计突水通道骨料截流模拟试验平台如图5所示。
![]()
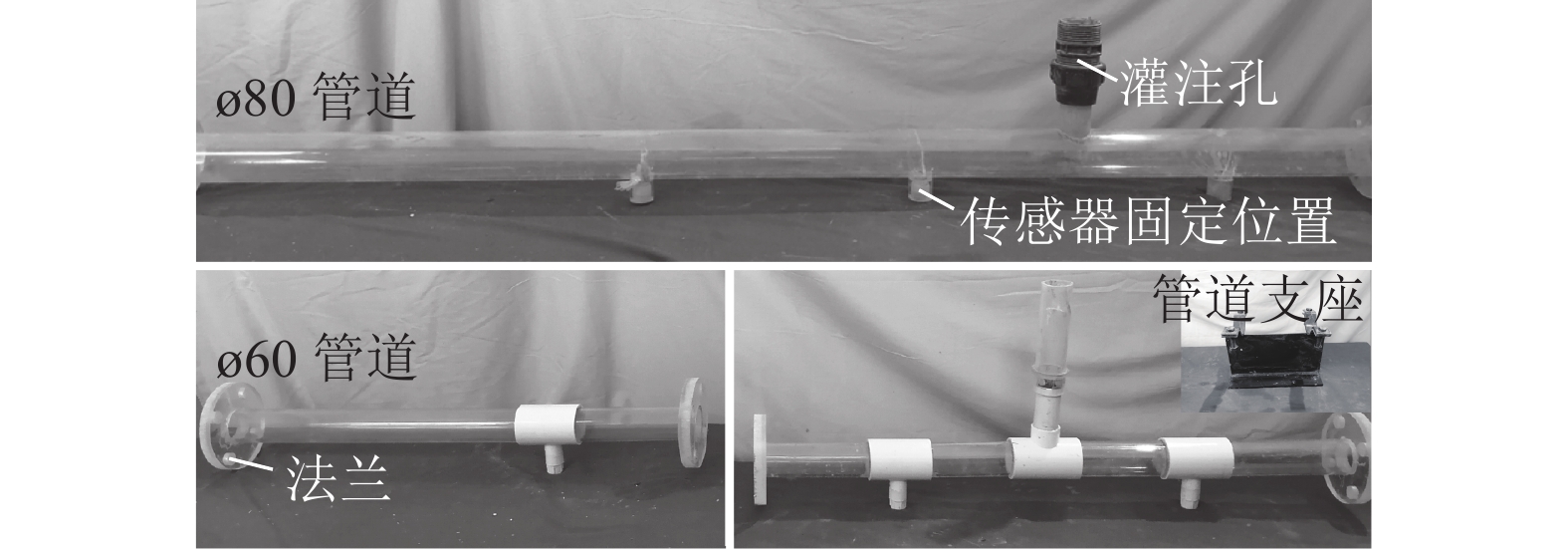
图 5 煤岩体突水通道骨料运移可视化的模型试验平台1—亚克力管道；2—管道支座；3—骨料灌注系统；4—压力传感器；5—无纸记录仪；6—电磁流量计；7—供水桶；8—恒压水泵；9—相机；10—称量装置；11—骨料回收系统Figure 5. Model test platform for visualization of aggregate migration in coal and rock water inrush channel煤岩体突水通道复杂多样，为便于观察和对比研究，采用亚克力透明管模拟突水通道，如图6所示。结合：几何相似性、力学相似性、运动相似性以及初始边界条件相似性等试验要求，将管壁内径确定为50 mm和70 mm，壁厚确定为10 mm，管道总长设置为5 m，具体参数见表1。两种管道均在距入水口1 m处设置高15 cm，内径5 cm的骨料灌注孔。进出水口处设置控水阀门，依据相似理论以及雷诺数的大小，将动水流速设定为0.15 m/s和0.30 m/s。为达到满流效果，关闭出水阀门后开始进水，待得管内水流充满后打开出水阀门，流量计所显示的动水流速稳定于要求流速后开展后续试验。
表 1 模拟突水通道参数表Table 1. Parameters of simulated water inrush channel规格 材质 内径/mm 壁厚/mm 连接方式 长度 ø60 PMMA 50 10 法兰
橡胶垫1 m×5 ø80 PMMA 70 10 法兰
橡胶垫3 m×1
1 m×1
0.5 m×2骨料灌注系统如图7所示，由储料桶、支架以及称量装置共同组成，其中支架由PVC管和若干管道三通拼接而成，骨料投注的初始高度设置为40 cm，后以此为基础，分别增加10、30和50 cm。
试验主要采用细（粒径≤5 mm）、粗（粒径>5 mm）2种骨料，结合土力学以及室内试验条件，筛选出细骨料（0～2、2～5 mm）和粗骨料（5～8、8～10 mm）4种粒径范围的骨料作为试验原料。
2.2 试验方案设计
实际工程中，骨料颗粒群在突水通道中的沉积运移受较多因素的影响。基于现场实践以及前期的预试验，将骨料粒径、动水流速、投注高度以及裂隙开度（管径）作为主要影响因素，试验的水平影响因素见表2，试验方案设计见表3。
表 2 试验水平因素Table 2. Test level factor试验
水平水平因素 动水流速A/(m·s−1) 粒径B/mm 投注高度C/cm 1 0.15 0~2 40 2 2~5 50 3 0.30 5~8 70 4 8~10 90 表 3 管径50 mm和70 mm骨料颗粒群运移试验Table 3. Test for migration of aggregate particles with diameter of 50 mm and 70 mm试验
编号水平因素
分配水平因素 动水流速/(m·s−1) 粒径
/mm投注高度
/cm1-1，2-1 A1B1C1 0.15 0~2 40 1-2，2-2 A1B2C2 0.15 2~5 50 1-3，2-3 A1B3C3 0.15 5~8 70 1-4，2-4 A1B4C4 0.15 8~10 90 1-5，2-5 A2B1C4 0.30 0~2 90 1-6，2-6 A2B2C3 0.30 2~5 70 1-7，2-7 A2B3C2 0.30 5~8 50 1-8，2-8 A2B4C1 0.30 8~10 40 注：1-1为针对管径为50 mm的试验编号；2-1为针对管径为70 mm的试验编号；其余试验编号以此类推。 2.3 骨料沉积运移规律分析
以编号1—2的试验组为例进行分析，对应骨料沉积运移的全过程如图8所示。
![]()
图 8 1-2号试验骨料沉积运移全过程示意Figure 8. Whole process of sedimentation and migration of test aggregate 1-2骨料进入灌注孔，做自由落体运动与水流相接触后：一部分骨料颗粒在动水条件作用下，运动状态转变为类平抛运动，沉积至突水通道底部后，受摩擦阻力影响，滑移一段距离静止；另一部分骨料颗粒受自身质量以及下落速度的影响，水平运移距离较小，直接堆砌至灌注孔底部。在沉积运移区部分，随着阻水段的逐渐建立，突水通道内动水压力增加，沉积静止后存在于上表层的少部分骨料颗粒，受其他颗粒运动撞击，所受动力大于摩擦阻力，发生二次运动，被水流携带至阻水段末尾处，形成拖尾区。
在试验过程中，虽骨料颗粒的运动表现出较高的随机性，但修正后的理论计算模型考虑到了实际情况中大部分骨料颗粒的沉积运移状态，前文将全过程划分为3个运动阶段的假设成立。
最后就动水流速，粒径以及投注高度对于骨料沉积运移状态的影响而言：动水流速与骨料运移距离呈正相关；粒径以及投注高度与骨料的运移距离呈负相关。骨料最终所形成的沉积运移区表现为小颗粒在上，大颗粒在下，中间存在大小骨料的咬合区。
2.4 骨料正向运移距离分析
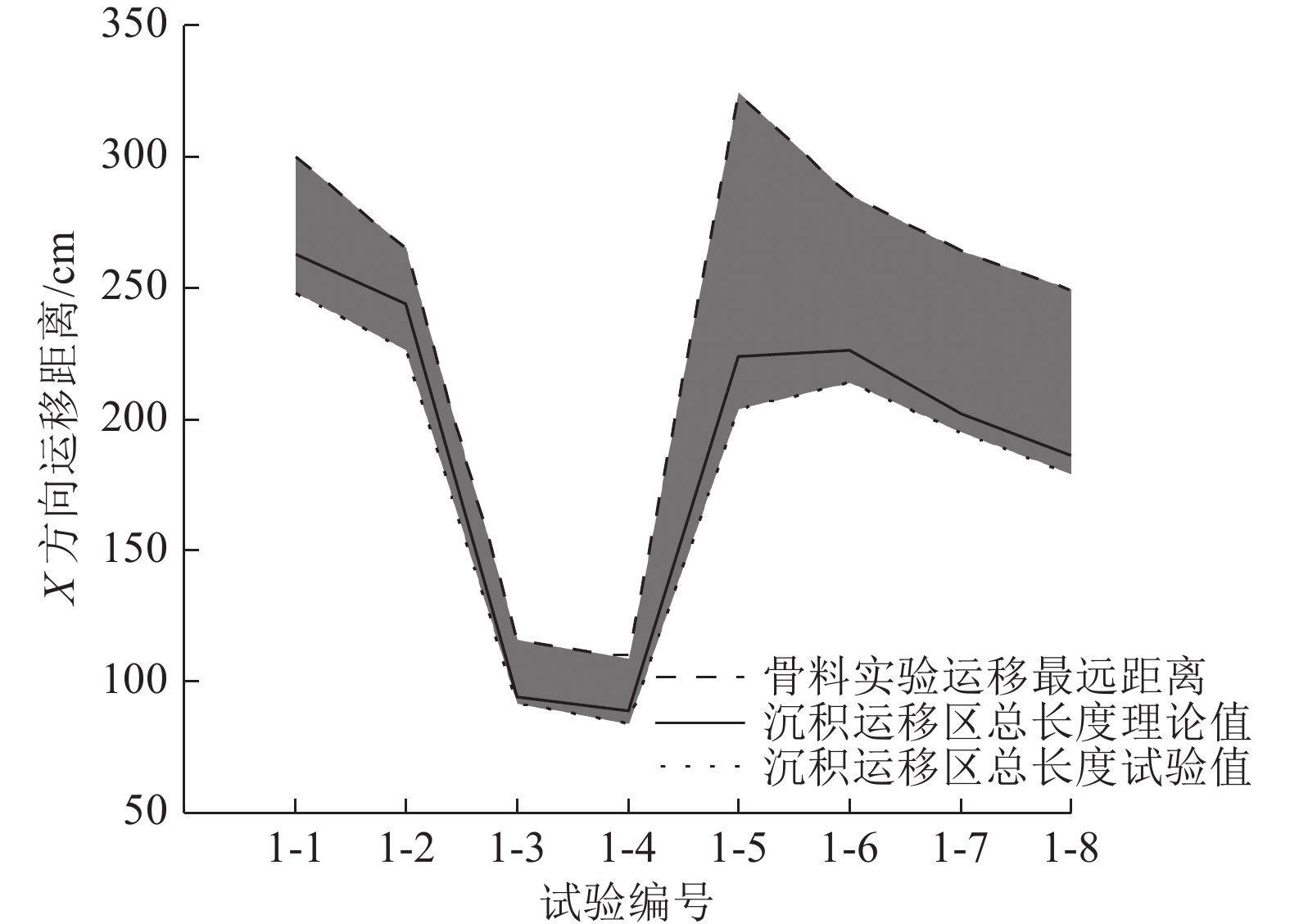
理论计算所得出的水平方向运移距离为沉积运移区（有效堵水段）的总长度，将分组试验后的结果与理论计算结果整理汇总，如图9和图10所示。
管径为50 mm的试验组中：1～5号试验组骨料运移距离最为324 cm，沉积运移区的总长度试验值为204 cm，理论计算值为224 cm，试验误差为20 cm，此组的误差率最大，为9.8%，拖尾区长度为100 cm；1～4号试验骨料运移距离最近为109 cm，沉积运移区总长度试验值为84 cm，理论计算值为89 cm，试验误差值为5 cm，拖尾区长度为25 cm。管径为70 mm的试验组中：2～6号试验骨料运移距离最远为191 cm，沉积运移区总长度试验值为144 cm，理论计算值为156 cm，试验误差值为5 cm，拖尾区实际长度为47 cm；1～4号试验X方向运移距离最小为38 cm，沉积运移区总长度为22 cm，拖尾区长度为16 cm，理论计算沉积运移区总长度为25 cm，试验误差值为3 cm，此组的误差率最大，为13.6%。1-1～1-8和2-1～2-8号试验中，只有一组试验误差率超过10%，但实际误差仅为3 cm，在理论的可接受的范围之内。
分别将试验组1-1、1-2、1-5、1-6和2-1、2-2、2-5、2-6与1-8、1-7、1-4、1-3和2-8、2-7、2-4、2-3进行对比可以发现，在相同投注高度以及动水条件作用下，较细骨料试验平均误差8.85%而言，粗骨料沉积运移区总长度的试验值与理论值更为接近，平均误差仅为5.83%，相较前者平均误差降低3.02%。就此结果，对试验全过程进行分析可以发现：粗骨料自身重力较大，在第Ⅱ，Ⅲ阶段所产生的随机性运动较少，与前文所划分的3个运动阶段吻合程度更高。
试验误差原因分析：前文对于运移规律的研究没有考虑到随着骨料颗粒的沉积，突水通道逐渐变窄，管内水压以及动水流速会有所增长，并非后期计算时所代入的固定值。
试验结果结合前文对于骨料沉积运移规律的研究可发现：在不同主要影响因素的水平组合中，沉积运移区总长度的理论计算值均略大于实际试验值，处于试验最终中所形成的拖尾区的位置，但其误差在允许范围内，前文所推导出的理论公式具有一定的适用性。在控制投注高度与动水条件一致的前提下，相较细骨料而言，理论公式对于粗骨料更具适用性。
3. 结 论
1）基于浆体管道输送理论以及泥沙运动力学，建立单颗骨料颗粒在突水通道中理想化的受力运动模型，划分自由落体、类平抛以及滑移三个运动阶段，求解其各阶段在X、Y方向上的运移距离，引入参数颗粒体积浓度，修正粒径后得出骨料颗粒群各阶段在X、Y方向上运移距离的计算公式。
2）建立煤岩体突水通道骨料运移可视化的模型试验平台，以骨料粒径、动水流速、投注高度以及裂隙开度（管径）为主要影响因素，水平组合设计试验方案。观察骨料颗粒在突水作用下实际的运动状态，验证划分为3个运动阶段的假设，测定骨料静止后所形成的沉积运移区总长度并与理论值进行对比，得出理论计算模型具有一定适用性的结论。
3）沉积运移区总长度的理论计算值均略大于试验值，平均误差为7.34%，处于试验最终所形成的拖尾区的位置。在控制投注高度与动水条件一致的前提下，相较细骨料而言，理论公式对于粗骨料更具适用性。工程中解决煤岩体突水问题时，若骨料粒径较大，可利用本文理论公式，初步估算出骨料颗粒群在水平方向的运移沉积距离，为骨料投注孔间距的确定提供参考依据。
-
![]()
图 5 煤岩体突水通道骨料运移可视化的模型试验平台
1—亚克力管道；2—管道支座；3—骨料灌注系统；4—压力传感器；5—无纸记录仪；6—电磁流量计；7—供水桶；8—恒压水泵；9—相机；10—称量装置；11—骨料回收系统
Figure 5. Model test platform for visualization of aggregate migration in coal and rock water inrush channel
![]()
图 8 1-2号试验骨料沉积运移全过程示意
Figure 8. Whole process of sedimentation and migration of test aggregate 1-2
表 1 模拟突水通道参数表
Table 1 Parameters of simulated water inrush channel
规格 材质 内径/mm 壁厚/mm 连接方式 长度 ø60 PMMA 50 10 法兰
橡胶垫1 m×5 ø80 PMMA 70 10 法兰
橡胶垫3 m×1
1 m×1
0.5 m×2
下载: 导出CSV
表 2 试验水平因素
Table 2 Test level factor
试验
水平水平因素 动水流速A/(m·s−1) 粒径B/mm 投注高度C/cm 1 0.15 0~2 40 2 2~5 50 3 0.30 5~8 70 4 8~10 90
下载: 导出CSV
表 3 管径50 mm和70 mm骨料颗粒群运移试验
Table 3 Test for migration of aggregate particles with diameter of 50 mm and 70 mm
试验
编号水平因素
分配水平因素 动水流速/(m·s−1) 粒径
/mm投注高度
/cm1-1，2-1 A1B1C1 0.15 0~2 40 1-2，2-2 A1B2C2 0.15 2~5 50 1-3，2-3 A1B3C3 0.15 5~8 70 1-4，2-4 A1B4C4 0.15 8~10 90 1-5，2-5 A2B1C4 0.30 0~2 90 1-6，2-6 A2B2C3 0.30 2~5 70 1-7，2-7 A2B3C2 0.30 5~8 50 1-8，2-8 A2B4C1 0.30 8~10 40 注：1-1为针对管径为50 mm的试验编号；2-1为针对管径为70 mm的试验编号；其余试验编号以此类推。
下载: 导出CSV
-
[1] 丁百川. 我国煤矿主要灾害事故特点及防治对策[J]. 煤炭科学技术,2017,45(5):109−114. doi: 10.13199/j.cnki.cst.2017.05.019 DING Baichuan. The characteristics prevention countermeasures of major coal mine disasters and accidents in China[J]. Coal Science and Technology,2017,45(5):109−114. doi: 10.13199/j.cnki.cst.2017.05.019
[2] 王国法,任世华,庞义辉,等. 煤炭工业“十三五”发展成效与“双碳”目标实施路径[J]. 煤炭科学技术,2021,49(9):1−8. doi: 10.13199/j.cnki.cst.2021.09.001 WANG Guofa,REN Shihua,PANG Yihui,et al. “The 13th Five-Year” development effect of coal industry and the implementation path of “Double Carbon” goals[J]. Coal Science and Technology,2021,49(9):1−8. doi: 10.13199/j.cnki.cst.2021.09.001
[3] 张立海,张业成. 中国煤矿突水灾害特点与发生条件[J]. 中国矿业,2008(2):44−46. doi: 10.3969/j.issn.1004-4051.2008.02.013 ZHANG Lihai,ZHANG Yecheng. Characteristics and occurrence conditions of coal mine water inrush disasters in China[J]. China Mining Industry,2008(2):44−46. doi: 10.3969/j.issn.1004-4051.2008.02.013
[4] 李 冰,刘见宝,任建刚,等. 水力冲孔对煤微观孔隙和结构成分影响的试验研究[J]. 煤炭科学技术,2021,49(8):131−138. doi: 10.13199/j.cnki.cst.2021.08.017 LI Bing,LIU Jianbao,REN Jiangang,et al. Experimental study on the effect of hydraulic punching on micropores and structural components of coal[J]. Coal Science and Technology,2021,49(8):131−138. doi: 10.13199/j.cnki.cst.2021.08.017
[5] 李 军. 地铁突水抢险中粗骨料注浆快速封堵数值模拟研究[D]. 徐州: 中国矿业大学, 2021: 39−56. LI Jun. Numerical simulation of rapid plugging of coarse aggregate grouting in subway water inrush emergency [D]. Xuzhou: China University of Mining and Technology, 2021: 39−56.
[6] 张二蒙,沈星宇,苗 葳,等. 奥灰顶部含水层注浆改造浆液扩散影响因素试验研究[J]. 煤炭学报,2021,46(11):3536−3549. ZHANG Ermeng,SHEN Xingyu,MIAO Wei,et al. Experimental study on the main influencing factor of slurry diffuse on in Ordovician limestone aquifer grouting reconstruction[J]. Journal of China Coal Society,2021,46(11):3536−3549.
[7] 牟 林,董书宁. 截流巷道骨料堆积体中浆液运移规律与阻水机制[J]. 地下空间与工程学报,2020,16(6):1891−1900. MOU Lin,DONG Shuning. Slurry migration law and water resistance mechanism in aggregate accumulation body of intercepting roadway[J]. Journal of Underground Space and Engineering,2020,16(6):1891−1900.
[8] 曹志国,张建民,王 皓,等. 西部矿区煤水协调开采物理与情景模拟实验研究[J]. 煤炭学报,2021,46(2):638−651. doi: 10.13225/j.cnki.jccs.2020.1173 CAO Zhiguo,ZHANG Jianmin,WANG Hao,et al. Study on physical and scenario simulation experiment of coal water coordinated mining in Western Mining Area[J]. Journal of China Coal Society,2021,46(2):638−651. doi: 10.13225/j.cnki.jccs.2020.1173
[9] 王 皓,董书宁,姬亚东,等. 煤矿水害智能化防控平台架构及关键技术[J]. 煤炭学报,2022,47(2):883−892. doi: 10.13225/j.cnki.jccs.xr21.1725 WANG Hao,DONG Shuning,JI Yadong,et al. Coal mine water disaster intelligent prevention and control platform architecture and key technologies[J]. Journal of China Coal Society,2022,47(2):883−892. doi: 10.13225/j.cnki.jccs.xr21.1725
[10] 涂昌德. 美国的输煤管道介绍[J]. 煤矿设计,1997(11):32−37. TU Changde. Introduction to coal pipelines in the United States[J]. Coal Mine Design,1997(11):32−37.
[11] DRUAND R. The hydraulic transportation of coal and solid materials in pipes [M]. Colloq of National Coal Board London, 1952: 39−52.
[12] WASP E J. 固体物料的浆体管道输送[M]. 北京: 水利出版社, 1980: 4−24. [13] WALTER Hans Graf, ALTINAKAR M S . 河川水力学[M]. 成都: 成都科技大学出版社, 1997: 77−88. [14] 费祥俊. 浆体与粒状物料输送水力学[M]. 北京: 清华大学出版社, 1994: 19−42. FEI Xiangjun. Hydraulics of conveying slurry and granular materials [M]. Beijing: Tsinghua University Press, 1994: 19−42.
[15] MATOUSEKV,VLASBLOMW J,ZWARTBOLA. Two-phase flow of highly conc-entrated slurry in a pipeline[J]. Journal of Hydrodynamics (Ser. B),2004(3):325−331.
[16] 许振良,张永吉,孙宝铮. 水平管道沉降性浆体速度分布与浓度分布关系的研究[J]. 水力采煤与管道运输,1997(3):21−27. doi: 10.14187/j.cnki.cn13-1185/tn.1997.03.004 XU Zhenliang,ZHANG Yongji,SUN Baozheng. Study on the relati-onship between velocity distribution and concentration distribution of horizontal pipeline settlement slurry[J]. Hydraulic coal mining and pipeline transportation,1997(3):21−27. doi: 10.14187/j.cnki.cn13-1185/tn.1997.03.004
[17] 李维欣. 圆型过水巷道骨料灌注模拟试验[D]. 徐州: 中国矿业大学, 2016: 32−41. LI Weixin. Simulation test of aggregate perfusion in circular roadway [D]. Xuzhou: China University of Mining and Technology, 2016: 32−41.
[18] 惠 爽. 矿井淹没巷道多孔灌注骨料封堵模拟试验[D]. 徐州: 中国矿业大学, 2018: 18−37. HUI Shuang. Simulation test of porous perfusion aggregate sealing in mine submerged roadway [D]. Xuzhou: China University of Mining and Technology, 2018: 18−37.
[19] DUBOYS M P. Lerhone et les rivieres a Lit affouillable [M]. Annales de Ponts et Cgausses, 1879, 18(5): 41−195.
[20] Rouse H. Experiments on the mechanics of sediment suspension [C]. Proceedings of the 5th international congers for applied mechanics, 1938: 166−198.
[21] BAGNOLD R A. An approach to the sediment transport problem from general physics[M]. Washington, D C: Geological Survey Professional Paper, 1966: 213−289.
[22] ENGELUND F A,FREDSOE J. Sediment ripples and dunes[J]. Annual Review of Fluid Mechanics,2003,14(1):13−37.
[23] 张瑞瑾. 河流泥沙动力学[M]. 北京: 中国水利水电出版社, 1989: 46−63. ZHANG Ruijin. River sediment dynamics [M]. Beijing: China Water Conservancy Hydropower Press, 1989: 46−63.
[24] 窦国仁. 再论泥沙起动流速[C]//中国水利学会2001学术年会论文集, 2001: 321−327. DOU Guoren. Further discussion on sediment incipient velocity [C]. Papers of the 2001 Academic Annual Meeting of the Chinese Water Conservancy guild, 2001: 321−327.
[25] 韩其为, 何明民. 泥沙运动统计理论[M]. 北京: 科学出版社, 1984: 88−129. HAN Qiwei, HE Mingmin. Statistical theory of sediment movement [M]. Beijing: Science Press, 1984: 88−129.
[26] 卢 勇. 管道固—液两相浆体输送理论研究[D]. 长沙: 湖南大学, 2015: 26−39. LU Yong. Pipeline solid-liquid two-phase slurry transportation theory research [D]. Changsha: Hunan University, 2015: 26−39.
[27] 匡翠萍,郑宇华,顾 杰,等. 泥沙颗粒团沉速[J]. 同济大学学报(自然科学版),2016,44(12):1845−1850,1866. doi: 10.11908/j.issn.0253-374x.2016.12.006 KUANG Cuiping,ZHENG Yuhua,GU Jie,et al. Sedimentation rate of sediment pellets[J]. Journal of Tongji University (Natural Science Edi-tion),2016,44(12):1845−1850,1866. doi: 10.11908/j.issn.0253-374x.2016.12.006
计量
- 文章访问数: 77
- HTML全文浏览量: 7
- PDF下载量: 23
