Mechanism, potential and regulation of carbon sequestration and sink enhancement in ecological restoration of mining areas in the Loess Plateau
-
摘要:
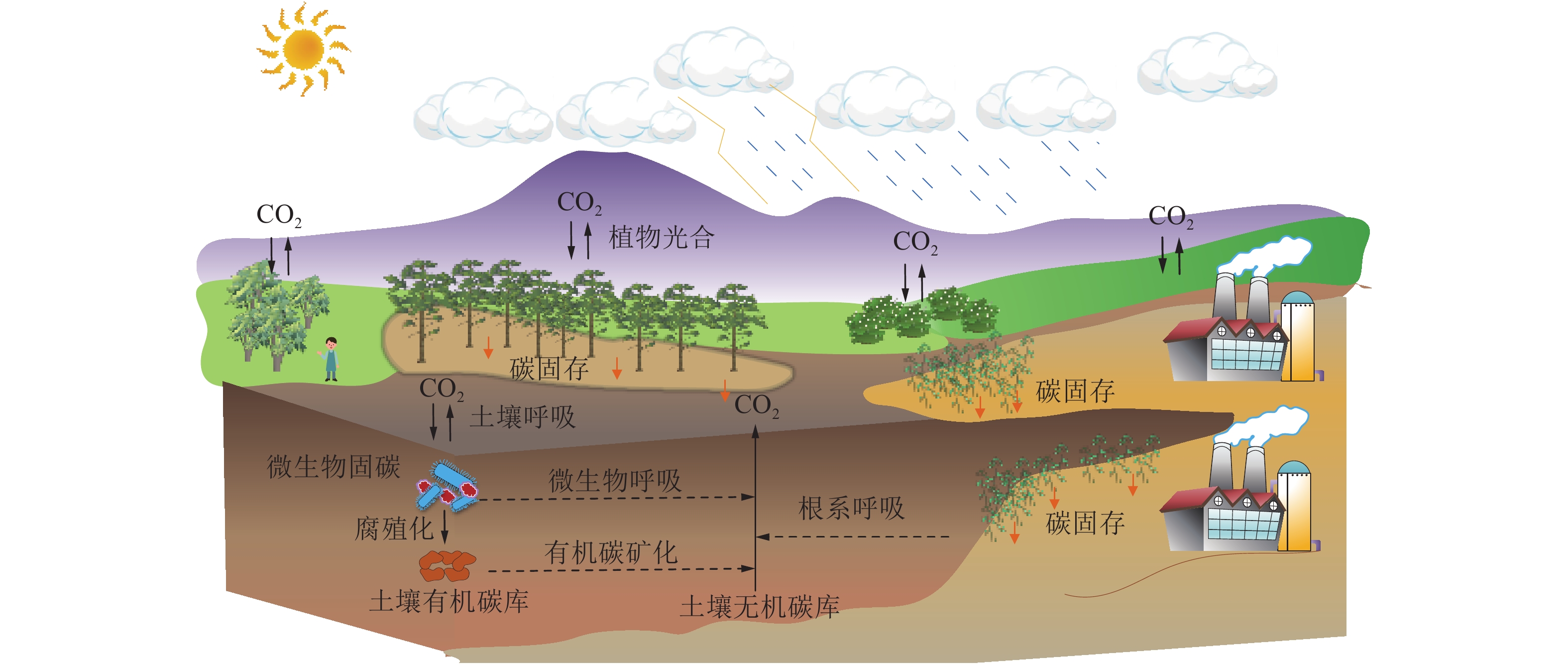
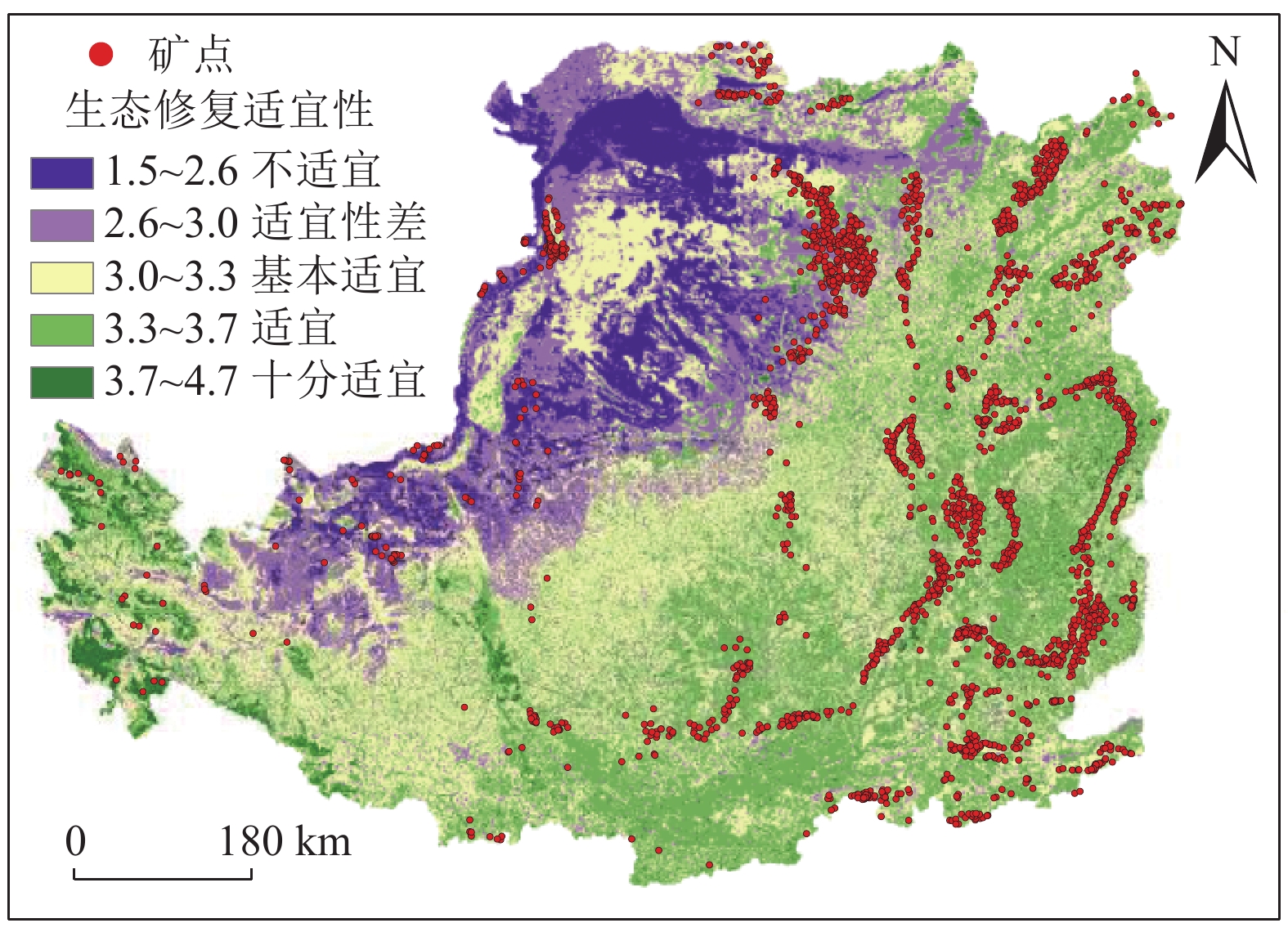
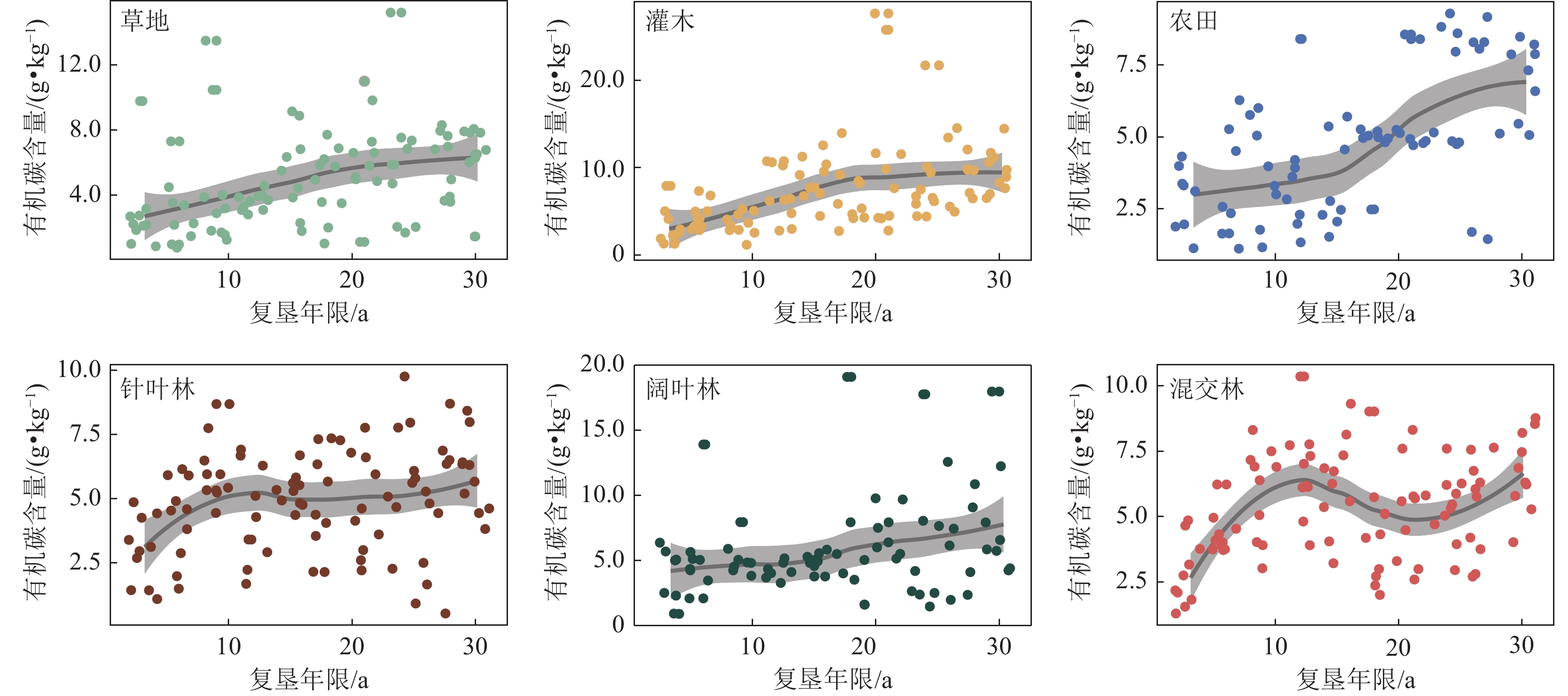
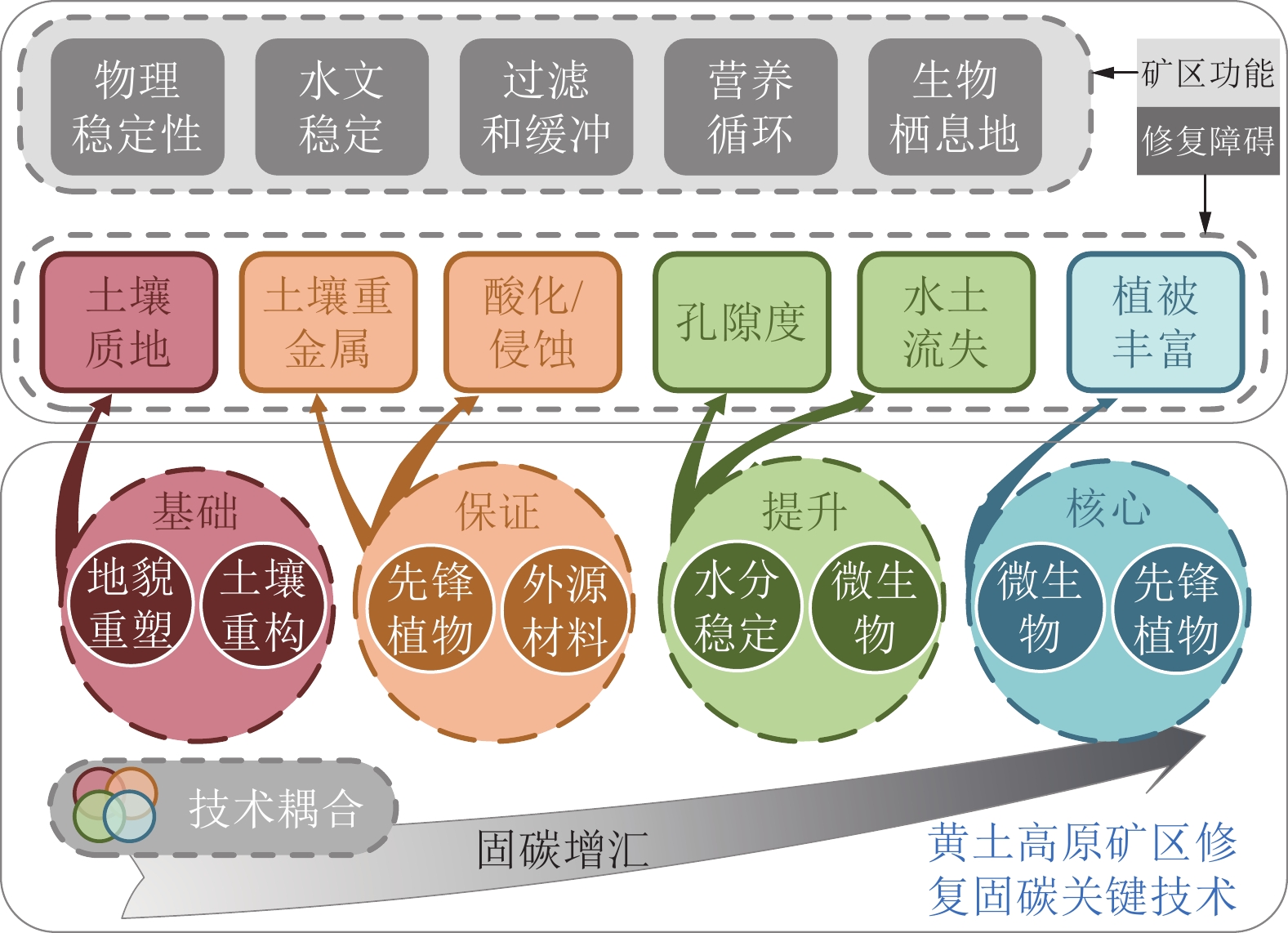
黄土高原矿区生态环境脆弱，科学认识黄土高原矿区生态修复固碳增汇机制、潜力对碳中和愿景目标至关重要。为此，需探究黄土高原矿区生态修复中碳汇形成的关键过程，修复的适应性和恢复力及增汇潜力，揭示矿区复垦土壤碳库稳定性机制，最终阐明矿区生态修复中固碳增汇的关键技术。结果表明：①黄土高原矿区生态修复碳汇形成的关键过程包含植物光合碳分配、土壤碳固持、微生物固碳和土壤呼吸等；②黄土高原矿区生态修复适应性总体呈东南高、西北低，适应性较差区主要分布于陕北与内蒙古和宁夏中部与内蒙古交界处。典型矿区生态恢复力指数为混交林（43.2%~100.0%）>阔叶林（49.2%~83.2%）>针叶林（47.9%~76.5%）>草地（39.1%~70.7%）>灌木草地（43.0%~69.0%）。修复年垦与土壤碳汇潜力呈正相关关系，修复10~15年时最高，混交林生态恢复耗时最长，但固碳潜力最大；③复垦土壤碳库稳定性机制与凋落物分解、黏土矿物交互作用、团聚体物理保护和微生物调控有关；④地貌重构-土壤重建-先锋植物/微生物-外源材料相耦合修复技术是黄土高原矿区生态修复固碳增汇的最优路径，有利于碳汇的长期稳定和提升。
Abstract:The ecological environment of mining areas in the Loess Plateau is extraordinary fragile, so it is crucial to achieve the carbon neutral vision to scientifically understand the mechanism and potential of carbon sequestration and sink enhancement in ecological restoration of mining areas in the Loess Plateau. Therefore, it is necessary to explore the key processes of carbon sink formation, the adaptability and resilience of restoration, and the potential of increasing carbon sink in the ecological restoration of the mining area in the Loess Plateau, as well as reveal the stability mechanism of the carbon pool in the reclaimed soil of the mining area. Finally, the key technologies of carbon fixation and sink enhancement in the ecological restoration of the mining area are clarified and elucidated. The results showed that: ① The critical processes of carbon sink formation in ecological restoration of mining areas in the Loess Plateau included plant photosynthetic carbon allocation, soil carbon sequestration, microbial carbon sequestration, soil respiration and so on; ② The ecological restoration adaptability of the mining areas in the Loess Plateau generally presented a trend of high in the southeast and low in the northwest, while the areas with poor adaptability were mainly distributed at the border between northern Shanxi and Inner Mongolia, as well as between central Ningxia and Inner Mongolia. The order of ecological resilience of typical mining areas was shown as follows: Mixed forest (43.2%-100.0%) > Broad-leaved forest (49.2%-83.2%) > Coniferous forest (47.9%-76.5%) > Grassland (39.1%-70.7%) > Shrub grassland (43.0%-69.0%). There was a positive correlation between the remediation years and soil carbon sink potential. The highest carbon sink potential generally occurred at 10-15 years of restoration. The mixed forests spent the longest ecological restoration time, but possessed the largest carbon sequestration potential; ③ The stability mechanism of reclaimed soil carbon pool was related to litter decomposition, clay mineral interaction, aggregate physical protection and microbial regulation; ④ The coupling repair technology including landform reconstruction, soil reconstruction, pioneer plant/microorganism and exogenous material is the optimal path for ecological restoration of coal mining areas in the Loess Plateau to fix carbon and increase carbon sink, and it is also conducive to the long-term stability and improvement of carbon sink.
-
0. 引 言
地下煤矿开采极易导致岩体移动变形，诱发地面沉降、山体滑坡、基础设施破坏、生态恶化等系列地质环境问题[1-2]。因此，准确认知上覆岩层及地表复杂的移动变形过程，并精准预测其演化趋势是矿山地质灾害精准防控的关键[3-4]。目前，煤矿区地表动态移动变形预测方法主要有物理力学法和时间函数法[2-3]。在两类方法中，物理力学法能够顾及矿山岩体物理力学变化过程，物理意义明确，但其高度依赖矿区地质条件刻画精细度和上覆岩体力学参数的可靠性，很大程度限制了该方法的实际应用。时间函数法是一种将描述地表单点动态沉降过程的时间函数（单点“动态”）与开采导致的最终沉降盆地（面域“静态”）融合形成的数学方法。该方法可通过历史形变观测值估计数学模型参数，并据此预测地表潜在移动变形趋势。由于时间函数法无需已知上覆岩层力学参数，因此被广泛应用于矿区地表动态移动变形预测[5-9]。
在时间函数法中，用于描述地表单点动态沉降过程的时间函数选取对于预测结果精度和适用范围起至关重要的作用。已有研究表明：长壁采煤（目前应用较多的地下采煤方法）引起的地表单点动态沉降基本服从“S”型增长。因此，Knothe函数[10]、Weibull函数[11]、Logistic函数[12]、Richards函数[13]、Boltzmann函数[14]和Bertalanffy函数[15]等“S”型增长数学模型被相继用于描述长壁开采导致的地表单点动态沉降过程，并据此修正时间函数法，取得了一定的成效。
然而，“S”型增长数学模型众多，且形式各异[16]。现有研究大都以拟合残差最小化（即强拟合性）为指标，以单个矿区少量沉降观测为样本，修正或引入“S”型数学模型以期提高时间函数法的预测精度[15-17]。该方式因过度追求拟合残差最小而易出现“过拟合”或“过度参数化”现象，进而增加构建模型的复杂度，极大提高参数反演难度。理论上，最优化时间函数模型应同时具备以下特征：①较好地描述地表单点沉降过程（强拟合性）；②适用于不同的地质采矿条件（高适应性）；③模型参数尽可能少（低复杂性）。因此，从时间函数优化选取角度来讲，现有研究不仅容易出现“过拟合”，而且以单个矿区少量观测样本为基础验证模型可靠性也容易出现所选模型在特定矿区应用效果较好，而适应性不高（即未考虑“高适应性”准则）。
鉴于此，引入了拟合残差和模型复杂度2个关键指标，通过选取不同地质采矿条件的大样本分析时间函数的优化选取。具体地，首先选取12种常见的“S”型函数模型为研究对象，推导和分析各模型的特征值与煤矿区地表单点动态沉降理论特征的差异。12种模型包含了已被引入时间函数法的7种模型以及目前尚未被引入但在生物种群增长等领域广泛使用的5种模型。在此基础上，以来自7个不同地质采矿条件煤矿区的103个地表时序沉降观测值为样本，利用赤池信息量准则（Akaike Information Criterion, AIC）系统地对比分析各模型的适应性、拟合优度和复杂度差异，并据此给出煤矿区地表单点动态沉降时间函数优化选取建议。研究有助于拓展时间函数法的应用范围，提高动态沉降预测精度，推动矿山动态地质灾害精准防控，丰富矿区开采沉陷理论。
1. 长壁采煤区地表单点沉降演化过程
地下长壁采煤导致的地表单点动态沉降服从“S”型函数增长，即该点大致经历初始沉降、加速沉降与残余沉降3个阶段[18]。如图1所示，在初始阶段，当地下开采即将影响到指定地表点时（
$t = 0$ ），该点的沉降量$W(t)$ 、沉降速度$v(t)$ 和加速度$a(t)$ 均为0；当地下开采开始影响该地表点时（$t > 0$ ），沉降逐渐显现，沉降速度逐渐增大，且沉降加速度逐渐增加到最大值；随着地下工作面继续推进，该点进入加速阶段，$W(t)$ 继续增大，$v(t)$ 逐渐加快至峰值并逐渐减缓，且沉降曲线拐点处下沉速度达到最快，加速度最小（此时$v(t) = {v_{\max }}$ 、$a(t) = 0$ ）；之后，随着时间的增长（$t \to \infty $ ），该点逐渐进入衰减阶段，沉降量$W(t)$ 继续增大且趋于常数，$v(t)$ 和$a(t)$ 渐趋于0，直至该点最终稳定。![]()
图 1 长壁采煤导致的地表单点沉降量、沉降速度和加速度演化过程示意Figure 1. Schematic of temporal evolution of subsidence, velocity and acceleration at single surface point caused by longwall coal mining2. 时间函数优化选取方法
为分析各模型的可靠性和复杂性，首先选取12种模型作为研究对象，其中包括目前已被引入时间函数法的7个模型（即Knothe、Weibull、Logistic、Bertalanffy、Richards、Gompertz和Boltzmann）以及目前尚未被引入时间函数法但在生物增长等领域常用的5个模型（即Hossfeld、Leavokic-I、Leavokic-III、Yoshida和Sloboda）。在12种模型中按参数个数分为二参数、三参数和四参数模型，其中，Knothe为二参数模型，Weibull、Logistic、Bertalanffy、Gompertz、Hossfeld和Leavokic-III（简称“Lea-III”）为三参数模型，而Leavokic-I（简称“Lea-I”）、Sloboda、Yoshida、Richards和Boltzmann为四参数模型。之后，将从理论分析和AIC准则辅助指导2个角度分析时间函数的优化选取。
2.1 理论特征分析
长壁采煤导致的地表不同点位动态沉降虽然在量级和过程等方面存在差异（与地质采矿条件相关），但正如第一节所述，各点在初始时刻（
$t = 0$ ）和稳定后（$t = + \infty $ ）的关键沉降量、沉降速度和加速度特性是一致的，即$W(t = 0) \equiv 0$ 、$v(t = 0) \equiv 0$ 、$a(t = 0) \equiv 0$ 、$W(t = + \infty ) \equiv $ C（C为常数）、$v(t = + \infty ) \equiv 0$ 和$a(t = + \infty ) \equiv 0$ ，本节将据此分析所选12种模型在$t = 0$ 和$t = + \infty $ 时的主要特征。为此首先根据12种所选模型的动态沉降表达式推导其对应的速度和加速度的表达式，并计算了其在$t = 0$ 和$t = + \infty $ 时的特征值，结果见表1。表 1 12种“S”型增长模型在$t = 0$ 和$t \to \infty $ 时的沉降量、速度和加速度特征Table 1. Comparison of subsidence, velocity, and acceleration of the selected 12 “S-shaped” time functions at the dates$t = 0$ and ,$t \to \infty $ respectively时间函数 下沉量$ y $ 下沉速度$ y' $ 下沉加速度$y''$ $ t=0 $ $ t \rightarrow \infty $ y y' y'' y y' y'' Knothe $y=a\left(1-{\rm{e}}^{-c t}\right)$ $y'=a c {\rm{e}}^{-c t}$ $y''=-a c^{2} {\rm{e}}^{-c t}$ 0 ac $ -a c^{2} $ a 0 0 Weibull $y=a\left(1-{\rm{e}}^{-b t^c}\right)$ $y'=a b c t^{c-1} {\rm{e}}^{-b t^{c} }$ $y''=a b c {\rm{e}}^{-b t^c} t^{c-2}\left[(c-1)-b c t^c\right]$ 0 0 0 a 0 0 Logistic $y=\dfrac{a}{1+b {\rm{e}}^{-c t} }$ $y'=\dfrac{a b c}{{\rm{e}}^{c t}\left(b {\rm{e} }^{-c t}+1\right)^2}$ $y''=\dfrac{a b c^2}{{\rm{e}}^{c t}\left(b {\rm{e}}^{-c t}-1\right)^2}\left[\dfrac{2 b}{{\rm{e}}^{c t}\left(b {\rm{e}}^{-c t}+1\right)}-1\right]$ $ \dfrac{a}{1+b} $ $ \dfrac{a b c}{(1+b)^{2}} $ $ \dfrac{a b c^{2}(c-1)}{(1+b)^{3}} $ a 0 0 Gompertz $y=a {\rm{e}}^{1-b {\rm{e}}^{-c t} }$ $y'=a b c {\rm{e} }^{-c t} {\rm{e}}^{\left(1-b {\rm{e} }^{-c t}\right)}$ $ y''=a b c^2 e^{-c t} e^{\left(1-b e^{-c t}\right)}\left(b e^{-c t}-1\right)$ $a {\rm{e}}^{1-b}$ $a b c {\rm{e}}^{1-b}$ $a b c^2(b-1)^* {\rm{e}}^{1-b}$ ae 0 0 Bertalanffy $y=a\left(1-b {\rm{e}}^{-c t}\right)^{-3}$ $y'=-3 a b c {\rm{e}}^{-c t}\left(1-b {\rm{e}}^{-c t}\right)^{-4}$ $\begin{aligned}y''= & 3 a b c^2 {\rm{e} }^{-c t}\left(1-b {\rm{e} }^{-c t}\right)^{-4}+ \\& 12 a b^2 c^2 {\rm{e} }^{-2 c t}\left(1-{\rm{e} }^{-c t}\right)^{-5}\end{aligned}$ $ \dfrac{a}{(1-b)^{3}} $ $ \dfrac{-3 a b c}{(1-b)^{4}} $ $ \dfrac{3 a b c^{2}}{(1-b)^{4}} $ a 0 0 Hossfeld $ y=\dfrac{t^c}{b+t^c / a}$ $y'=\dfrac{b c t^{c-1}}{\left(b+t^c / a\right)^2}$ $y''=y'\left[\dfrac{c-1}{t}-\dfrac{2 c t^{c-1} }{a\left(b+t^c / a\right)}\right]$ 0 0 0 a 0 0 Leavokic-Ⅲ $y=a\left(\dfrac{t^2}{b+t^2}\right)^c $ $ y'=\dfrac{2 b c y}{t\left(b+t^2\right)} $ $y''=2 b c \dfrac{t\left(b+t^2\right) y'-\left(3 t^2+b\right) y}{t^2\left(b+t^2\right)^2}$ 0 0 0 a 0 0 Leavokic-Ⅰ $ y=a\left(\dfrac{t^d}{b+t^d}\right)^c $ $y'=b c d \dfrac{y}{t\left(b+t^d\right)}$ $y''=\dfrac{t\left(b+t^d\right) y'-\left[(d+1) t^d+b\right] y}{t^2\left(b+t^d\right)^2}$ 0 0 0 a 0 0 Yoshida $y=\dfrac{a t^d}{b+t^d}+c $ $ y'=\dfrac{t^{d-1}}{\left(b+t^d\right)^2} $ $y''=\dfrac{t^{d-2} }{\left(b+t^d\right)}\left[(d-1)-\dfrac{2 d t^d}{b+t^d}\right]$ c 0 0 a+c 0 0 Sloboda $y=a {\rm{e}}^{-b {\rm{e}}^{-c t^d} }$ $y'=b c d t^{d-1} {\rm{e}}^{-c t^d} y$ $\begin{array}{l}y'' = {t^{d - 1} }{{\rm{e}}^{ - c{t^d} } }y' + \\\left[ {(d - 1){t^{d - 2} } - dc{t^{2d - 2} } } \right]{{\rm{e}}^{ - c{t^d} } }y\end{array}$ $a {\rm{e}}^{-b}$ 0 0 a 0 0 Richards $y=a\left(1-b {\rm{e}}^{-c t}\right)^{\dfrac{1}{1-d} }$ $\begin{array}{l}y' = {\left( {1 - b{ {\rm{e} }^{ - ct} } } \right)^{\frac{d}{ {1 - d} } } }\\{ {\rm{e} }^{ - ct} } y/(1 - d)\end{array}$ $\begin{array}{l}y'' = abc{\left[ {b{ { {{\rm{e}}} }^{ - ct} } - (1 - d)} \right]\times}\\{ {\rm{e} }^{ - ct} }{\left( {1 - b{e^{ - ct} } } \right)^{\left( {\frac{1}{ {1 - d} } - 2} \right)} }/{(1 - d)^2}\end{array}$ $a(1-b)^{\dfrac{1}{1-d}}$ $\dfrac{a b c(1-b)^{\dfrac{d}{1-d}}}{(1-d)}$ $\dfrac{a b c(b-1+d)(1-b)^{\left(\dfrac{1}{1-d}-2\right)} }{(1-d)^2}$ a 0 0 Boltzmann $y=b+\dfrac{a-b}{1+{\rm{e} }^{(t-c) / d} }$ $y'=\dfrac{(a-b) {\rm{e}}^{[(t-c) / d)]} }{d\left[1+{\rm{e}}^{(t-c) / d}\right]^2}$ $y''=y' \dfrac{{\rm{e}}^{((c-t) / d)}-1}{d {\rm{e}}^{[(c-t) / d+1]} }$ $\dfrac{a+b {\rm{e}}^{-\dfrac{c}{d} } }{{\rm{e}}^{-\dfrac{c}{d} }+1}$ $\dfrac{(a-b) {\rm{e}}^{-\dfrac{c}{d} } }{d \left({\rm{e}}^{-\dfrac{c}{d} }+1\right)^2}$ $\dfrac{{\rm{e}}^{(c / d-1)} }{{\rm{e}}^{(c / d+1)^3} } \dfrac{(a-b) {\rm{e}}^{(c / d} )}{d^2}$ a 0 0 注：a、b、c、d为时间函数模型参数。 从表1中可以看出，当
$t = + \infty $ 时，所选的12种模型均能满足沉降量、沉降速度和加速度理论特征（即$W(t = + \infty ) \equiv $ C（C为常数），$v(t = + \infty ) \equiv 0$ 和$a(t = + \infty ) \equiv 0$ ）。然而，当$t = 0$ 时，Weibull、Hossfeld、Lea-I和Lea-III模型的沉降值、沉降速度和加速度同时为零，Yoshida和Sloboda模型的初始沉降以及Knothe模型的初始速度和加速度为0，而Logistic、Gompertz、Bertalanffy、Richards和Boltzmann的初始沉降量、沉降速度和加速度均不为0。该结果表明，仅Weibull、Hossfeld、Leavokic-I和Leavokic-III能严格满足煤矿区地表单点在$t = 0$ 和$t = + \infty $ 时的动态沉降、沉降速度和加速度特征。2.2 基于AIC准则的时间函数优化选取
如前所述，最优化时间函数应同时兼顾强拟合性、高适应性和低复杂性3个关键指标。然而，现有研究大都仅通过拟合误差（Fitting Error）和
${R^2}$ 等拟合指标指导时间函数优化选取（即仅追求强拟合性），并未同时考虑强拟合性、高适应性和低复杂性之间的平衡。事实上，非线性模型强拟合性与低复杂性（即模型参数个数）之间通常是矛盾的，即追求强拟合性可能以增加模型参数个数（即复杂性）为代价，导致过拟合或“过度参数化”现象，进而产生模型参数难以准确反演或参数反演不稳定等关键问题。因此，如何能够平衡强拟合性和低复杂性2个重要指标是模型优化选取的关键。AIC准则是一种权衡所估计模型的复杂度和模型拟合优度有效方法[19-21]。该方法首先假设模型拟合误差服从正态分布，并在“熵”概念的基础上建立了AIC指标估计表达式[22]：
$$ A_{\rm{IC}} = {\rm{ln}}\frac{{R_{\rm{SS}}}}{n} + 2k $$ (1) 式中，
$R_{\rm{SS}}$ （Residual Sum of Squares）为残差平方和；$n$ 为样本数量；$k$ 为模型参数个数。从式（1）可以看出，AIC指标在考虑模型拟合可靠性（用$R_{\rm{SS}}$ 表示）的同时附加参数惩罚项，以此避免对数据的过拟合（Over-fitting）。在AIC准则中，最小AIC值对应的模型即被认为是当前观测样本下拟合可靠性和复杂性的最佳平衡模型。然而，受观测样本数量、观测误差和拟合误差等因素影响，AIC估计值通常含有误差。为减小误差对模型优化选取的影响，依据统计分级思想，通过以不同模型AIC值与最小值之差
$\Delta $ AIC（即$\Delta $ AIC(i)=AIC(i)−min(AIC), i=1, 2, 3, ···, 12）为分级标准[23]（具体见表2），依次将各个模型划分至3个不同的推荐使用等级（Level-I、Level-II和Level-III，其中Level-I为最优化模型），并据此分析12种模型在煤矿区地表单点动态沉降过程描述中的优化选取。表 2 基于$\Delta $ AIC分类参照标准Table 2. Reference based on$\Delta $ AIC classification水平 $\Delta $AIC 差异强弱 推荐使用等级 Level-I 0～2 弱差异 最高 Level-II 2～6 中等差异 适中 Level-III >6 强差异 较低 3. 真实数据分析与结果
3.1 试验数据概况
为顾及时间函数优化选取中的高适应性指标，在7个不同地质采矿条件长壁采煤矿区（详细信息见表3）收集了103个地表动态沉降观测值作为“S”型时间函数优化选取的样本。其中，7个矿区涵盖了我国比较常见的软弱、中硬和坚硬覆岩力学特征，且松散层厚度也涵盖了薄松散层到巨厚松散层的特征变化。来自不同地质采矿条件的大观测样本有助于验证时间函数优化选取的强适应性。同时，AIC准则的应用有助于选取12种模型中具有强拟合性和低复杂性的最优平衡模型，从而实现煤矿区单点动态沉降时间函数的优化选取（即同时兼顾高可靠性、强适应性和低复杂性）。
表 3 103个沉降观测值样本所处矿区的松散层和覆岩力学特征对比Table 3. Lithology of the seven interesting mining areas where subsidence observations at 103 surface points were collected矿区信息 松散层厚度 覆岩岩性 观测点数量 东营市某矿 巨厚松散层 中硬 26 宿州市某矿 厚松散层 中硬 15 榆林市某矿 厚黄土层 中硬 7 淮北市某矿 厚松散层 软弱 7 唐山市某矿 厚松散层 中硬 16 阳泉市某矿 薄松散层 坚硬 17 丰城市某矿 薄松散层 中硬 15 3.2 试验结果
在利用AIC准则优化选取时间函数之前，准确估计12种时间函数模型的参数至关重要。考虑到12种模型均具有高度非线性特征，因此使用遗传算法[24]（Genetic Algorithm, GA）和Levenberg-Marqurdt算法（LM）串行的非线性全局优化算法估计模型参数。具体地，为增大全局选优能力，首先设置遗传算法种群数量为20 000，最大迭代次数为5 000，交叉概率为0.7。根据该参数设定并利用遗传算法分别反演103个样本点处12个“S”型增长模型参数。之后，以遗传算法估计值作为初始参数，利用LM算法精化参数，提升全局反演能力。
图2展示了7个矿区随机选取的一个观测样本点处12个模型的拟合对比。从图2可以看出，除二参数Knothe模型外，其余11个模型基本能够描述随机选取的7个样本点的动态沉降过程。然而，从图2还可看出，除Knothe外的11个模型对于所展示的观测样本拟合优度仍存在差异，因此需着重研究描述煤矿区地表单点动态沉降过程的时间函数优化选取。
![]()
图 2 12种模型在7个矿区地表单个观测样本点动态沉降的拟合效果对比Figure 2. Comparison of 12 models for fitting time-series subsidence observations at single point in seven different mining areas为实现时间函数优化选取，利用式（1）计算了12种模型在各观测样本点的AIC值以及模型间的
$ \Delta $ AIC值。之后，参照表2所列$\Delta $ AIC分级规则将同一观测样本点的12种模型分级（即Level-I、II和III）。图3展示了12种“S”型模型在103个观测样本点所对应的不同分级所占比例。由图3可知，12种模型的$\Delta $ AIC均存在Level-I、II和III的分级。换句话说，面对不同地质采矿条件，12种模型均难以完美描述所有样本点的动态沉降过程（即Level-I占比为100%）。![]()
图 3 12种模型的$\Delta $ AIC分级占比统计Figure 3. Percent of selected 12 models at Level-I, II, and III based on$\Delta $ AIC estimates然而，从图3中可以看出，Hossfeld模型被划入Level-I等级的比例最高，达到了57.3%，且Level-I和Level-II高达87.3%。该结果表明，在12种时间函数模型中，Hossfeld模型能够较好地兼顾高适应性、强拟合性和低复杂性3个重要的优化选取指标。此外，从2.1节的理论分析可知，Hossfeld模型在
$t = 0$ 和$t = + \infty $ 时的曲线特征与长壁采煤导致的地表沉降过程理论特征值完全一致。因此，从兼顾强适应性、高可靠性和低复杂性的角度而言，Hossfeld模型（目前尚未被引入）可作为时间函数法中描述地表单点动态沉降过程的优化模型。4. 讨 论
4.1 覆岩结构与岩性对时间函数选取的影响
由3.2节的分析可知，在
$\Delta $ AIC分级规则下，Hossfeld模型在大部分（占比87.3%）观测样本中被划分至Level-I或II等级，是所有模型中占比最高的。然而，需要注意的是，在103个样本点中，Hossfeld模型在13个观测样本点（约占12.6%）被划定Level-III等级（即其他模型的$\Delta $ AIC指标比Hossfeld模型更优）。为进一步分析Hossfeld模型被划分为Level-III的原因，按照上覆岩层的岩性（即软、中硬和坚硬）和松散层厚度（薄、厚和巨厚）将13个Level-III等级的观测样本点分类。分析发现在13个Level-III等级的观测样本中，绝大部分点（8个观测样本点）位于坚硬覆岩和薄松散层覆盖的阳泉某矿区。图4显示了阳泉某矿区两个典型动态沉降观测序列以及Hossfeld（处于Level-III水平）和Weibull（处于Level-I水平）2个模型的拟合结果，图中动态沉降为阳泉某矿区随机抽取2个样本点的动态沉降观测值。由图4可知，Hossfeld模型对于样本点的沉降初始阶段（50~100 d）和残余沉降阶段描述效果均不及Weibull模型。根据式（1）可知，在相同的模型参数个数（Hossfeld和Weibull模型均为三参数）和时序沉降观测值的前提下，Weibull模型的拟合残差越小，其对应的AIC值也越小。因此，在
$\Delta $ AIC分级规则下即被划入了更优等级。![]()
图 4 Level-III等级下Hossfeld和Weibull模型的拟合效果对比Figure 4. Comparison diagram of fitting effects of Hossfeld and Weibull models at Level-III Level造成Hossfled模型被划入Level-III的主要原因如下。相较于较软或中硬上覆岩层，坚硬覆岩和薄松散层的地质条件对于上覆岩层的支撑力更强，因此其地表点位达到最大下沉速度和最大沉降量的时间较为滞后。从表1中可以发现，Hossfeld模型动态沉降“拐点”（即最大沉降速度发生时刻）处的沉降值约为最大沉降的37%，而Weibull模型则可达50%。这就意味着，Weibull模型对于拐点出现较为滞后（比如坚硬覆岩和/或薄松散层）的描述效果比Hossfeld模型更好。因此，坚硬覆岩和薄松散层地质条件下可优选Weibull模型作为优化时间函数。
4.2 仅考虑拟合残差的时间函数选取
如前所述，AIC准则实际上是通过附加参数惩罚项以避免“过拟合”问题，基于AIC准则选取的时间函数能够在保证拟合精度的同时最简化函数模型，提高计算效率和参数反演稳健性。为对比不同准则对于时间函数模型选取的影响，本节仅考虑强拟合性（即绝大部分现有研究选取的模型优选准则）分析12种“S”型增长模型的拟合效果和模型选取。具体地，利用3.2节中所述的算法反演103个样本点处12种“S”型增长模型参数，计算观测值与拟合值之间的均方根误差（Root Mean Square Error，RMSE），该值越小，拟合优度越好，反之亦然。图5显示了12种函数模型在各样本点的拟合均方根误差统计直方图。
![]()
图 5 12种选取模型在选取的样本点的拟合均方根误差统计直方图Figure 5. Frequency of root mean square errors of the selected 12 “S-shaped” models for fitting the dynamic subsidence at the selected 103 observation points由图5可知，除Knothe函数外，其余函数的拟合均方根误差绝大部分集中在0~0.05 m，其中四参数模型（Lea-I、Yoshida、Sloboda、Richards、Boltzmann）拟合均方根误差在此区间的平均样本点数为85个，占比82.5%；三参数模型（Gompertz、Bertalanffy、Hossfeld、Lea-III、Weibull和Logisitic）拟合均方根误差在0~0.05 m区间平均样本点数约77个，占比74.8%，略低于四参数模型。为进一步分析12种模型的拟合优度差异，采用“分位数断点法”将每个点的拟合优度差异分为3个等级。具体地，对于任意样本点，分位数断点法首先将所有模型在该点的拟合均方根误差按照从小到大排序。然后以33.3%、66.6%和100%三个分位数值作为分段“断点”，并据此将各模型划定为Level-I（[0~33.3%)）、Level-II（[33.3%~66.6%)）和Level-III（[66.6%~100%]）三个等级。其中，Level-I表示该模型在12种“S”型增长模型中拟合优度最好，Level-II次之，Level-III较差。
图6展示了12种模型在103个观测样本点被划定为Level-I到III的相对比例。由图6可知，12种“S”型模型中四参数模型（即Lea-I、Yoshida、Slobada、Richards和Boltzmann模型）的总体拟合优度（Level-I的平均比例为42.7%）要高于三参数（Level-I平均比例为27.7%）和二参数模型（Level-I平均比例为5.08%）。事实上，该结果也是预期的，因为相较于二参数和三参数模型，四参数“S”型函数增加了未知数个数，并且可通过调整参数值减少样本的拟合残差。
![]()
图 6 12种模型拟合均方根误差划定的Level-I、Level-II和Level-III的占比对比Figure 6. Comparison of the RMSE levels (I, II, and III) of the selected 12 models此外，从图6中还可以看出，相较于其它四参数模型，Slobada整体拟合精度较高（Level-I和Level-II占比达到了87.5%）；相较于其他三参数模型，Hossfeld的整体拟合精度最高（Level-I和Level-II占比达到了87.2%）。然而，通过对比Slobada和Hossfeld模型拟合优度发现，2个模型被划定为Level-I和Level-II的比例相当（仅相差0.3%）。此外，从图5中可以看出，Slobada模型平均拟合均方根误差为0.0315 m，仅比Hossfeld模型（0.0368 m）低5.3 mm。换句话说，四参数Slobada模型以增加模型复杂性（新增一个未知参数）为代价，但其平均拟合精度仅提高了5.3 mm。考虑到采煤沉陷区地表最大沉降量级通常可达几十甚至数百厘米，因此，5.3 mm的精度改善对于拟合结果影响甚微。
5. 结 论
1）Weibull、Hossfeld、Lea-I和Lea-III模型能完全满足煤矿区地表单点在
$t = 0$ 和$t = + \infty $ 时的动态沉降、沉降速度和加速度理论极值特征。2）在AIC准则下，12种模型中，三参数的Hossfeld函数能够较好地兼顾强拟合性、高适应性和低复杂性3个优化选取关键指标。
3）当地质采矿未知时，可优选Hossfeld模型作为描述煤矿区地表单点动态沉降的时间函数；当地质采矿条件已知且上覆岩层坚硬时，优选Weibull模型，其他条件下可优选Hossfeld模型。
4）相较于三参数Hossfeld模型，四参数Slobada模型（四参数表现最优）在新增一个未知数的代价下拟合精度仅提高了5.3 mm，存在“过度参数化”现象。
-
![]()
图 1 矿区生态修复碳汇形成的关键过程
Figure 1. Key process of carbon sink formation in ecological restoration of mining area
![]()
图 2 黄土高原矿区生态修复适应性
Figure 2. Adaptability of ecological restoration in mining areas of the Loess Plateau
![]()
图 3 黄土高原典例矿区土壤-植物系统的恢复力
Figure 3. Recoverability of ecological restoration in typical mining areas of the Loess Plateau
![]()
图 4 黄土高原矿区不同恢复年限下表层土壤有机碳含量
Figure 4. Soil surface organic carbon content in the restoration times of mining areas in the Loess Plateau
![]()
图 5 黄土高原矿区土壤碳库稳定性机制
Figure 5. Mechanism of soil carbon pool stability in mining area of Loess Plateau
![]()
图 6 黄土高原矿区生态修复固碳增汇关键技术
Figure 6. Key technologies for carbon sequestration and sink enhancement in ecological restoration of mining areas on the Loess Plateau
表 1 评价因子相关信息
Table 1 Evaluation factor related information
数据 权重 来源 土壤有机碳含量 0.2967 联合国粮农组织
HWSD项目数据土壤容重 0.1379 联合国粮农组织
HWSD项目数据坡度（SRTMDEM 250M分辨率原始高程） 0.1806 地理空间数据云 中国年降水量空间插值数据（2010年） 0.2043 资源环境科学与
数据中心中国年度植被指数（NDVI）（2015年） 0.1805 资源环境科学与
数据中心
下载: 导出CSV
-
[1] 于昊辰,卞正富,陈 浮. 矿山土地生态动态恢复机制: 基于LDN框架的分析[J]. 中国土地科学,2020,34(9):86−95. YU Haochen,BIAN Zhengfu,CHEN Fu. Dynamic mechanism of land ecological restoration in mining area: based on Land Degradation Neutrality (LDN) framework[J]. China Land Science,2020,34(9):86−95.
[2] 朱 磊, 古文哲, 宋天奇, 等. 采空区煤矸石浆体充填技术研究进展与展望[J/OL]. 煤炭科学技术: 1-12[2022-12-29]. DOI: 10.13199/j.cnki.cst.2022-1725. ZHU Lei, GU Wenzhe, SONG Tianqi, et al. Research progress and prospect of coal gangue slurry filling technology in goaf[J/OL]. Coal Science and Technology, 1-12[2022-12-29]. DOI: 10.13199/j.cnki.cst.2022-1725.
[3] 李肖肖,骆占斌,马 静,等. 黄土高原矿区土壤细菌群落对地表塌陷和土地复垦的响应[J]. 环境化学,2020,39(5):1384−1394. doi: 10.7524/j.issn.0254-6108.2019090205 LI Xiaoxiao,LUO Zhanbin,MA Jing,et al. Response of coal mining bacterial community to surface subsidence and land reclamation in the Loess Plateau[J]. Environmental Chemistry,2020,39(5):1384−1394. doi: 10.7524/j.issn.0254-6108.2019090205
[4] 白中科,周 伟,王金满,等. 再论矿区生态系统恢复重建[J]. 中国土地科学,2018,32(11):1−9. doi: 10.11994/zgtdkx.20181107.162318 BAI Zhongke,ZHOU Wei,WANG Jinman,et al. Rethink on ecosystem restoration and rehabilitation of mining areas[J]. China Land Science,2018,32(11):1−9. doi: 10.11994/zgtdkx.20181107.162318
[5] 陈 浮, 赵 姣, 马 静, 等. 植被恢复对黄土高原露天矿区土壤碳氮磷功能微生物类群的影响[J/OL]. 土壤学报: 1-13[2022-12-29]. https://kns.cnki.net/kcms/detail/32.1119.P.20220729.1321.002.html. CHEN Fu, ZHAO Jiao, MA Jing, et al. Effects of vegetation restoration on functional groups related to soil carbon, nitrogen and phosphorus cycles in open-pit mining area of the loess plateau[J/OL]. Acta Pedologica Sinica, 1-13[2022-12-29]. http://kns.cnki.net/kcms/detail/32.1119.P.20220729.1321.002.html
[6] 陈 浮,王思遥,于昊辰,等. 碳中和目标下煤炭变革的技术路径[J]. 煤炭学报,2022,47(4):1452−1461. doi: 10.13225/j.cnki.jccs.2022.0192 CHEN Fu,WANG Siyao,YU Haochen,et al. Technological innovation paths of coal industry for achieving carbon neutralization[J]. Journal of China Coal Society,2022,47(4):1452−1461. doi: 10.13225/j.cnki.jccs.2022.0192
[7] 陈 浮,曾思燕,杨永均,等. 从乡村振兴视角引导新时代矿区生态修复[J]. 环境保护,2018,46(12):39−42. CHEN Fu,ZENG Siyan,YANG Yongjun,et al. Guiding on current ecological restoration in post-mining area from the perspective of rural revitalization[J]. Environmental Protection,2018,46(12):39−42.
[8] 李智兰. 矿区复垦对土壤养分和酶活性以及微生物数量的影响[J]. 水土保持通报,2015,35(2):6−13. LI Zhilan. Soil nutrients, enzyme activity and microbe quantity in reclaimed soil in mining area[J]. Bulletin of Soil and Water Conservation,2015,35(2):6−13.
[9] 胡振琪,张子璇,孙 煌. 试论矿山生态修复的地质成土[J]. 煤田地质与勘探,2022,50(12):21−29. doi: 10.12363/issn.1001-1986.22.06.0463 HU Zhenqi,ZHANG Zixuan,SUN Huang. Geological soil formation for ecological restoration of mining areas and its case study[J]. Coal Geology and Exploration,2022,50(12):21−29. doi: 10.12363/issn.1001-1986.22.06.0463
[10] 张 召,白中科,贺振伟,等. 基于RS与GIS的平朔露天矿区土地利用类型与碳汇量的动态变化[J]. 农业工程学报,2012,28(3):230−236. ZHANG Zhao,BAI Zhongke,HE Zhenwei,et al. Dynamic changes of land use type and carbon sinks based on RS and GIS in Pingshuo opencast coal mine[J]. Transactions of the Chinese Society of Agricultural Engineering,2012,28(3):230−236.
[11] 毕银丽,郭 芸,刘 峰,等. 西部煤矿区生物土壤结皮的生态修复作用及其碳中和贡献[J]. 煤炭学报,2022,47(8):2883−2895. doi: 10.13225/j.cnki.jccs.2022.0509 BI Yinli,GUO Yun,LIU Feng,et al. Ecological restoration effect and carbon neutrality contribution of biological soil crusts in western mining area[J]. Journal of China Coal Society,2022,47(8):2883−2895. doi: 10.13225/j.cnki.jccs.2022.0509
[12] 陈 浮,朱燕峰,马 静,等. 生物炭+速生植物对工业场地周边土壤镉污染原位修复[J]. 煤炭学报,2021,46(5):1477−1486. doi: 10.13225/j.cnki.jccs.st21.0183 CHEN Fu,ZHU Yanfeng,MA Jing,et al. In-situ remediation of Cd contaminated soil around industrial site by biochar combined with rank vegetation[J]. Journal of China Coal Society,2021,46(5):1477−1486. doi: 10.13225/j.cnki.jccs.st21.0183
[13] 张黎明,张绍良,侯湖平,等. 矿区土地复垦碳减排效果测度模型与实证分析[J]. 中国矿业,2015,24(11):65−70. ZHANG Liming,ZHANG Shoaling,HOU Huping,et al. Evaluation model and empirical study of carbon emission reduction effect from mining land reclamation[J]. China Mining Magazine,2015,24(11):65−70.
[14] 周广胜,周梦子,周 莉,等. 中国陆地生态系统增汇潜力研究展望[J]. 科学通报,2022,67(31):3625−3632. doi: 10.1360/TB-2022-0032 ZHOU Guangsheng,ZHOU Mengzi,ZHOU Li,et al. Advances in the carbon sink potential of terrestrial ecosystems in China[J]. Chinese Science Bulletin,2022,67(31):3625−3632. doi: 10.1360/TB-2022-0032
[15] 刘祥宏,阎永军,刘 伟,等. 碳中和战略下煤矿区生态碳汇体系构建及功能提升展望[J]. 环境科学,2022,43(4):2237−2250. LIU Xianghong,YAN Yongjun,LIU Wei,et al. System construction and the function improvement of ecological carbon sink in coal mining areas under the carbon neutral strategy[J]. Environmental Science,2022,43(4):2237−2250.
[16] WANG Biao,GONG Jirui,ZHANG Zihe,et al. Nitrogen addition alters photosynthetic carbon fixation, allocation of photoassimilates, and carbon partitioning of Leymus chinensis in a temperate grassland of Inner Mongolia[J]. Agricultural and Forest Meteorology,2019,279:107743. doi: 10.1016/j.agrformet.2019.107743
[17] 马 静,董文雪,朱燕峰,等. 东部平原矿区复垦对土壤微生物固碳潜力的影响[J]. 煤炭学报,2022,47(3):1306−1317. MA Jing,DONG Wenxue,ZHU Yanfeng,et al. Impact of land reclamation on the carbon sequestration potential of soil microorganisms in the disturbed mining area of eastern plain[J]. Journal of China Coal Society,2022,47(3):1306−1317.
[18] 黄 麟. 造林气候调节效应及其影响机理研究进展[J]. 生态学报,2021,41(2):469−478. HUANG Qi. A review on the climatic regulation effects of afforestation and its impact mechanisms[J]. Acta Ecologica Sinica,2021,41(2):469−478.
[19] ZHANG Qi,MA Jing,YANG Yongjun,et al. Mining subsidence-induced microtopographic effects alter the interaction of soil bacteria in the sandy pasture, China[J]. Frontiers in Environmental Science,2021,9:656708. doi: 10.3389/fenvs.2021.656708
[20] DE DEYN G B,CORNELISSEN J H C,BARDGETT R D. Plant functional traits and soil carbon sequestration in contrasting biomes[J]. Ecology Letters,2008,11(5):516−531. doi: 10.1111/j.1461-0248.2008.01164.x
[21] 刘亚龙, 王 萍, 汪景宽. 土壤团聚体的形成和稳定机制: 研究进展与展望 [J/OL]. 土壤学报: 1-18[2022-12-29]. https://kns.cnki.net/kcms/detail/32.1119.P.20220729.1322.004.html. LIU Yalong, WANG Ping, WANG Jingkuan. A review on the climatic regulation effects of afforestation and its impact mechanisms[J]. Acta Pedologica Sinica, 1-18[2022-12-29]. https://kns.cnki.net/kcms/detail/32.1119.P.20220729.1322.004.html.
[22] 赵 姣, 马 静, 朱燕峰, 等. 植被类型对黄土高原露采矿山复垦土壤碳循环功能基因的影响[J/OL]. 环境科学: 1-12[2022-12-29]. DOI: 10.13227/j.hjkx.202206219. ZHAO Jiao, MA Jing, ZHU Yanfeng, et al. Effects of Vegetation Types on Carbon Cycle Functional Genes in Reclaimed Soil from Open Pit Mines in the Loess Plateau[J]. Environmental Science, 1-12[2022-12-29]. DOI: 10.13227/j.hjkx.202206219.
[23] HONG Songbai,YIN Guodong,PIAO Shilong,et al. Divergent responses of soil organic carbon to afforestation[J]. Nature Sustainability,2020,3(9):694−700. doi: 10.1038/s41893-020-0557-y
[24] ZHU Yanfeng,GE Xiaoping,WANG Liping,et al. Biochar rebuilds the network complexity of rare and abundant microbial taxa in reclaimed soil of mining areas to cooperatively avert cadmium stress[J]. Frontiers in Microbiology,2022,13:972300. doi: 10.3389/fmicb.2022.972300
[25] CAI Yuanfeng,ZHENG Yan,BODELIER Paul L E,et al. Conventional methanotrophs are responsible for atmospheric methane oxidation in paddy soils[J]. Nature Communications,2016,7(1):11728. doi: 10.1038/ncomms11728
[26] CHAO Liang,JOSHU P Schimel,JULIE D Jastrow,et al. The importance of anabolism in microbial control over soil carbon storage[J]. Nature Microbiology,2017,2:17105. doi: 10.1038/nmicrobiol.2017.105
[27] JING Shi,BAILEY Vanessa,DORHEIM Kalyn,et al. Historically inconsistent productivity and respiration fluxes in the global terrestrial carbon cycle[J]. Nature Communications,2022,13(1):1733. doi: 10.1038/s41467-022-29391-5
[28] SHARMA Richa,PRADHAN Lolita,KUMARI Maya,et al. Assessment of carbon sequestration potential of tree species in amity university campus noida[J]. Environmental Sciences Proceedings,2021,3(1):52.
[29] LUDěK Bujalský,SATOSHI Kaneda,PETR Dvorščíka,et al. In situ soil respiration at reclaimed and unreclaimed post-mining sites: Responses to temperature and reclamation treatment[J]. Ecological Engineering,2014,68:53−59. doi: 10.1016/j.ecoleng.2014.03.048
[30] 谢苗苗,刘金莹,陈 彬,等. 生态脆弱区复垦排土场干扰类型划分及影响因素分析[J]. 煤炭科学技术,2022,50(2):280−288. XIE Miaomiao,LIU Jinying,CHEN Bin,et al. Classification of disturbance types and influencing factors of reclamation dump in ecological fragile area[J]. Coal Science and Technology,2022,50(2):280−288.
[31] 王同智,薛 焱,包玉英,等. 不同复垦方式对黑岱沟露天矿排土场土壤有机碳的影响[J]. 安全与环境学报,2014,14(2):174−178. WANG Tongzhi,XUE Yan,BAO Yuying,et al. Soil organic carbon content of the spoiled bank under different reclamation modes, Heidaigou open pit, Inner Mongolia[J]. Journal of Safety and Environment,2014,14(2):174−178.
[32] 史利江,高 杉,姚晓军,等. 晋西北黄土丘陵区不同植被恢复下的土壤碳氮累积特征[J]. 生态环境学报,2021,30(9):1787−1796. SHI Lijiang,GAO Bin,YAO Xiaojun,et al. Characteristics of soil carbon and nitrogen accumulation under different vegetation restoration in the loess hilly region of northwest shanxi province[J]. Ecology and Environmental Sciences,2021,30(9):1787−1796.
[33] 张江周,李奕赞,李 颖,等. 土壤健康指标体系与评价方法研究进展[J]. 土壤学报,2022,59(3):603−616. ZHANG Jiangzhou,LI Yizan,LI Ying,et al. Advances in the indicator system and evaluation approaches of soil health[J]. Acta Pedologica Sinica,2022,59(3):603−616.
[34] SHRESTHA R K,LAL R. Ecosystem carbon budgeting and soil carbon sequestration in reclaimed mine soil[J]. Environment International,2006,32(6):781−796. doi: 10.1016/j.envint.2006.05.001
[35] 李俊超,党廷辉,薛 江,等. 植被重建下露天煤矿排土场边坡土壤碳储量变化[J]. 土壤学报,2015,52(2):453−460. doi: 10.11766/trxb201402200074 LI Junchao,DANG Yanhui,XUE Jiang,et al. Variability of soil organic carbon storage in dump slope of opencast coal mine under revegetation[J]. Acta Pedologica Sinica,2015,52(2):453−460. doi: 10.11766/trxb201402200074
[36] 马 静,董文雪,朱燕峰,等. 东部平原矿区复垦土壤微生物群落特征及其组装过程[J]. 环境科学,2022,43(7):3844−3853. MA Jing,DONG Wenxue,ZHU Yanfeng,et al. Characteristics and assembly process of reclaimed soil microbial communities in eastern plain mining areas[J]. Environmental Science,2022,43(7):3844−3853.
[37] POONAM Panchal,CATHERINE Preece,JOSEP Peñuelas,et al. Soil carbon sequestration by root exudates[J]. Trends in Plant Science,2022,27(8):749−757. doi: 10.1016/j.tplants.2022.04.009
[38] CHEN Chunmei, HALL S J, COWARD E. Hall, et al. Iron-mediated organic matter decomposition in humid soils can counteract protection[J]. Nature communications, 2020, 11(1): 2255.
[39] TORIYAMA Jumpei,HAK Mao,IMAYA Akihiro,et al. Effects of forest type and environmental factors on the soil organic carbon pool and its density fractions in a seasonally dry tropical forest[J]. Forest Ecology and Management,2015,335:147−155. doi: 10.1016/j.foreco.2014.09.037
[40] IMANSK Vladimir,DANIEL Bajan. Stability of soil aggregates and their ability of carbon sequestration[J]. Soil and Water Research,2014,9(3):111−118. doi: 10.17221/106/2013-SWR
[41] 刘满强,胡 锋,陈小云. 土壤有机碳稳定机制研究进展[J]. 生态学报,2007,27(6):2642−2650. doi: 10.3321/j.issn:1000-0933.2007.06.059 LIU Manqiang,HU Feng,CHEN Xiaoyun. A review on mechanisms of soil organic carbon stabilization[J]. Acta Ecologica Sinica,2007,27(6):2642−2650. doi: 10.3321/j.issn:1000-0933.2007.06.059
[42] CARTER Martin R. Soil quality for sustainable land management[J]. Agronomy Journal, 2002, 94(1): 3800.
[43] 李如剑,张彦军,赵 慢,等. 坡度和降雨影响土壤CO2通量和有机碳流失的模拟研究[J]. 环境科学学报,2016,36(4):1336−1342. LI Rujian,ZHANG Yanjun,ZHAO Man,et al. Simulation on the effects of slope and rainfall on soil CO2 flux and SOC loss[J]. Acta Scientiae Circumstantiae,2016,36(4):1336−1342.
[44] 胡振琪. 矿山复垦土壤重构的理论与方法[J]. 煤炭学报,2022,47(7):2499−2515. doi: 10.13225/j.cnki.jccs.yg22.0696 HU Zhenqi. Theory and method of soil reconstruction of reclaimed mined land[J]. Journal of China Coal Society,2022,47(7):2499−2515. doi: 10.13225/j.cnki.jccs.yg22.0696
[45] LI Yuan,ZU Yanqun,FANG Qixian,et al. Characteristics of heavy-metal tolerance and growth in two ecotypes of oxyria sinensisHemsl. grown on Huize Lead–Zinc Mining Area in Yunnan Province, China[J]. Communications in Soil Science and Plant Analysis,2013,44(16):2428−2442. doi: 10.1080/00103624.2013.803559
[46] MORGAN J A,BENDING G D,WHITE P J. Biological costs and benefits to plant–microbe interactions in the rhizosphere[J]. Journal of Experimental Botany,2005,56(417):1729−1739. doi: 10.1093/jxb/eri205
[47] Thavamani Palanisami,Samkumar R A,Satheesh V,et al. Microbes from mined sites: Harnessing their potential for reclamation of derelict mine sites[J]. Environmental Pollution,2017,230:495−505. doi: 10.1016/j.envpol.2017.06.056
[48] 陶雨萱, 郭 亮, 高 聪, 等. 代谢工程改造微生物固定二氧化碳研究进展[J]. 化工进展: 1-16[2022-12-29]. DOI: 10.16085/j.issn.1000-6613.2022-1545. LU Yuxuan, GUO Liang, GAO Cong, et al. Progress in metabolic engineering of microorganisms for CO2 fixation[J]. Chemical Industry and Engineering Progress, 1-16 [2022-12-29]. DOI: 10.16085/j.issn.1000-6613.2022-1545.
[49] 李树志,李学良,尹大伟. 碳中和背景下煤炭矿山生态修复的几个基本问题[J]. 煤炭科学技术,2022,50(1):286−292. LI Shuzhi,LI Xueliang,YIN Dawei. Several basic issues of ecological restoration of coal mines under background of carbon neutrality[J]. Coal Science and Technology,2022,50(1):286−292.
[50] 黄雨晗,曹银贵,周 伟,等. 秸秆生物炭对草原矿区重构土苜蓿生长状况的影响[J]. 生态学报,2021,41(2):588−602. HUANG Yuhan,CAO Yingui,ZHOU Wei,et al. Effects of straw biochar on the growth of Medicago falcata in the reconstructed soil of grassland mining area[J]. Acta Ecologica Sinica,2021,41(2):588−602.
-
期刊类型引用(1)
1. 胡海洋，阳富芹，陈捷，娄毅，万玉亭. 贵州省盘关向斜煤层气储层地质特征及开发效果评价. 石油实验地质. 2025(01): 213-222 . 
百度学术
其他类型引用(0)
计量
- 文章访问数: 214
- HTML全文浏览量: 38
- PDF下载量: 150
- 被引次数: 1
