Interaction study on surrounding rock and bolting-shotcrete U-shaped steel support structure in deep soft rock roadway
-
摘要:
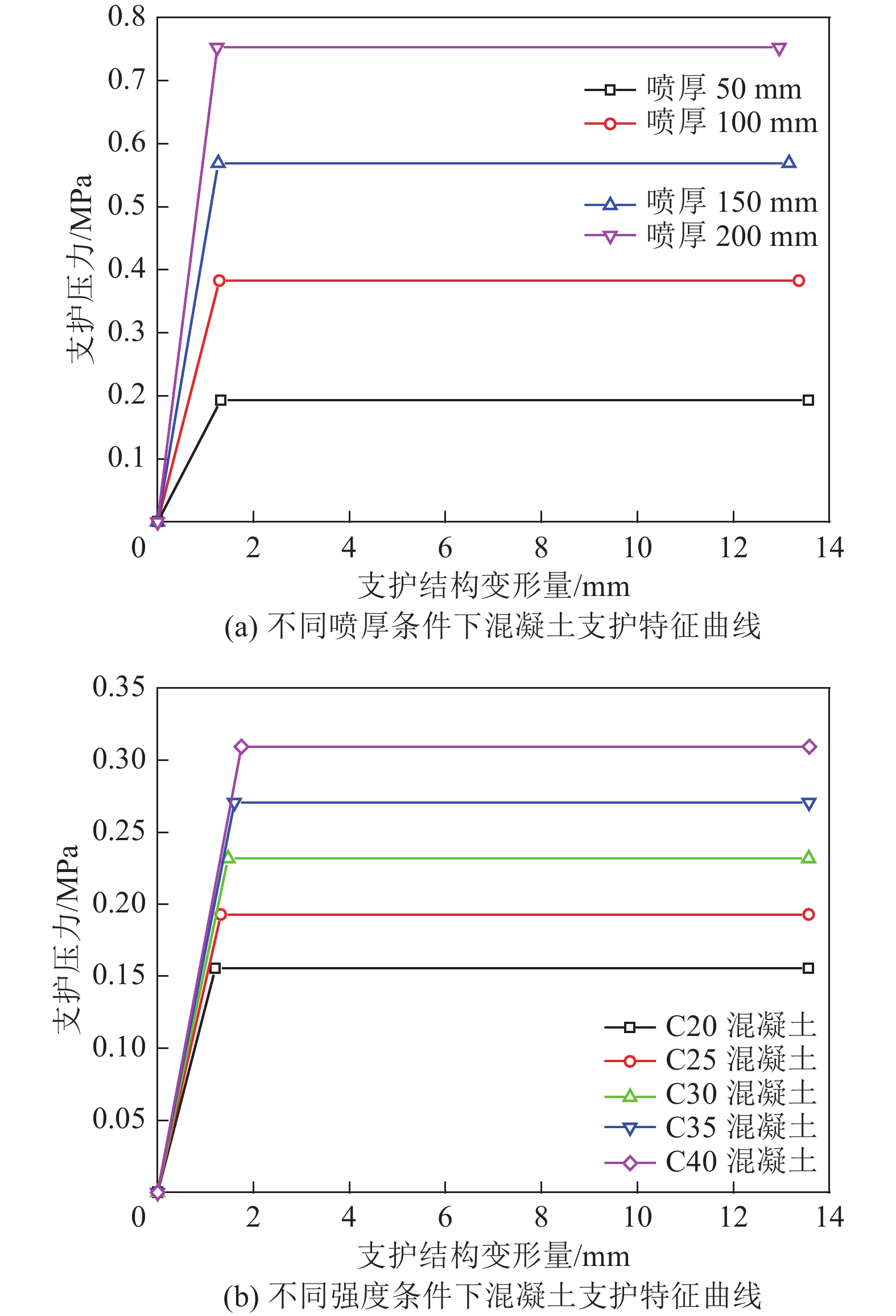
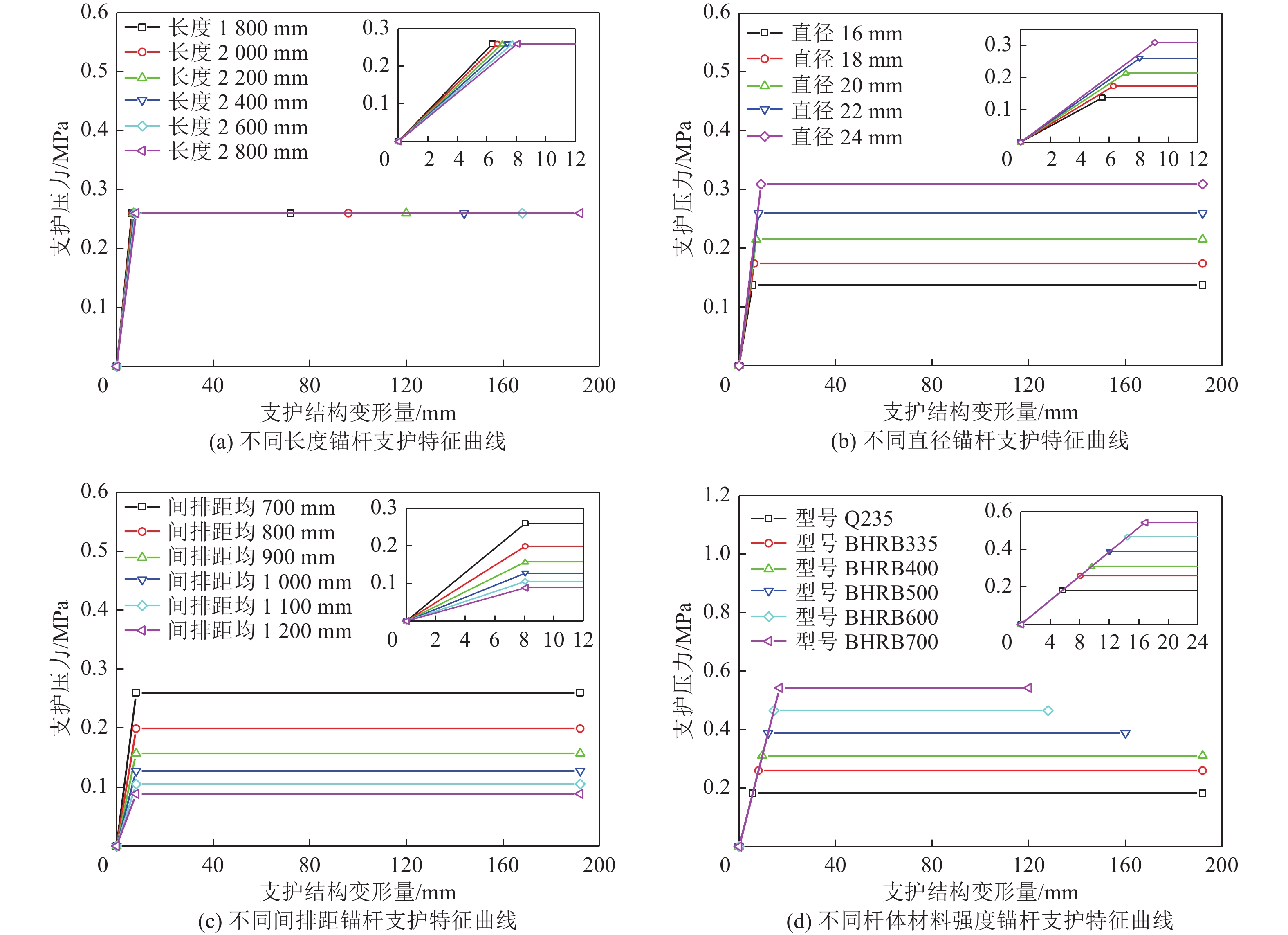
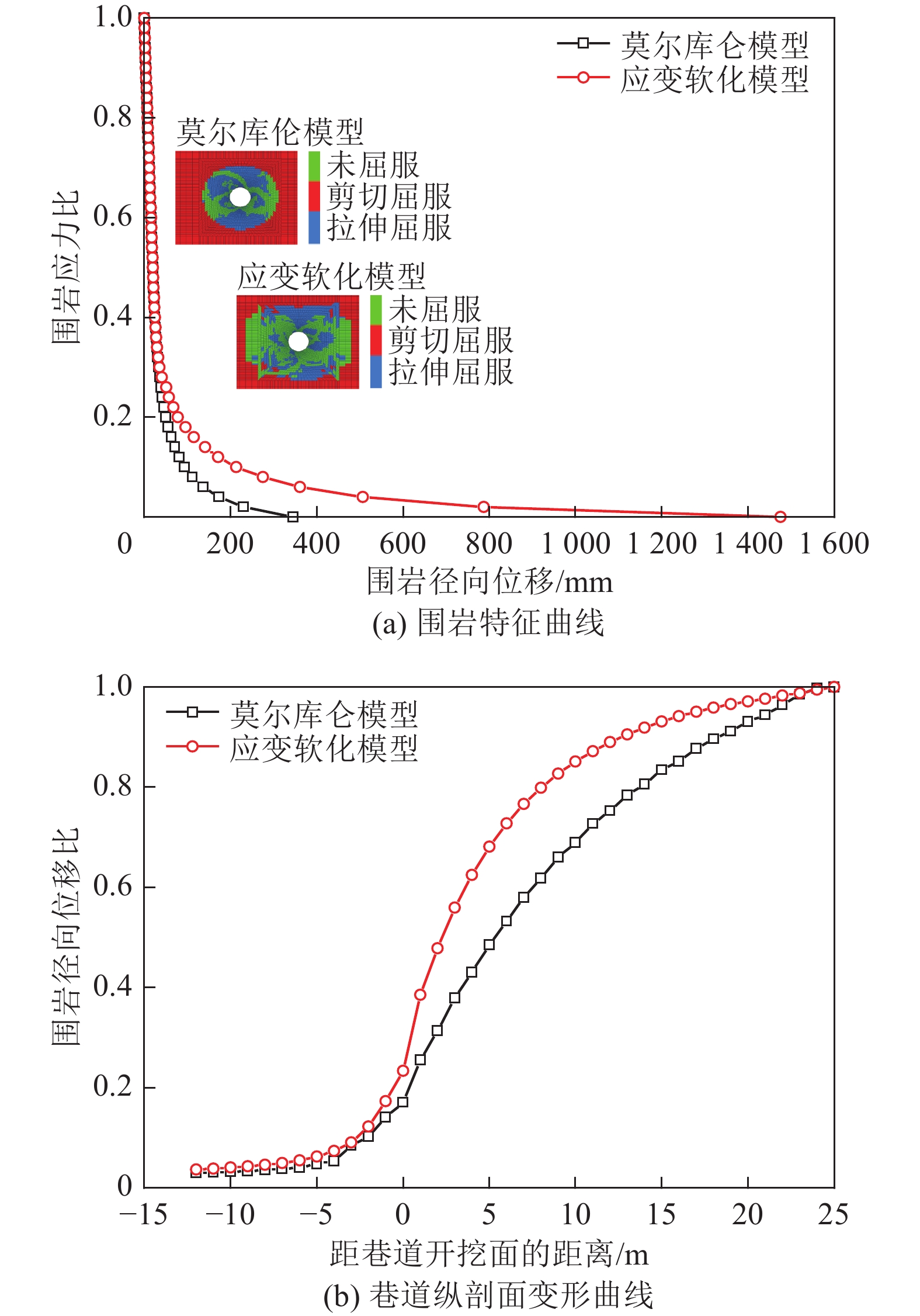
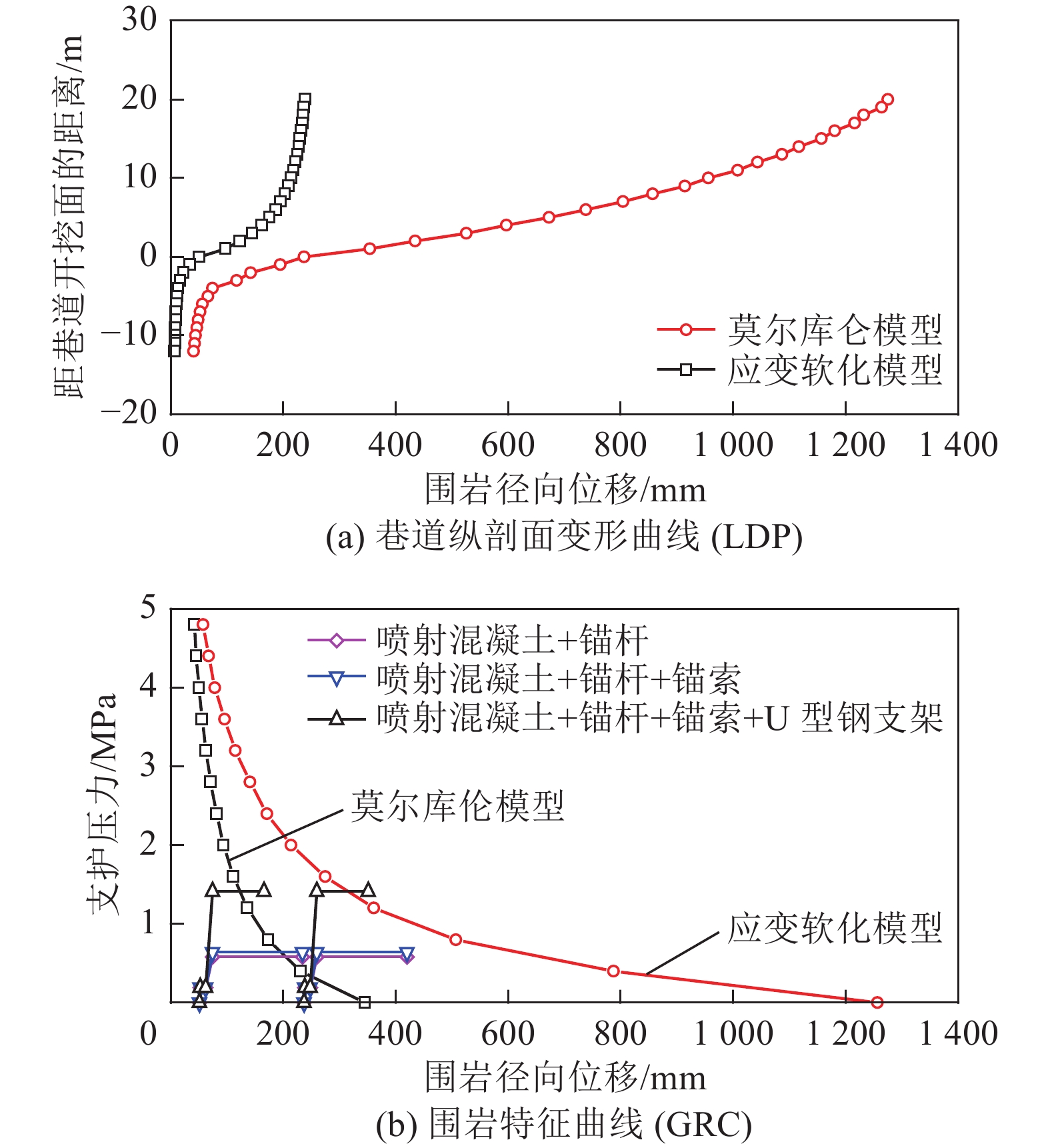
收敛−约束法(特性曲线法)是将理论解析、现场实测、工程经验相结合的一种地下工程结构设计方法，是目前分析围岩−支护相互作用关系及开展支护优化设计的常用方法。基于收敛−约束法的基本原理，总结给出了经典的支护特征与支护结构变形方程，理论计算获得了喷射混凝土、锚杆(索)、U型钢支架等支护结构的支护特征曲线，分析了支护结构的几何尺寸(直径、长度)、间排距、材料强度等参数对支护压力的影响特征；随着喷射混凝土厚度及强度等级的增加，喷射混凝土提供的支护刚度和支护压力逐渐增大；随着锚杆(索)直径、长度、杆体材料强度的增加及间排距的减小，锚杆的支护压力显著增加；U型钢支架的排距越小及材料强度越大，其提供的支护压力越大。采用FLAC3D内嵌的莫尔库伦应变软化本构模型，建立了考虑岩石峰后应变软化与扩容特性的深部软岩巷道数值分析模型，计算获得了不同应力状态下巷道纵剖面变形曲线、围岩特征曲线，分析了锚喷、锚杆(索)喷、锚喷U型钢等3种联合支护技术对深部巷道围岩大变形控制的适用性，验证了锚喷U型钢联合支护技术应用于深部巷道支护工程的可行性。考虑岩石峰后应变软化与扩容特性的应变软化本构模型和经典的莫尔库伦本构模型的数值模拟结果相差较大，采用莫尔库伦本构模型的数值模拟结果保守，支护结构提供的支护压力无法满足深部巷道稳定性控制要求，支护后巷道围岩变形较大甚至会发生冒顶、片帮等安全事故。
Abstract:Convergence-constraint method is a design approach for underground engineering structures that combines theoretical analysis, field measurements, and engineering experience. It is a commonly used method for analyzing the interaction between surrounding rock and support system, as well as for conducting optimized support design. Based on the basic principle of convergence-constraint method, the classical supporting characteristics and supporting structure deformation equations were summarized. The supporting characteristic curves of supporting structures such as shotcrete, bolt (cable) and U-shaped steel were obtained by theoretical calculation. The effects of the geometric size (diameter, length), row spacing and material strength of supporting structure on the support pressure were analyzed. As the thickness and strength grade of shotcrete increase, the support stiffness and support pressure provided by shotcrete gradually escalate. Simultaneously, the support pressure of bolts experiences a significant increase with the augmentation of bolt (cable) diameter, length, material strength, and reduction in row-spacing between them. Furthermore, the support pressure delivered by U-shaped supports intensifies as the row-spacing decreases and the material strength increases. By using the Mohr-Coulomb strain softening constitutive model embedded in FLAC3D, the numerical analysis model of deep soft rock roadway considering the post-peak strain softening and dilatancy characteristics of rock was established. The deformation curves of roadway longitudinal section and the characteristic curves of surrounding rock under different stress states were calculated. The applicability of three kinds of combined support technologies such as bolting shotcrete, bolt (cable) shotcrete and bolting-shotcrete U-shaped steel to the large deformation control of surrounding rock in deep roadway were analyzed. The feasibility of applying the combined support technique of bolting-shotcrete U-shaped steel in deep roadway support projects has been verified. The numerical simulation results of the strain-softening constitutive model considering the post-peak strain-softening and dilatancy characteristics of rocks are quite different from those based on the classical Mohr-Coulomb constitutive model. The numerical simulation results of the Mohr-Coulomb constitutive model are conservative, and the supporting pressure provided by the supporting structure cannot meet the stability control requirements of the deep roadway. After roadway support, the surrounding rock exhibits pronounced deformation, which may give rise to safety incidents such as roof collapse and wall instability.
-
-
![]()
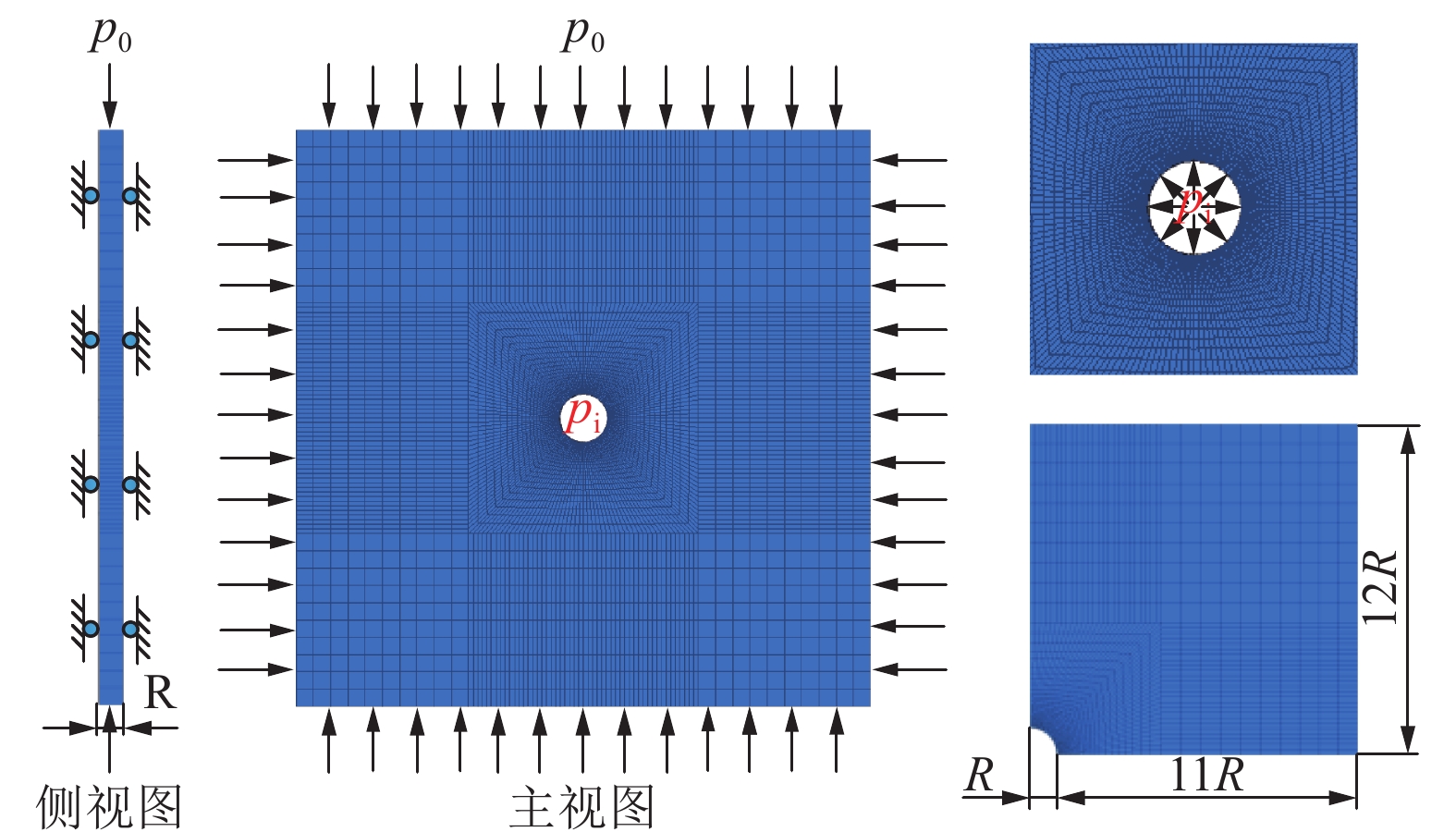
图 1 收敛−约束法基本原理示意(基于文献[28]修改)
Figure 1. Schematic diagram of convergence confinement method
![]()
图 6 巷道纵剖面变形曲线求解数值计算模型
Figure 6. Numerical calculation model for deformation curve of longitudinal section of roadway
![]()
图 8 巷道围岩特征曲线求解数值计算模型
Figure 8. Numerical calculation model of surrounding rock characteristic curve of roadway
![]()
图 12 深部巷道围岩−支护相互作用关系
Figure 12. Interaction between deep roadway surrounding rock and support
表 1 深部巷道经典的支护特征与支护结构变形方程[23-24,29]
Table 1 Classical supporting characteristics and deformation equation of supporting structure of deep roadway[23-24,29]
支护结构 支护特征方程及物理含义 支护结构变形方程及物理含义 混凝土 $ \left\{ \begin{gathered} {K_{{\mathrm{shot}}}} = \frac{{{E_{{\mathrm{con}}}}}}{{\left( {1 + {\mu _{{\mathrm{con}}}}} \right)}}\frac{{\left[ {{R^2} - {{\left( {R - {t_{{\mathrm{shot}}}}} \right)}^2}} \right]}}{{\left( {1 - 2{\mu _{{\mathrm{con}}}}} \right){R^2} + {{\left( {R - {t_{{\mathrm{shot}}}}} \right)}^2}}}\frac{1}{R} \\ {P_{{\mathrm{max, shot}}}} = \frac{1}{2}{\sigma _{\mathrm{c}}}\left[ {1 - \frac{{{{\left( {R - {t_{{\mathrm{shot}}}}} \right)}^2}}}{{{R^2}}}} \right] \\ \end{gathered} \right. $ $ \left\{ \begin{gathered} {u_{{\mathrm{in, shot}}}} = \frac{{{P_{{\mathrm{max, shot}}}}}}{{{K_{{\mathrm{shot}}}}}} \\ {u_{{\mathrm{max, shot}}}} = {u_{{\mathrm{in, shot}}}} + {\varepsilon _{{\mathrm{br, con}}}}\left( {R - {t_{{\mathrm{shot}}}}} \right)- \\ \frac{{2\left( {1 - {v_{{\mathrm{con}}}}} \right)}}{{{{\left( {R - {t_{{\mathrm{shot}}}}} \right)}^2} + \left( {1 - 2{v_{{\mathrm{con}}}}} \right){R^2}}} \cdot \frac{{{P_{{\mathrm{max, shot}}}}}}{{{K_{{\mathrm{shot}}}}}} \\ \end{gathered} \right. $ 式中：Kshot为混凝土的支护刚度，MPa/m；Pmax, shot为混凝土的最大承载力，MPa；
tshot为混凝土的厚度，mm；Econ为混凝土的弹性模量，GPa；μcon为混凝土的
泊松比；R为等效圆形巷道开挖半径，m式中：uin, shot为混凝土的弹性变形量，mm；umax, shot为混凝土的最大变形量，mm；εbr, con为混凝土的破坏应变，mm/mm 锚杆(索) $\left\{ \begin{gathered} {K_{{\mathrm{bol}}}} = \frac{1}{{{S_{\mathrm{c}}}{S_{\mathrm{l}}}\left[ {\frac{{4{L_{{\mathrm{bol}}}}}}{{\pi {\varphi ^2}{E_{{\mathrm{bol}}}}}} + Q} \right]}} \\ {P_{{\mathrm{max, bol}}}} = \frac{{{T_{\max }}}}{{{S_{\mathrm{c}}}{S_{\mathrm{l}}}}} \\ \end{gathered} \right.$ $ \left\{ \begin{gathered} {u_{{\mathrm{in, bol}}}} = \frac{{{P_{{\mathrm{max, bol}}}}}}{{{K_{{\mathrm{bol}}}}}} \\ {u_{{\mathrm{max, bol}}}} = {u_{{\mathrm{in, bol}}}} + {\varepsilon _{{\mathrm{br, st}}}}{L_{{\mathrm{bol}}}} \\ \end{gathered} \right. $ 式中：Kbol为锚杆(索)的支护刚度，MPa/m；Pmax, bol为锚杆(索)的最大承载力，MPa；
Ebol为锚杆(索)材料的弹性模量，MPa；Sc、Sl依次为锚杆(索)的间距和排距，mm；
Lbol为锚杆(索)的自由段长度，m；φ为锚杆(索)的直径，mm；Tmax为锚杆(索)的
抗拉拔力，若不考虑锚杆(索)被拔出情况可为杆(索)体的抗拉强度，kN；
Q为与锚杆(索)体、托盘等变形特征相关的常数式中：uin, bol为锚杆(索)的弹性变形量，mm；umax, bol为锚杆(索)的最大变形量，mm；
εbr, st为锚杆(索)破坏应变，mm/mmU型钢 $ \left\{ \begin{gathered} {K_{{\mathrm{set}}}} = \frac{{{E_{{\mathrm{set}}}}{A_{{\mathrm{set}}}}}}{{d{{\left( {R - {{{h_{{\mathrm{set}}}}} \mathord{\left/ {\vphantom {{{h_{{\mathrm{set}}}}} 2}} \right. } 2}} \right)}^2}}} \\ {P_{{\mathrm{max, bol}}}} = \frac{{{\sigma _{{\mathrm{set}}}}{A_{{\mathrm{set}}}}}}{{d\left( {R - {{{h_{{\mathrm{set}}}}} \mathord{\left/ {\vphantom {{{h_{{\mathrm{set}}}}} 2}} \right. } 2}} \right)}} \\ \end{gathered} \right. $ $ \left\{ \begin{gathered} {u_{{\mathrm{in, set}}}} = \frac{{{P_{{\mathrm{max, set}}}}}}{{{K_{{\mathrm{set}}}}}} \\ {u_{{\mathrm{max, set}}}} = {u_{{\mathrm{in, set}}}} + {\varepsilon _{{\mathrm{br, st}}}}\left( {R - \frac{{{h_{{\mathrm{set}}}}}}{2}} \right) \\ \end{gathered} \right. $ 式中：Kset为钢拱架的支护刚度，MPa/m；Pmax, set为钢拱架的最大承载力，MPa；
Eset为钢拱架的弹性模量，MPa；d为钢拱架的排距(棚距)，m；Aset为钢拱架的
横截面面积，mm2；σset为钢拱架材料的屈服强度，MPa；hset为钢拱架的
横截面高度，m式中：uin, set为钢拱架的弹性变形量，mm；umax, set为钢拱架的最大变形量，mm；εbr, st为钢拱架的破坏应变，mm/mm 
下载: 导出CSV
表 2 σ3=20 MPa条件下岩石峰后强度参数与剪胀角数值模拟取值[30]
Table 2 Numerical simulation values of post-peak strength parameters and dilatancy angle of rock under condition of σ3=20 MPa[30]
γP/(mm·mm−1) 0 0.002 3 0.004 6 0.006 9 0.009 2 0.011 5 0.013 8 0.016 1 0.018 4 0.020 7 0.023 0 εps/(mm·mm−1) 0.002 9 0.004 2 0.005 5 0.006 2 0.007 3 0.008 9 0.010 6 0.012 0 0.013 2 0.013 7 0.014 0 c´/MPa 25.77 24.63 23.59 22.61 19.57 16.81 13.89 12.06 11.14 10.05 9.37 φ´/（°） 25.59 26.90 27.23 28.36 28.06 27.86 26.83 25.20 22.36 20.26 18.99 ψ/（°） 36.13 39.59 39.37 37.19 34.08 30.62 27.15 23.88 20.85 18.14 15.73
下载: 导出CSV
表 3 支护构件的特征参数
Table 3 Characteristic parameter of supporting parts
序号 支护形式 pmax K uel umax 1 混凝土 0.19 146.40 1.32 13.57 2 锚杆 0.39 32.27 12.02 160.00 3 锚索 0.30 5.33 55.75 301.00 4 U型支架 0.76 153.22 4.99 19.94 注：Pmax为支护结构的最大支护压力，MPa；K为支护结构的刚度，MPa/m；uel为支护结构的弹性变形量，mm；umax为支护结构的最大变形量，mm。
下载: 导出CSV
表 4 联合支护结构的特征参数
Table 4 Characteristic parameter of combined support structure
支护组合 pmax, com Kcom uel, com umax, com 锚喷 0.39 32.27 12.02 160.00 锚杆(索)喷 0.45 37.60 12.02 160.00 锚喷U型钢 1.41 190.82 12.02 91.56 注：pmax, com为联合支护结构的最大支护压力，MPa；Kcom为联合支护结构的刚度，MPa/m；uel, com为联合支护结构的弹性变形量，mm；umax, com为联合支护结构的最大变形量，mm。
下载: 导出CSV
-
[1] 谢和平,李存宝,高明忠,等. 深部原位岩石力学构想与初步探索[J]. 岩石力学与工程学报,2021,40(2):217−232. XIE Heping,LI Cunbao,GAO Mingzhong,et al. Conceptualization and preliminary research on deep in situ rock mechanics[J]. Chinese Journal of Rock Mechanics and Engineering,2021,40(2):217−232.
[2] 何满潮. 深部建井力学研究进展[J]. 煤炭学报,2021,46(3):726−746. HE Manchao. Research progress of deep shaft construction mechanics[J]. Journal of China Coal Society,2021,46(3):726−746.
[3] 孙晓明,张勇,何满潮,等. 深部井巷工程高预应力NPR耦合支护技术[J]. 矿业科学学报,2023,8(1):50−65. SUN Xiaoming,ZHANG Yong,HE Manchao,et al. Research of high pre-stress NPR support technology in deep shaft roadway engineering[J]. Journal of Mining Science and Technology,2023,8(1):50−65.
[4] 刘泉声,卢兴利. 煤矿深部巷道破裂围岩非线性大变形及支护对策研究[J]. 岩土力学,2010,31(10):3273−3279. doi: 10.3969/j.issn.1000-7598.2010.10.039 LIU Quansheng,LU Xingli. Research on nonlinear large deformation and support measures for broken surrounding rocks of deep coal mine roadway[J]. Rock and Soil Mechanics,2010,31(10):3273−3279. doi: 10.3969/j.issn.1000-7598.2010.10.039
[5] 康永水,耿志,刘泉声,等. 我国软岩大变形灾害控制技术与方法研究进展[J]. 岩土力学,2022,43(8):2034−2059. KANG Yongshui,GENG Zhi,LIU Quansheng,et al. Research progress on support technology and methods for soft rock with large deformation hazards in China[J]. Rock and Soil Mechanics,2022,43(8):2034−2059.
[6] JIANG B,XIN Z X,ZHANG X F,et al. Mechanical properties and influence mechanism of confined concrete arches in high-stress tunnels[J]. International Journal of Mining Science and Technology,2023,33(7):829−841. doi: 10.1016/j.ijmst.2023.03.008
[7] 靖洪文,孟庆彬,朱俊福,等. 深部巷道围岩松动圈稳定控制理论与技术进展[J]. 采矿与安全工程学报,2020,37(3):429−442. JING Hongwen,MENG Qingbin,ZHU Junfu,et al. Theoretical and technical progress of stability control of broken rock zone of deep roadway surrounding rock[J]. Journal of Mining & Safety Engineering,2020,37(3):429−442.
[8] 王琦,何满潮,许硕,等. 恒阻吸能锚杆力学特性与工程应[J]. 煤炭学报,2022,47(4):1490−1500. WANG Qi,HE Manchao,XU Shuo,et al. Mechanical properties and engineering application of caonstant resistance energy absorbing bolt[J]. Journal of China Coal Society,2022,47( 4):1490−1500.
[9] 王卫军,范磊,马谕杰,等. 基于蝶形破坏理论的深部巷道围岩控制技术研究[J]. 煤炭科学技术,2023,51(1):157−167. WANG Weijun,FAN Lei,MA Yujie,et al. Research on surrounding rock control technology of deep roadway based on butterfly failure theory[J]. Coal Science and Technology,2023,51(1):157−167.
[10] 侯朝炯,王襄禹,柏建彪,等. 深部巷道围岩稳定性控制的基本理论与技术研究[J]. 中国矿业大学学报,2021,50(1):1−12. HOU Chaojiong,WANG Xiangyu,BAI Jianbiao,et al. Basic throry technology study of stability control for surrounding rock in deep roadway[J]. Journal of China University of Mining & Technology,2021,50(1):1−12.
[11] 左建平,洪紫杰,于美鲁,等. 破碎围岩梯度支护模型及分级控制研究[J]. 中国矿业大学学报,2022,51(2):221−231. doi: 10.3969/j.issn.1000-1964.2022.2.zgkydxxb202202002 ZUO Jianping,HONG Zijie,YU Meilu,et al. Research on gradient support mode and classification control of broken surrounding rock[J]. Journal of China Univerity of Mining & Technology,2022,51(2):221−231. doi: 10.3969/j.issn.1000-1964.2022.2.zgkydxxb202202002
[12] 康红普,姜鹏飞,黄炳香,等. 煤矿千米深井巷道围岩支护-改性-卸压协同控制技术[J]. 煤炭学报,2020,45(3):845−864. KANG Hongpu,JIANG Pengfei,HUANG Bingxiang,et al. Roadway strata control technology by means of bolting-modification-destressing in synergy in 1000 m deep coal mines[J]. Journal of China Coal Society,2020,45(3):845−864.
[13] 袁亮,薛俊华,刘泉声,等. 煤矿深部岩巷围岩控制理论与支护技术[J]. 煤炭学报,2011,36(4):535−543. YUAN Liang,XUE Junhua,LIU Quansheng,et al. Surrounding rock stability control theory and support technique in deep rock roadway for coal mine[J]. Journal of China Coal Society,2011,36(4):535−543.
[14] 程敬义,魏泽捷,白纪成,等. 基于爆破卸压的深部构造应力富水软岩巷道底鼓控制技术研究[J]. 煤炭科学技术,2022,50(7):117−126. CHENG Jingyi, WEI Zejie, BAI Jicheng,et al. Study on floor heave control technology of deep tectonic stress water-rich soft rock roadway based on blasting pressure relief[J]. Coal Science and Technology,2022,50(7):117−126.
[15] 刘学生,武允昊,谭云亮,等. 深部高水平应力巷道倾斜锚杆破断机制及加强支护时机[J]. 煤炭学报,2023,48(2):609−622. LIU Xuesheng,WU Yunhao,TAN Yunliang,et al. Breaking mechanism of inclined bolts in deep mine roadway with high horizontal stress and the timing of strengthening support[J]. Journal of China Coal Society,2023,48(2):609−622.
[16] 黄炳香,张农,靖洪文,等. 深井采动巷道围岩流变和结构失稳大变形理论[J]. 煤炭学报,2020,45(3):911−926. HUANG Bingxiang,ZHANG Nong,JING Hongwen,et al. Large deformation theory of rheology and structural instability of the surrounding rock in deep mining roadway[J]. Journal of China Coal Society,2020,45(3):911−926.
[17] 李英明,赵呈星,刘增辉,等. 岩承载层分层演化规律及“层-双拱”承载结构强度分析[J]. 岩石力学与工程学报,2020,39(2):217−227. LI Yingming,ZHAO Chengxing,LIU Zenghui,et al. Research on layered evolution law of surrounding rock bearing layers and strength analysis of “layer-double arch” bearing structure[J]. Chinese Journal of Rock Mechanics and Engineering,2020,39(2):217−227.
[18] 于凤海,赵同彬,胡善超,等. 大松动圈围岩锚网索联合支护参数确定方法探讨[J]. 岩土力学,2016,37(7):2021−2027. YU Fenghai,ZHAO Tongbin,HU Shanchao,et al. Discussion on determination method of anchor-net-cable coupling support parameters of surrounding rock in a large broken zone[J]. Rock and Soil Mechanics,2016,37(7):2021−2027.
[19] 侯公羽,梁金平,李小瑞. 常规条件下巷道支护设计的原理与方法研究[J]. 岩石力学与工程学报,2022,41(4):691−711. HOU Gongyu,LIANG Jinping,LI Xiaorui. Research on principles and methods of roadway support design under conventional conditions[J]. Chinese Journal of Rock Mechanics and Engineering,2022,41(4):691−711.
[20] ORESTE P P. Analysis of structural interaction in tunnels using the convergence-confinement approach[J]. Tunnelling and Underground Space Technology,2003,13(2):347−363.
[21] ALEJANO L R,ALONSO E. Application of the convergence-confinement method to tunnels in rock masses exhibiting Hoek-Brown strain-softening behaviour[J]. International Journal of Rock Mechanics and Mining Sciences,2010,12(6):150−160.
[22] VLACHOPOULOS N,DIEDERICHS M. Improved longitudinal displacement profiles for convergence confinement analysis of deep tunnels[J]. Rock Mechanics and Rock Engineering,2009,42(2):131−146. doi: 10.1007/s00603-009-0176-4
[23] 孙闯,张向东,张建俊. 深部断层破碎带竖井围岩与支护系统稳定性分析[J]. 煤炭学报,2013,38(4):587−594. SUN Chuang,ZHANG Xiangdong,ZHANG Jianjun. Stability analysis of vertical shaft surrounding rock and supporting system in deep fault fracture[J]. Journal of China Coal Society,2013,38(4):587−594.
[24] 孙闯,张向东,李永靖. 高应力软岩巷道围岩与支护结构相互作用分析[J]. 岩土力学,2013,34(9):2601−2607. SUN Chuang,ZHANG Xiangdong,LI Yongjing. Analysis of interaction between surrounding rock and support structure in high stressed soft rock roadway[J]. Rock and Soil Mechanics,2013,34(9):2601−2607.
[25] 崔岚,郑俊杰,苗晨曦,等. 隧道纵向变形曲线与围岩特征曲线耦合分析[J]. 岩土工程学报,2014,36(4):707−715. CUI Lan,ZHENG Junjie,MIAO Chenxi,et al. Coupling analysis of longitudinal deformation profile and ground reaction curve[J]. Chinese Journal of Geotechnical Engineering,2014,36(4):707−715.
[26] 梁明纯,苗胜军,蔡美峰,等. 考虑剪胀特性和峰后形态的岩石损伤本构模型[J]. 岩石力学与工程学报,2021,40(12):2392−2401. LIANG Mingchun,MIAO Shengjun,CAI Meifeng,et al. A damage constitutive model of rock with consideration of dilatation and post-peak shape of the stress-strain curve[J]. Chinese Journal of Rock Mechanics and Engineering,2021,40(12):2392−2401.
[27] 刘泉声,邓鹏海,毕晨,等. 深部巷道软弱围岩破裂碎胀过程及锚喷−注浆加固FDEM数值模拟[J]. 岩土力学,2019,40(10):4065−4083. LIU Quansheng,DENG Penghai,BI Chen,et al. FDEM numerical simulation of the fracture and extraction process of soft surrounding rock mass and its rockbolt-shotcrete-grouting reinforcement methods in the deep tunnel[J]. Rock and Soil Mechanics,2019,40(10):4065−4083.
[28] ALEJANO L R,RODRIGUEZ-DONO A,ALONSO E,et al. Ground reaction curves for tunnels excavated in different quality rock masses showing several types of post-failure behaviour[J]. Tunnelling and Underground Space Technology,2009,11(6):689−705.
[29] GSCHWANDTNER G G,GALLER R. Input to the application of the convergence confinement method with time-dependent material behaviour of the support[J]. Tunnelling and Underground Space Technology,2012,12(2):13−22.
[30] 王从凯. 基于收敛-约束法的深部巷道围岩与支护相互作用机制研究[D]. 徐州:中国矿业大学,2021:9-61. WANG Congkai. The research on the interaction between the deep surrounding rock and the supporting structure based on characteristic curve method[D]. Xuzhou:China University of Mining and Technology,2021:9-61.
[31] 孟庆彬,韩立军,张帆舸,等. 深部高应力软岩巷道耦合支护效应研究及应用[J]. 岩土力学,2017,38(5):1424−1444. MENG Qingbin,HAN Lijun,ZHANG Fange,et al. Coupling support effect on high-stress deep soft rock roadway and its application[J]. Rock and Soil Mechanics,2017,38(5):1424−1444.
[32] 康红普. 我国煤矿巷道围岩控制技术发展70年及展望[J]. 岩石力学与工程学报,2021,40(1):1−30. KANG Hongpu. Seventy years development and prospects of strata control technologies for coal mine roadways in China[J]. Chinese Journal of Rock Mechanics and Engineering,2021,40(1):1−30.
[33] 吴政,梁斌,祝方才,等. 基于FLAC3D的深部隧道分区破裂特性研究[J]. 湖南工业大学学报,2022,36(6):15−21. WU Zheng,LIANG Bing,ZHU Fangcai,et al. Research on zonal fracture characteristics of deep tunnels based on FLAC3D[J]. Journal of Hunan University of Technology,2022,36(6):15−21.
[34] MENG Q B,LIU J F,REN L,et al. Experimental study on rock strength and deformation characteristics under triaxial cyclic loading and unloading conditions[J]. Rock Mechanics and Rock Engineering,2021,54(3):777−797.
[35] 孟庆彬,韩立军,张建,等. 深部高应力破碎软岩巷道支护技术研究及其应用[J]. 中南大学学报(自然科学版),2016,47(11):3861−3872. MENG Qingbin,HAN Lijun,ZHANG Jian,et al. Research and application of supporting technology in deep high stress fractured soft-rock roadway[J]. Journal of Central South University (Science and Technology),2016,47(11):3861−3872.
计量
- 文章访问数: 114
- HTML全文浏览量: 21
- PDF下载量: 47
