Experimental study on characterization hydraulic fracturing coal fracture network and evolution of fracture forming performance
-
摘要:
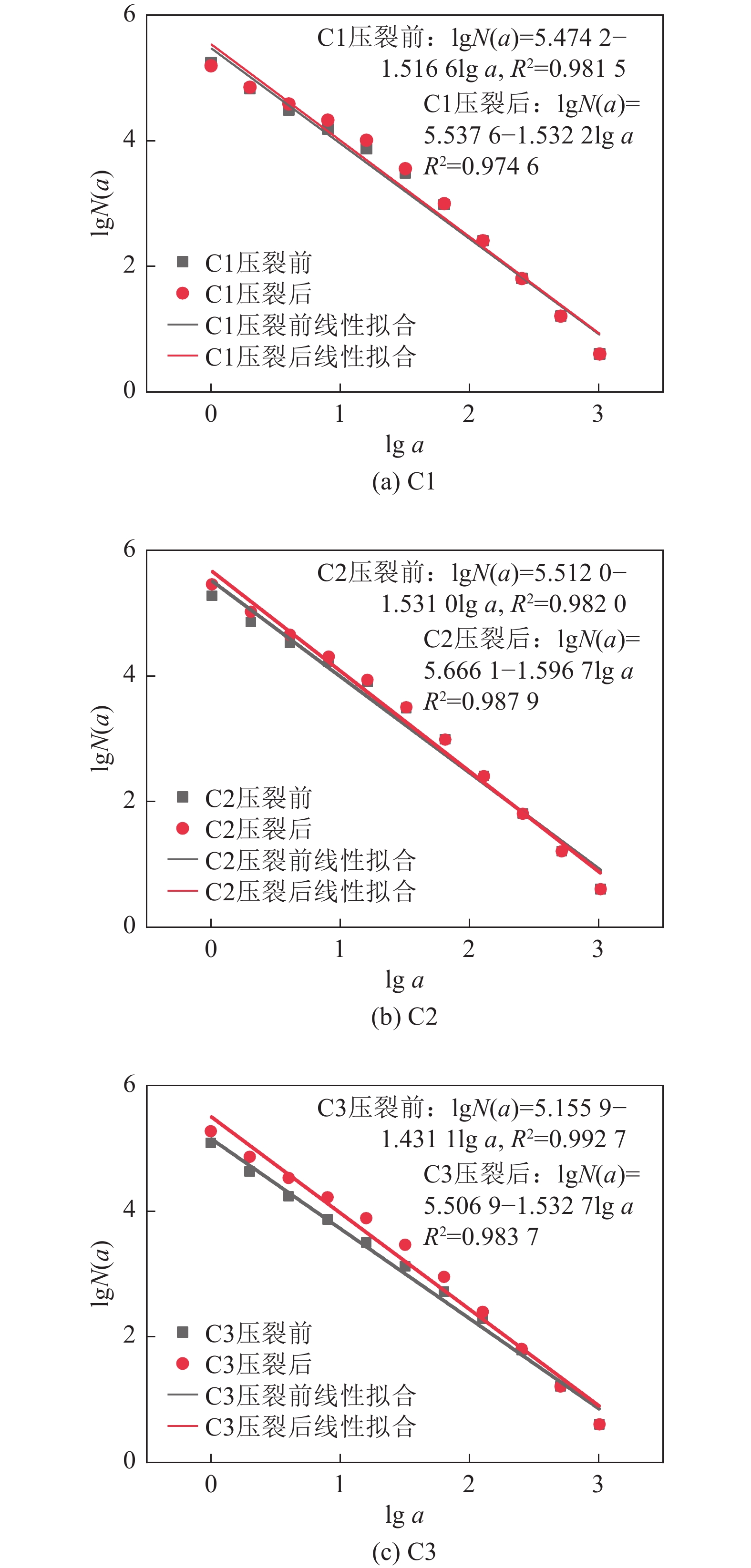
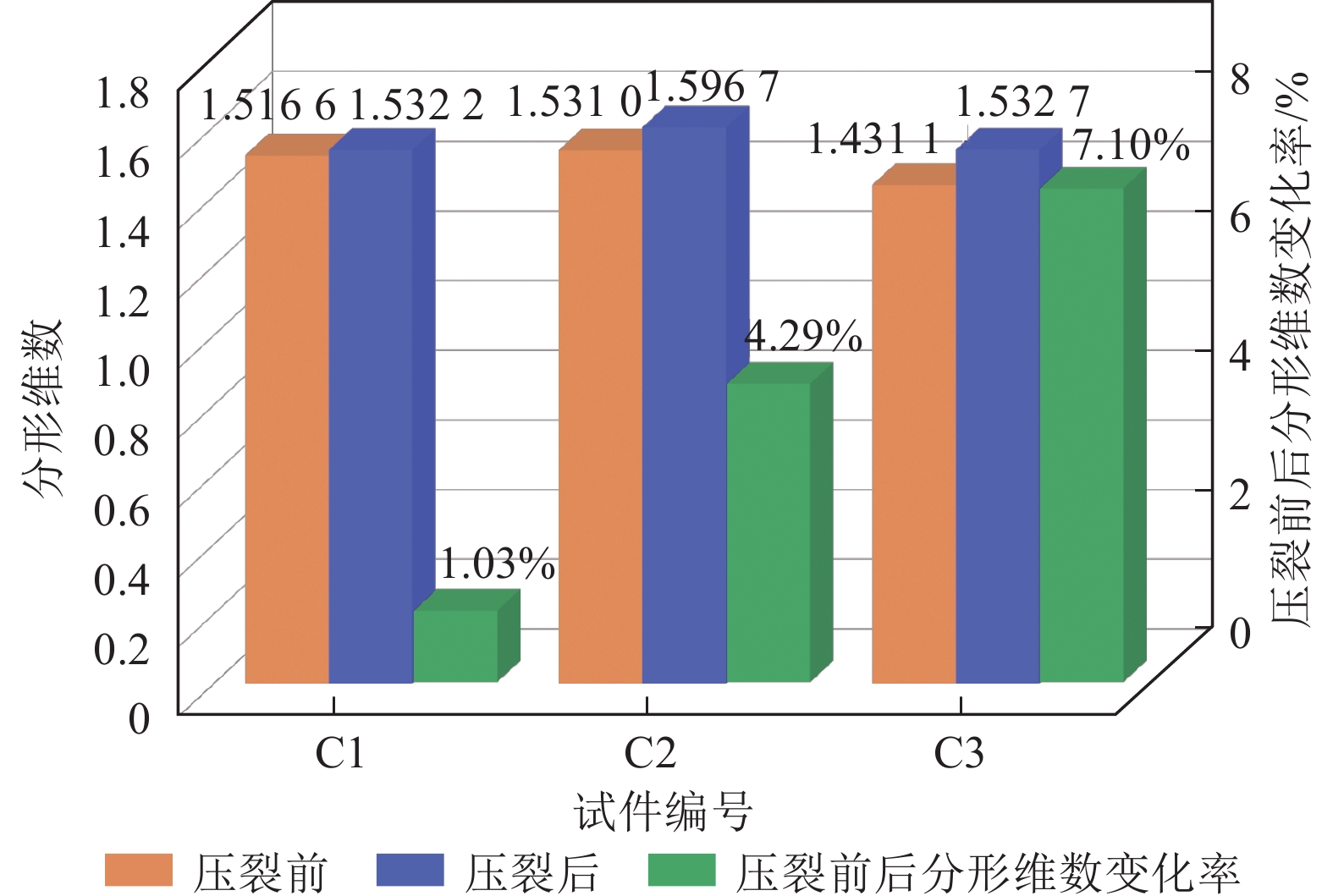
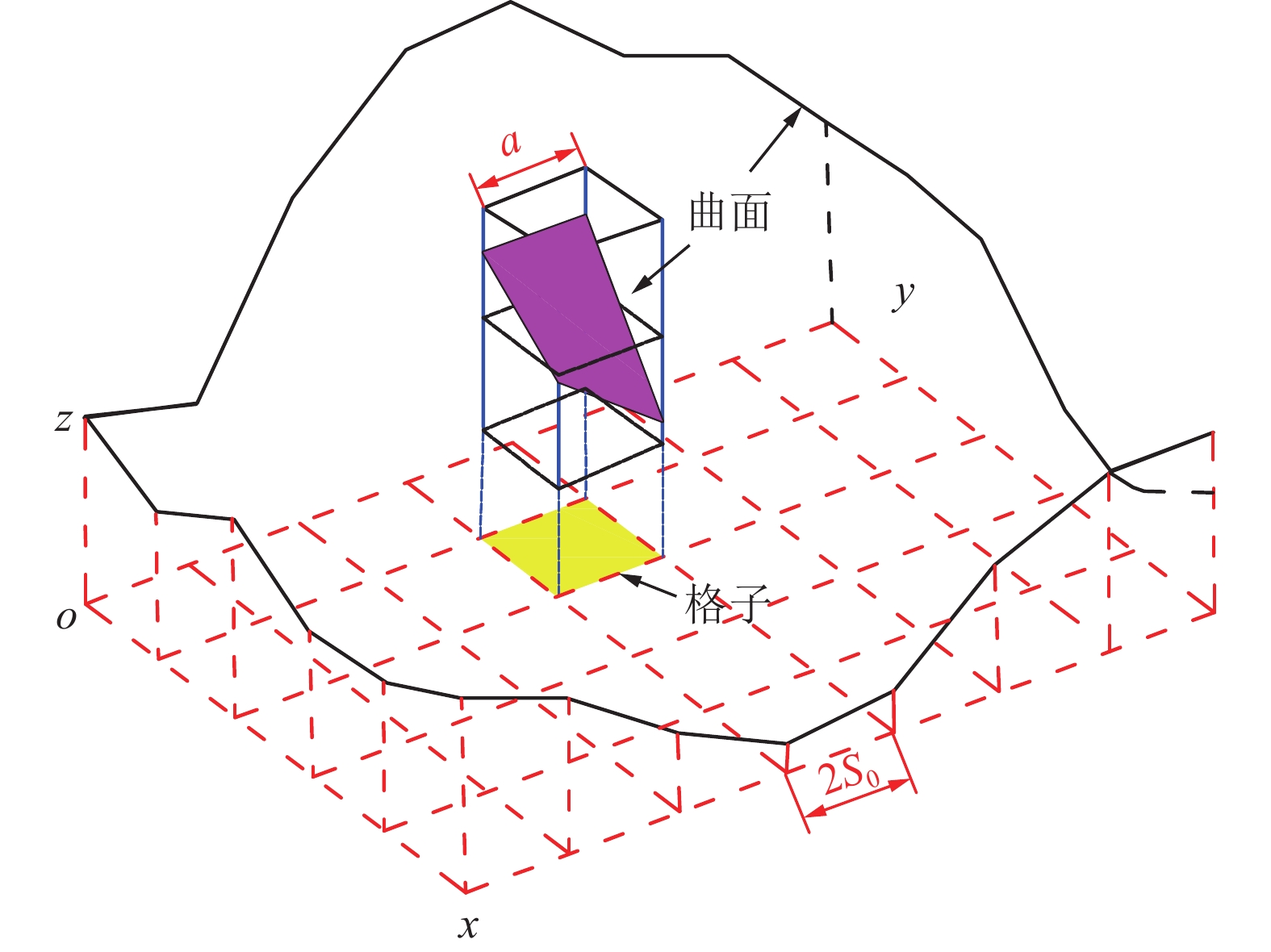
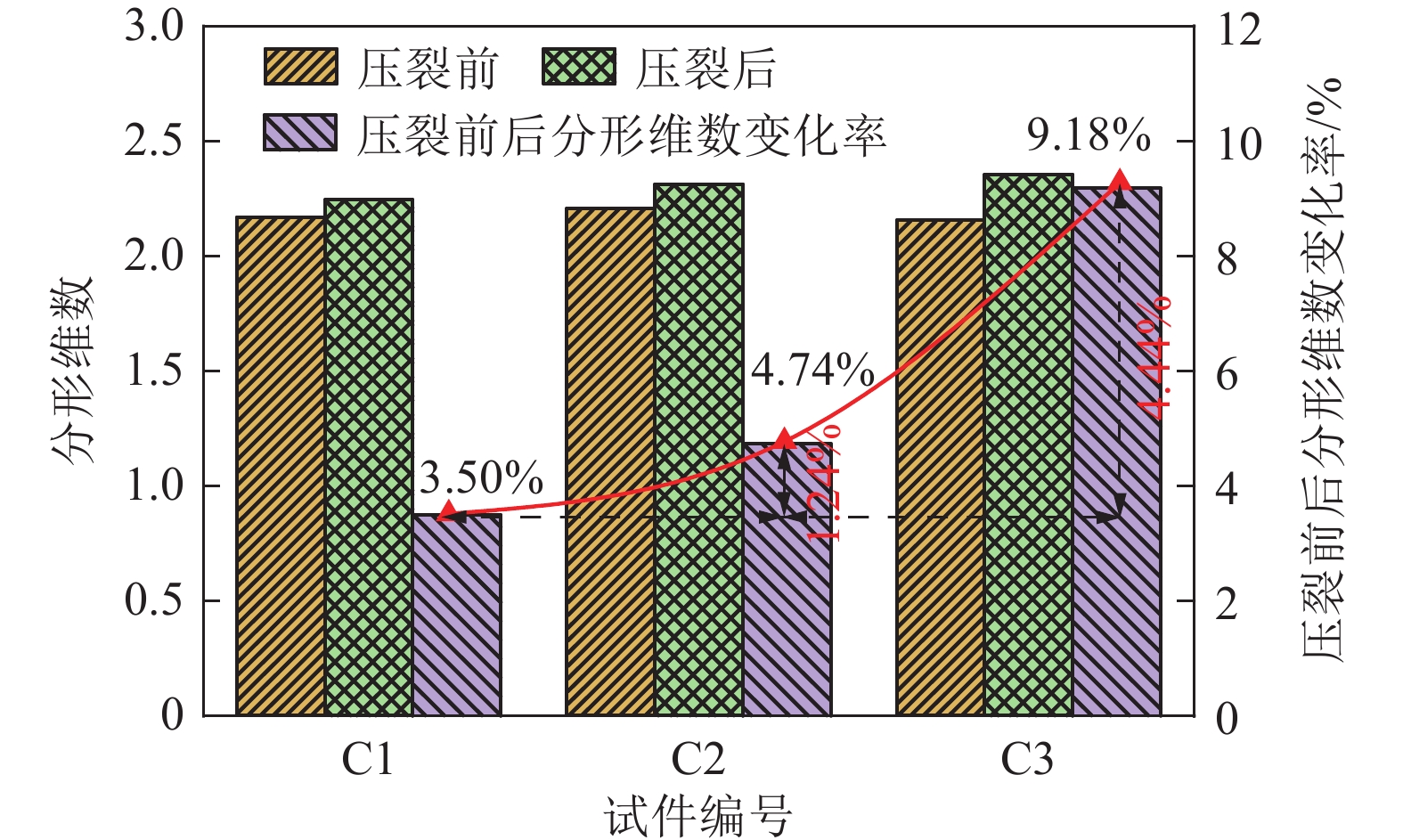
煤裂隙网络的准确表征可以有效评估深部煤层经水力压裂后的压裂效果，为了定量评估煤层经水力压裂后的复杂程度，利用自制的真三轴试验系统进行了煤的水力压裂试验，结合CT扫描，重建了具有拓扑结构的孔裂隙网络，用分形理论和拓扑学定量表征了断裂网络的复杂程度。探究了在真三轴应力条件下，中间主应力对裂缝网络复杂程度的影响。结果表明：压裂后煤样的二维分形维数变化率K为1.03%~7.10%，三维分形维数的变化率为3.50%～9.18%，经过水力压裂后煤样的二维和三维分形维数均显著增大。基于分形理论和拓扑学的方法能有效表征水力压裂的裂缝结构和造缝能力。压裂后煤样的二维拓扑参数为1.18～1.52，与压裂前后煤样的二维分形维数变化率呈正相关关系，二维分形维数变化率的增加速率随着拓扑参数的增大逐渐减小。重构的内部结构和裂缝分布表明，压裂后的三维分形维数比二维分形维数更具优势，压裂后煤样的三维拓扑参数为1.82～1.93，随着三维分形维数变化率的增加而增大，三维分形维数变化率的增加速率随着拓扑参数的增大而增大。中间主应力对煤层的造缝能力存在积极影响。水力压裂前后的分形维数和拓扑参数都随着中间主应力的增大而增大，即随着中间主应力的增大而增大，产生的裂缝网络更复杂，连通性更好，水力压裂的造缝能力更强。
Abstract:The accurate characterization of coal fracture network can effectively evaluate the fracturing effect of the deep coal seam after hydraulic fracturing. In order to quantitatively evaluate the complexity of coal seam after hydraulic fracturing, the hydraulic fracturing test of coal was carried out by using the self-made true triaxial test system, combined with CT scan, the pore network and fracture network with topological structure are reconstructed, and the complexity of the fracture network is quantitatively characterized by fractal theory and topology. The effect of the intermediate principal stress on the complexity of the fracture networks under the condition of true triaxial stress is explored. The results showed that the change rate of the two-dimensional fractal dimension (K) are 1.03% ~ 7.10%, and the change rate of three-dimensional fractal dimension are 3.50% ~ 9.18%, the two-dimensional and three-dimensional fractal dimensions of coal samples after hydraulic fracturing increase significantly. The method based on fractal theory and topology can effectively characterize the fracture structure and fracture forming ability of hydraulic fracturing. The two-dimensional topological parameter of coal samples after fracturing are1.18 ~ 1.52, which is positively correlated with the change rate of two-dimensional fractal dimension．Increasing rate of change rate of two-dimensional fractal dimension decreases with the increase of topological parameters. The reconstructed internal structure and fracture distribution showed that the three-dimensional fractal dimension after fracturing is more advantaged than the two-dimensional fractal dimension. The three-dimensional topological parameters of coal samples after fracturing are 1.82-1.93, increased with the increase of the change rate of three-dimensional fractal dimension. The intermediate principal stress has a positive effect on the seam forming ability of coal seam. The fractal dimension and topological parameters increase with the increase of intermediate principal stress before and after hydraulic fracturing. In other words, Increased with the intermediate principal stress, the resulting fracture network is more complex, the connectivity is better, and the fracture forming ability of hydraulic fracturing is stronger.
-
0. 引 言
对旋风机作为矿井广泛使用的主通风设备，其在小流量工况运行时极易出现旋转失速乃至喘振等失稳现象，严重影响其安全运行[1-2]。两级动叶之间的轴向间距作为对旋风机的重要结构参数，不仅与其效率、全压等性能参数密切相关，而且对对旋风机运行的稳定性也具有重要影响[3-4]。
大量研究表明，失速起始扰动具有2种典型的类型，一种是发展速度相对较慢、线性的大尺度模态型；另一种是发展速度相对较快、非线性的小尺度突尖型。MOORE和GREITZER[5]从系统稳定性的角度出发建立了压缩系统稳定性模型，该模型模拟得到压气机旋转失速起始的模态波，并认为压气机的旋转失速是由模态波引起的。VO等[6]提出了“突尖型”失速起始扰动出现的先决条件，即叶顶泄漏流发生前缘溢流和尾缘反流。然而，由于压气机、风机设计的多样性，现有文献中也常出现不同于模态波型和突尖波型的失速起始扰动类型。DELL’ERA等[7]发现了一种不同于模态波和突尖波的压气机叶根失速起始扰动，且其类型随工作转速不同而改变。YAMADA等[8]在所研究的压气机中发现，失速是从轮毂侧的分离开始的，随着分离范围的增长，逐步发展为旋转失速。李思敏等[9]、潘天宇等[10]在一台低速轴流压气机上，发现了特征为低频、轴对称、首发于叶根区域且其形成的失速团以较低转速沿周向旋转的失速起始扰动，称其为“局部喘振”。武文倩等[11]在实验中发现了起始于叶根的新型压气机失速起始形式，并通过数值方法对该压气机进行了研究，结果表明，静子叶根区域首先形成堵塞区，验证了失速起始扰动起源于叶根区域。XU等[12]研究了不同轴向间距下的压力损失分布，结果表明：当轴向间距减小时，静子的入射角减小，吸力侧的流动分离得到抑制，从而提高了升压能力。李传鹏等[13]发现轴向间距对压气机失速点流量影响显著，转子与静子轴向距离减小时，压气机失速推迟，并且压气机进入旋转失速的方式与轴向间距有关，轴向间距较大时，压气机进入多涡团全叶高旋转失速。HEWKIN-SMITH等[14]、杜鹃等[15]研究了压气机失速过程中叶顶间隙泄漏流前移的动力学机制，结果表明随着压气机的节流，叶顶泄漏流与主流的轴向动量比逐渐增大，从而推动压气机进入失速状态。JIANG等[16]和强冠杰等[17]研究了轮毂角区径向涡流与叶顶泄漏流相互作用引发叶顶失速的发生机制。总之，轴流压气机在不同设计或工作条件下的失速起始扰动类型、起始位置、发展及传播过程都存在显著差异。
通过数值模拟研究不同轴向间距下对旋风机的失速起始及其发展过程，揭示轴向间距对失速起始及其发展过程的影响规律，对于优化对旋风机结构及提高其运行稳定性具有重要意义。
1. 几何模型与网格划分
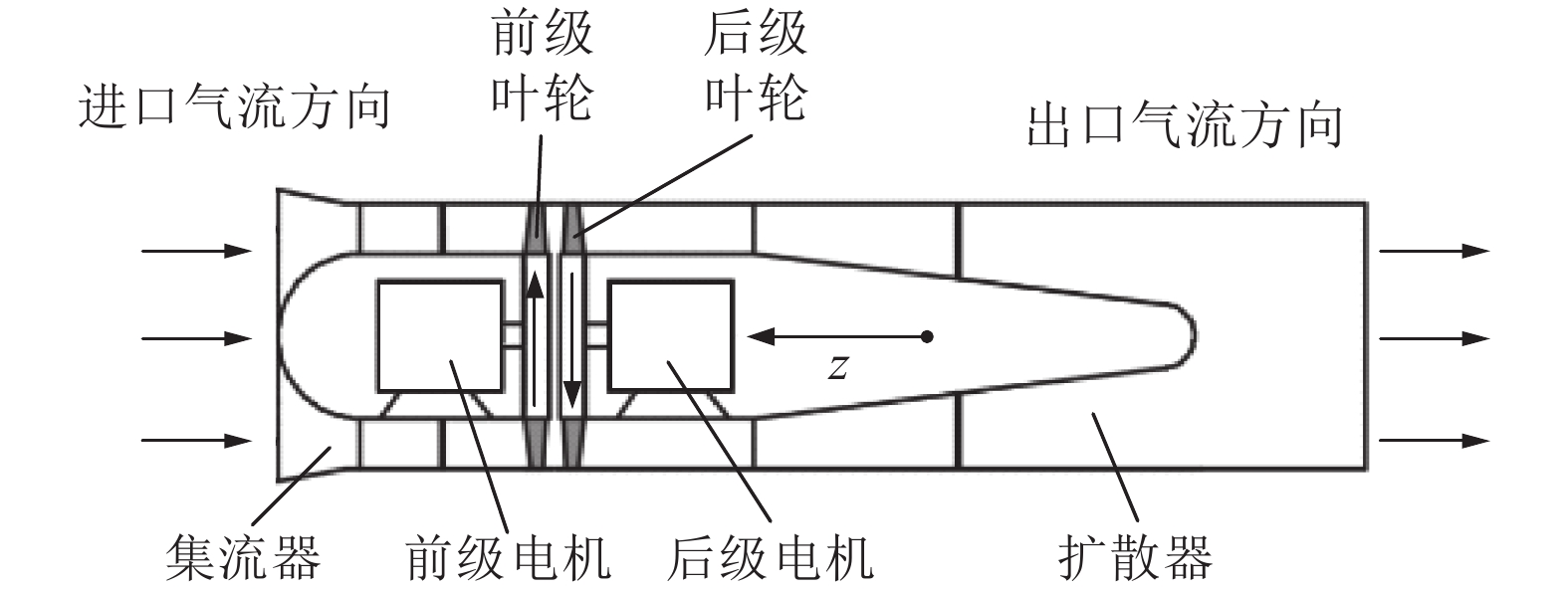
以FBCDZ-10-No20型对旋风机(下文简称风机)为对象，其设计工况流量为75 m3/s，前、后级叶片数分别为19、17，叶顶间隙为2 mm，两级转子额定转速为980 r/min，图1为其结构简图。
图2为5种轴向间距下风机全压效率随网格数的变化情况。当网格总数达到650万时，全压效率已基本保持不变，因此4种间距下整体计算域采用的网格数均为650万左右。
2. 数值模拟方法
2.1 数值计算方法
采用能够精准预测逆压梯度流动的SST（shear stress transport）k-ω湍流模型[18]，非定常计算采用隐式双时间步推进法，时间步长设为0.000 3 s，即在一个时间步内叶轮转过1.8°。
2.2 边界条件设置
数值模拟计算域以集流器入口、扩散器出口为入、出口边界，入口边界设置为总压入口，相对总压值为0；出口边界为静压出口；进气方式为轴向均匀进气；所有壁面均设为无滑移边界；计算过程中，通过逐步提升背压的方式逼近失速点。
2.3 监测点布置
为更好地了解风机叶轮内部的压力脉动情况，在后级叶轮通道内部设置压力监测点M1、M2、M3，布置方式如图3所示，M1、M2、M3分别位于10%、50%、90%叶高处。
3. 模拟结果分析
3.1 轴向间距对风机性能的影响
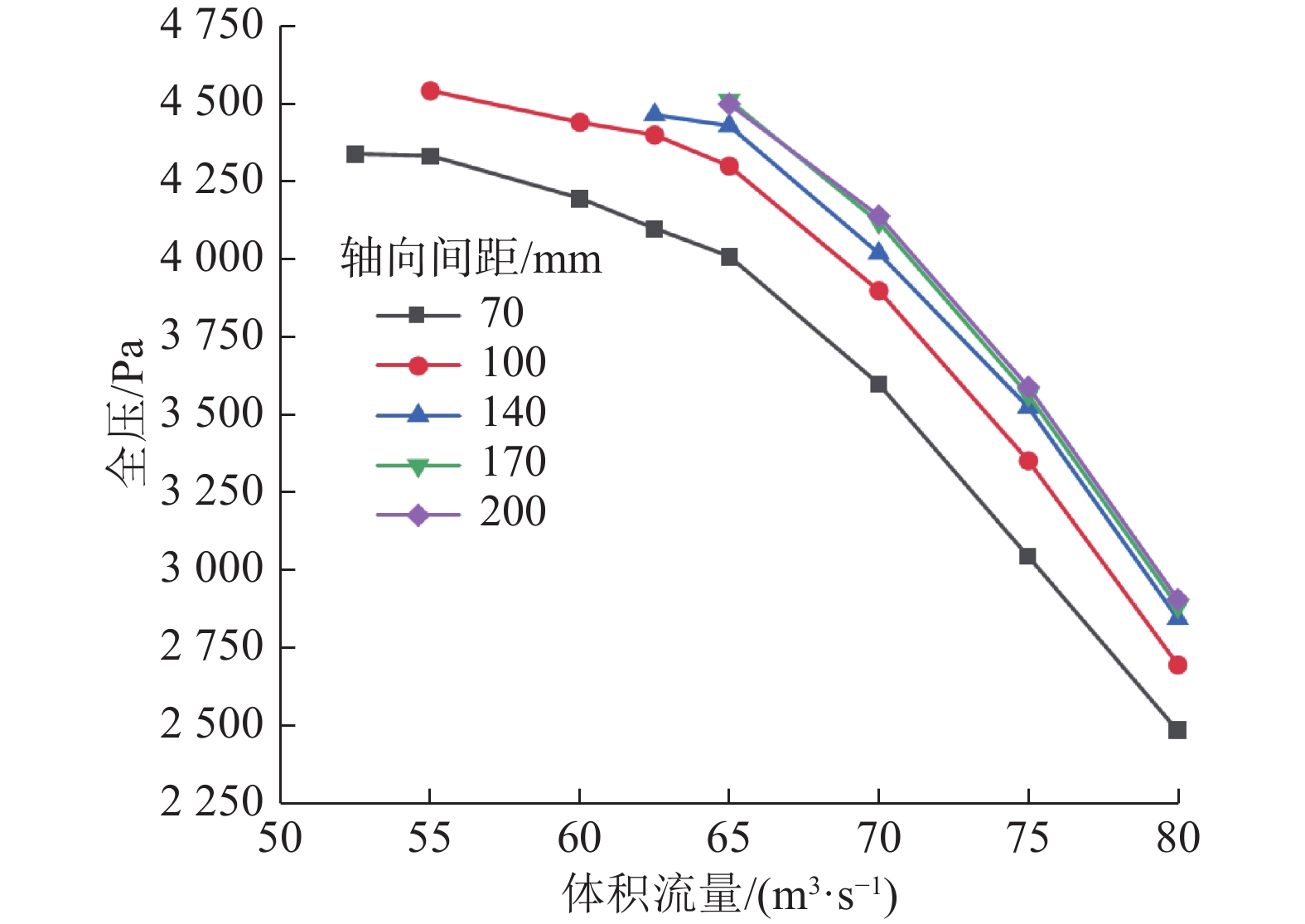
图4为5种轴向间距下的风机特性曲线。可以看出，随着轴向间距的增大，风机的失速临界流量逐渐增大，稳定运行范围逐渐缩小。根据计算结果及文献[6，10]，将5种不同轴向间距下的失速类型分为2类，一类是70、100、140 mm下的突尖型失速起始扰动；另一类是170、200 mm下的局部喘振型失速起始扰动。为此，选取了具有代表性的间距(70、170 mm)，用来揭示不同轴向间距时的失速起始扰动类型和失速发展过程。选取70 mm轴向间距是因为其为该型对旋风机的设计轴向间距，且其失速临界流量最小，失速裕度最大；选取170 mm轴向间距是由于当轴向间距增至170 mm后，失速临界流量已基本维持不变。另外，在相对合理的轴向间距范围内，2种间距下的失速临界流量相差最大且其涵盖了所出现的失速起始扰动类型。
3.2 轴向间距为170 mm时风机的失速过程分析
3.2.1 失速起始扰动的产生
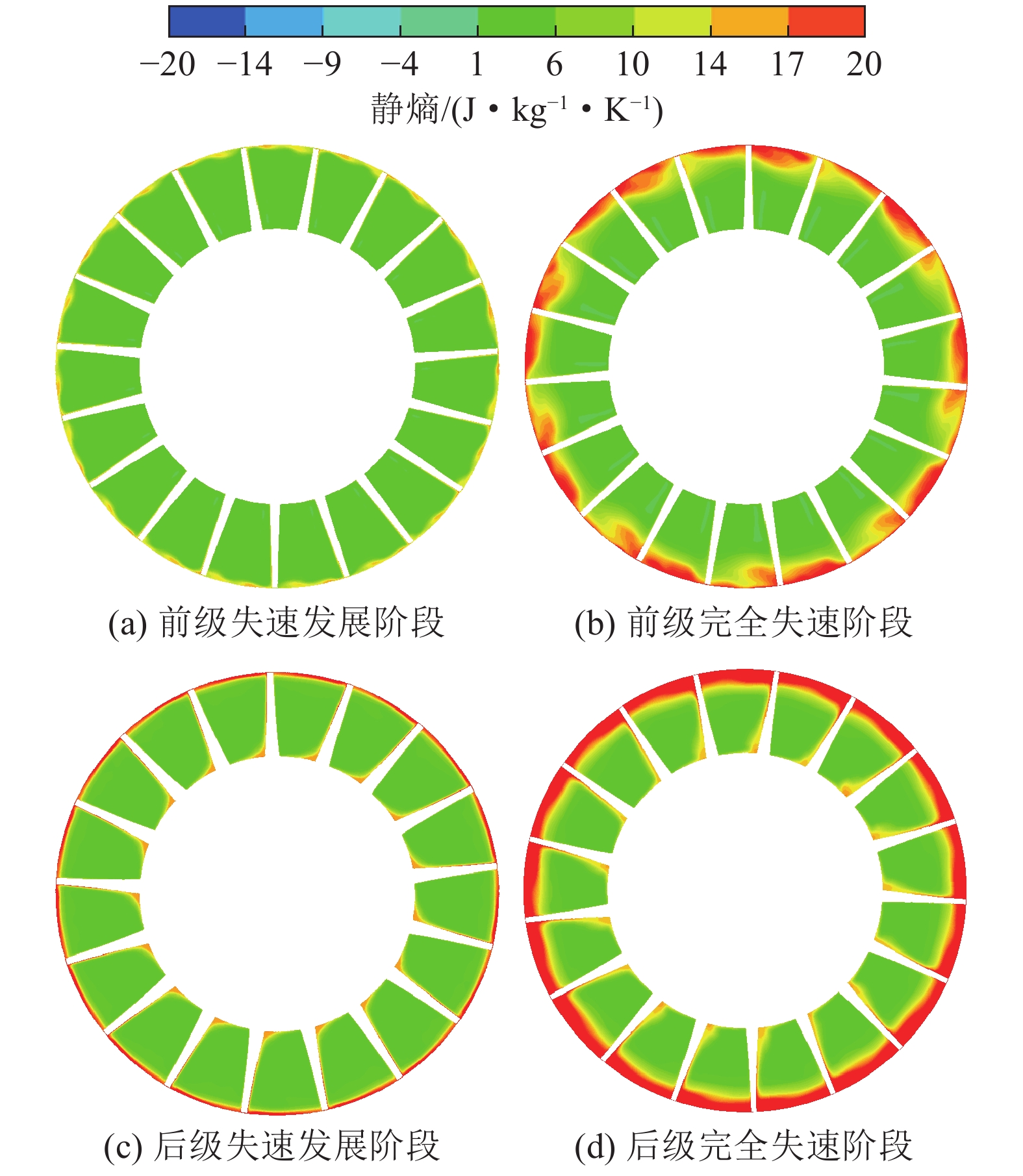
角区分离通常是指在叶片吸力面尾缘和轮毂相连接的角区内出现的大面积分离、回流现象。图5为近失速阶段两级叶轮在叶高5%、95%处的速度矢量图，图6给出了近失速阶段两级叶轮的流线和不同叶高环面的静熵分布。由图5a和图6可知，随着出口背压的提高，后级叶轮的叶根吸力面尾缘区域首先出现失速起始扰动，并伴有角区分离，这种分离会造成低能流体在叶根区域聚集，而后在逆压梯度及离心力的作用下，形成沿吸力面尾缘上升的径向涡流，从而使叶根出现高熵区；前级叶片通道内的流动状态良好。由图5b和图6可知，两级的叶顶区域都未出现明显的流动分离现象。
![]()
图 5 近失速工况两级叶轮的速度矢量Figure 5. The velocity vectors distribution of two-stage impellers under near stall condition![]()
图 6 两级叶轮流线和不同叶高环面静熵分布Figure 6. The streamline distribution of the two-stage impellers and static entropy distribution of the different blade height sections3.2.2 失速起始扰动的发展
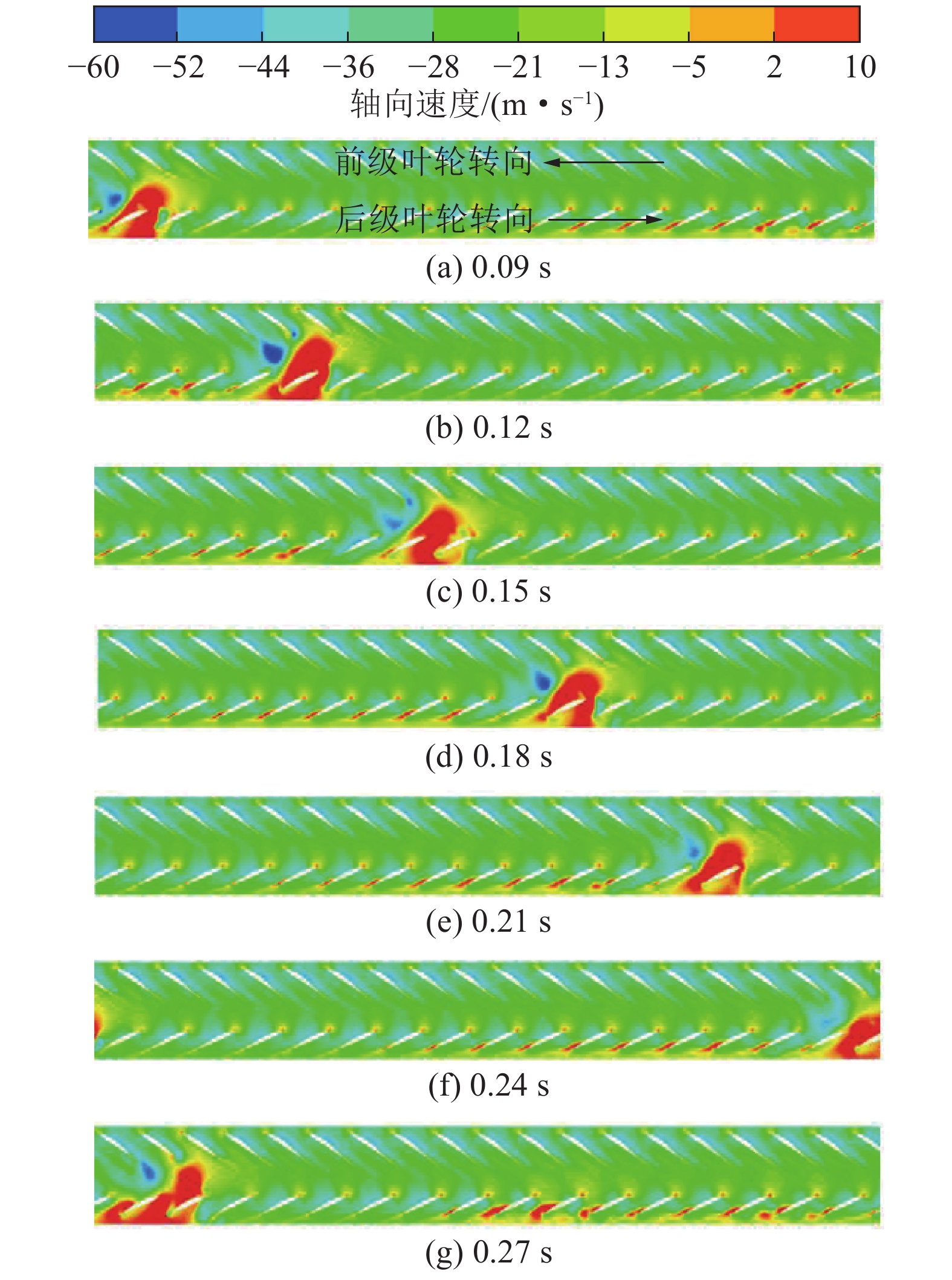
图7为失速发展过程中不同时刻后级叶轮z=1.55 m(50%轴向弦长)截面处的静熵云图。失速涡团的整个发展过程历经约300个时间步，即0.09 s，并以出现失速起始扰动的时刻作为起始时刻。由图7a可见，设计流量下，后级叶片通道内流动良好，仅在叶顶存在因泄漏流引起的局部高熵区域。由图7b可见，后级叶根吸力面的径向涡流整体增强，从而引起叶根的高熵区扩大，各叶片通道内高熵区域的分布基本一致。由图7c可见，径向涡流在压力梯度和离心力的作用下进一步向叶顶迁移，此时堵塞区已沿径向延伸至各通道40%叶高处。由图7d—图7e可见，随着失速过程的发展，高熵区域沿周向呈现出明显的分布规律，即分散在各个通道内的径向涡流逐渐向某几个叶片通道聚集并增强，高熵区域沿径向自叶根扩展至80%叶高处，此时失速涡团已见雏形。由图7e—图7h可见，在风机进入完全失速过程中，失速涡团雏形在沿周向旋转过程中不断卷吸各通道内由角区分离产生的低能流体，从而形成成熟的失速涡团。
![]()
图 7 失速发展过程中不同时刻后级叶轮的静熵分布Figure 7. Static entropy distribution of the rear-stage impeller developed from design conditions to full stall conditions3.2.3 失速涡团沿后级转向的传播规律
图8为完全失速阶段两级叶轮在不同时刻5%叶高处的轴向速度分布，以图7中成熟失速涡团的形成时刻0.09 s作为图8的起始时刻。因为风机的进气方向与z轴正方向相反，所以轴向反流区域的速度为正值。可见，成熟的失速涡团占据约2个叶片通道，为单涡团失速，其运动方向与后级叶轮的旋转方向相同，其旋转一周耗时0.18 s，而后级叶轮旋转周期仅需0.06 s，即失速涡团的转速约为后级叶轮转速的33.3%。失速涡团沿周向的传播机制与二维Emmons[19]模型类似，即进入失速的通道流动迅速恶化，退出失速的通道流动得到改善。
3.2.4 失速涡团的轴向传播规律
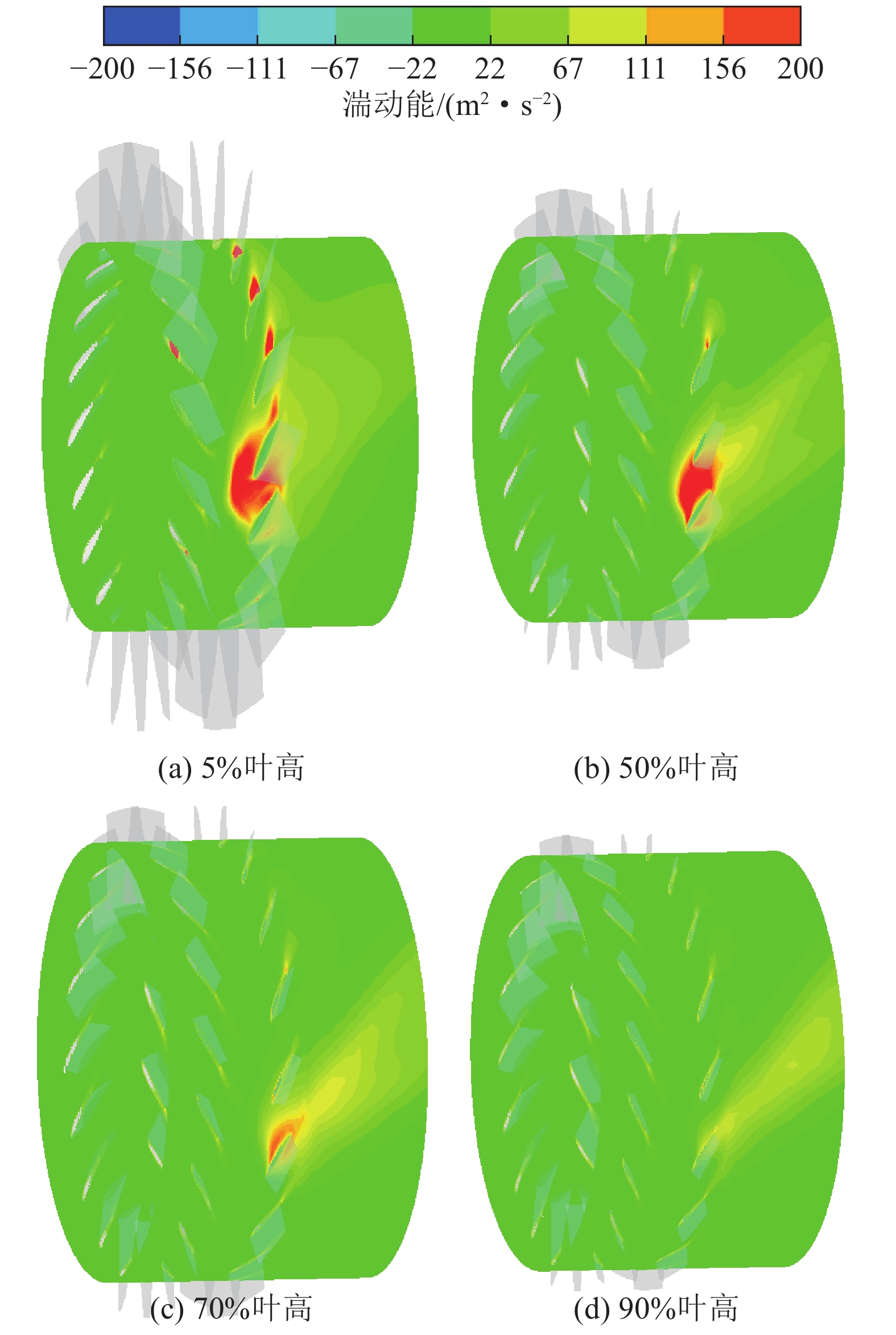
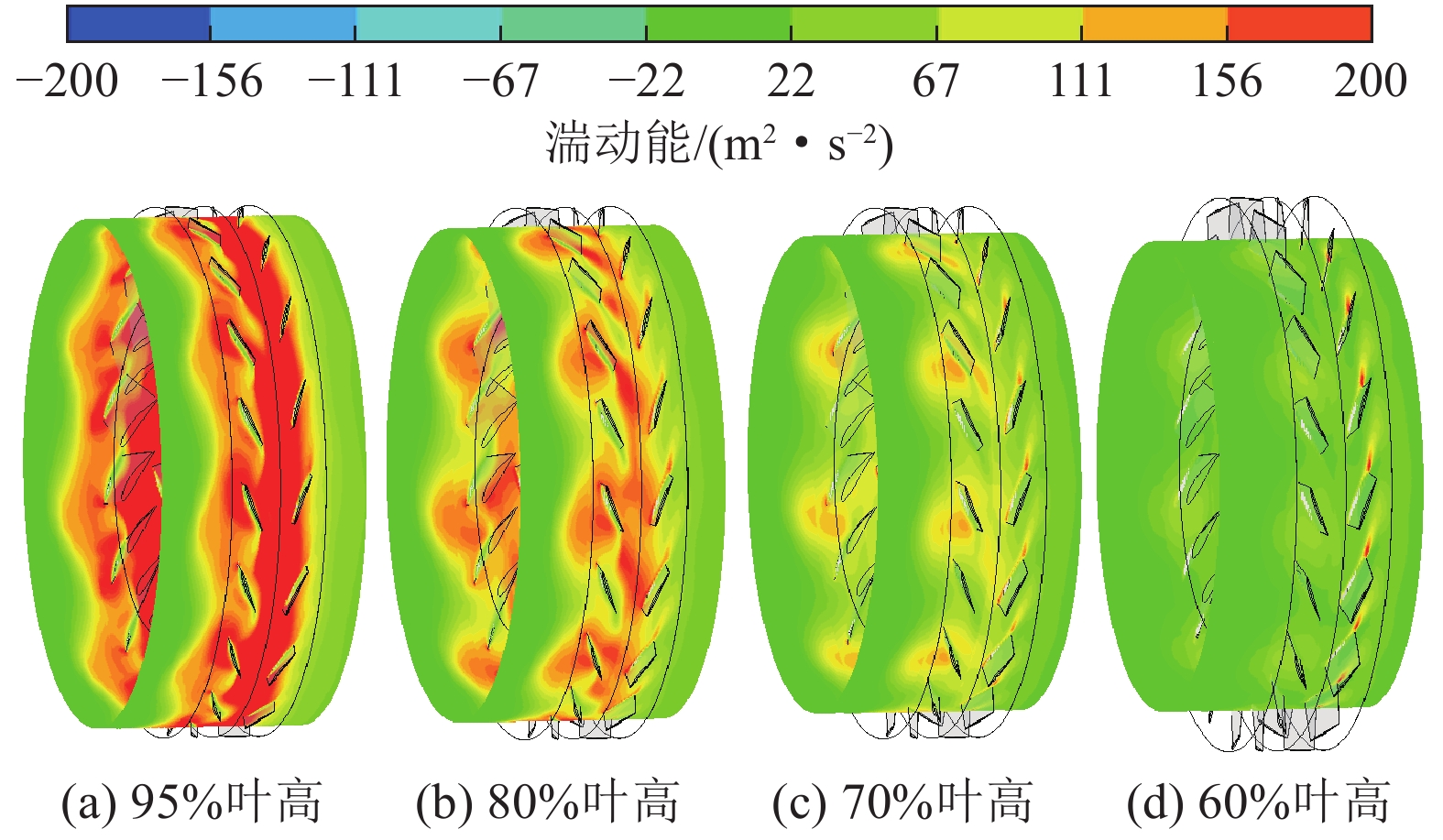
图9为完全失速阶段不同叶高环面的湍动能分布。沿轴向失速涡团扰动并未向前级叶轮区域传播而是限于后级叶轮和级间通道内，这是由于上游尾迹的干扰作用，抑制了失速涡团扰动向上游的传播；但其对于后级叶轮下游区域的影响范围较大，在5%叶高环面的下游4倍弦长处依然存在较大的高湍动能区。随着叶高的增加，失速涡团对于后级叶轮下游区域的影响范围变小，强度减弱。
![]()
图 9 完全失速阶段不同叶高环面的湍动能分布Figure 9. Turbulent kinetic energy distribution of the different blade height sections under full stall conditions3.2.5 失速涡团的径向传播规律
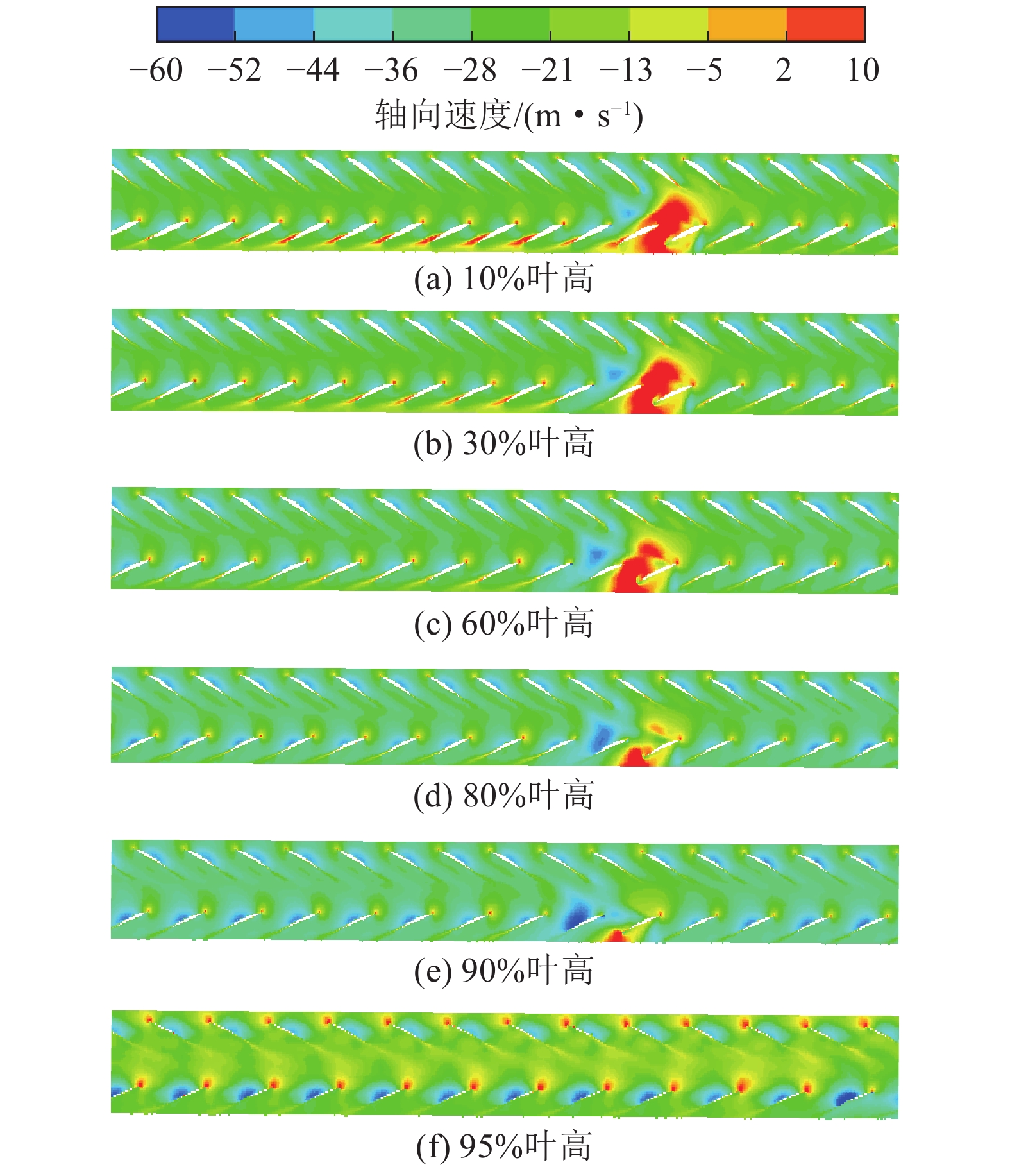
图10为完全失速阶段两级叶轮在同一时刻不同叶高处的轴向速度云图。由图10a—图10e可见，因径向涡流引起的两级区域的轴向反流面积随叶高的增加，逐渐减小，在90%叶高处达到最小，仅占距一个叶片通道，轴向反流的强度也最弱。由图10e—图10f可见，此时由径向涡流引起的轴向反流面积已经很小，而由叶顶泄漏涡引起的反流面积却在不断扩大，2种不同成因的反流区在90%以上的某一叶高处汇合，形成全叶高失速涡团。
![]()
图 10 不同叶高截面的轴向速度分布Figure 10. Velocity w distribution of the different blade height sections为分析径向涡流和叶顶泄漏流相互作用的规律，图11给出完全失速阶段后级叶轮叶片通道的流线分布。如图10中a区域所示，叶根区域的径向涡流速度较低，扭曲变形严重，但其影响范围较小，聚集于叶片吸力面的尾缘附近；如图11中b区域所示，当径向涡流到达叶顶区域后，其速度有所提高，影响范围扩大，并在逆压梯度的作用下出现轴向反流，堵塞后级叶轮通道的下游区域。由特性曲线分析易知，风机在轴向间距为170 mm时更易发生失速，原因可能在于径向涡流从叶根区域向叶顶区域迁移的过程中堵塞了叶根及后级叶顶的下游区域，从而使叶顶泄漏流无法随通道主流向下游流动，而是演化为叶顶泄漏涡，堵塞叶顶通道，使失速提前发生。
![]()
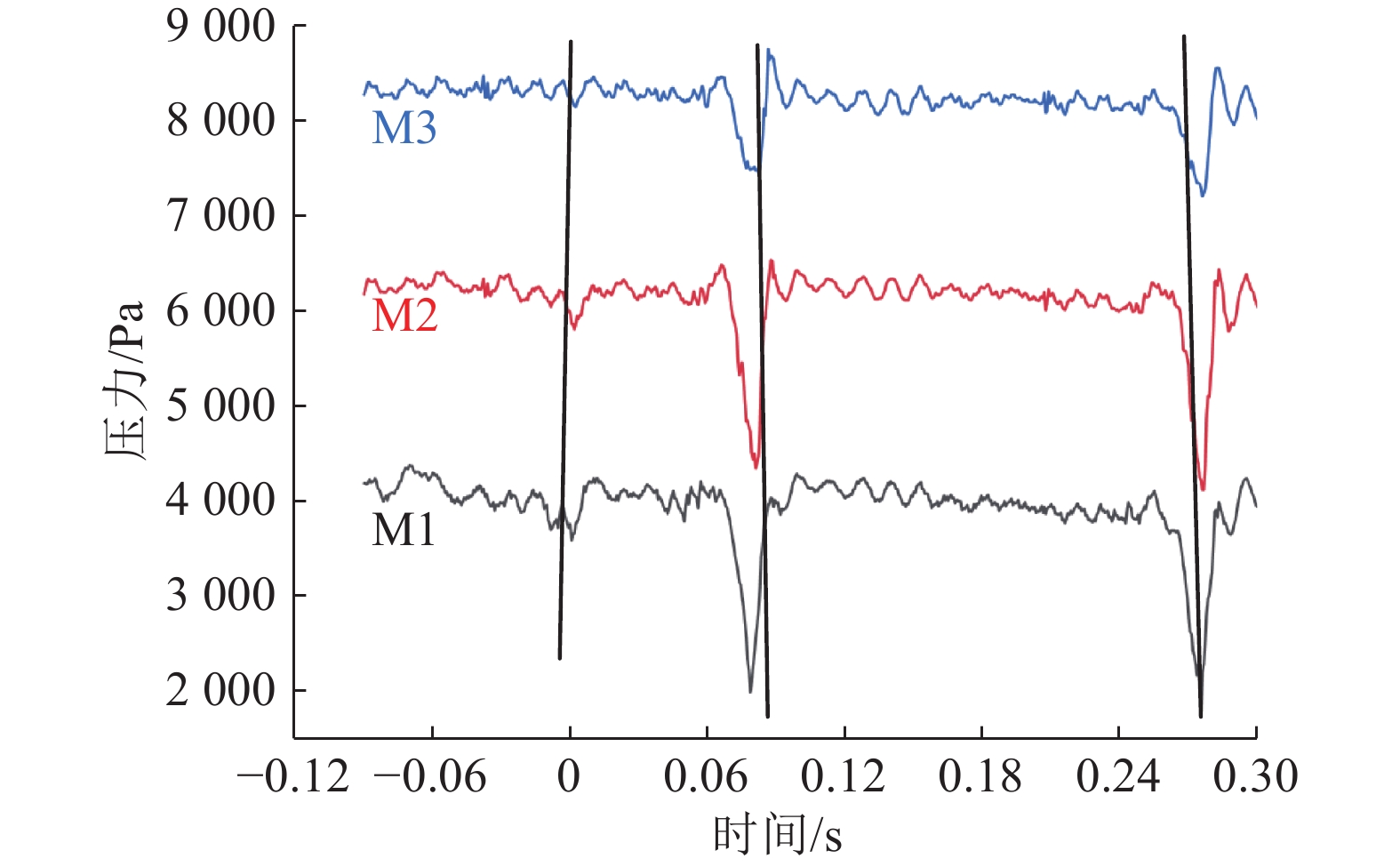
图 11 完全失速阶段叶片通道内的流线Figure 11. The streamline distribution of the blade channel under full stall conditions3.2.6 旋转失速过程中径向监测点的压力信号
前文已对170 mm轴向间距下风机失速过程的流场结构进行分析，图12进一步给出风机在失速过程中后级叶轮监测点所监测到的动态压力信号，图中横坐标表示失速过程所对应的时间，第0 s表示失速起始时刻。为使压力信号便于观察，将M2、M3在M1的基础上分别向上平移2 000、4 000个单位。由图可知，从失速起始扰动发展为成熟失速涡团所需时间约0.09 s，与图7中失速起始扰动发展时间一致；另外，由图中M1点的压力脉动情况可得，失速团的转速约为后级叶轮转子转速的33.3%，与图8所得转速一致。从径向上看，M1、M2、M3能够同时监测到失速涡团的存在，说明该失速涡团为全叶高失速涡团。无论是失速起始阶段还是完全失速阶段，风机内部的压力脉动程度均是随着叶高的增加逐渐减弱，这是由于失速起始扰动及失速涡团均是在叶根区域产生并逐渐向叶顶区域发展。由图12结合图5a和图7b可以推断，轴向间距为170 mm时，风机失速起始扰动的类型为“局部喘振型”，其符合潘天宇等[7]提出的失速起始扰动特征。
![]()
图 12 后级叶轮内监测点的压力脉动时域Figure 12. Pressure fluctuation time domain diagram of monitoring points of the rear-stage impeller3.3 两种轴向间距失速起始及其发展过程的对比
图13给出近失速阶段70 mm轴向间距下两级叶轮不同叶高处的速度矢量分布，由图13b可知，随着出口背压的提高，前级的叶顶区域首先出现失速起始扰动，并伴有前缘溢流和尾缘反流，而后级叶片通道内的流动良好。由图13a可知，两级叶轮叶根区域均未见明显的分离现象。结合文献[20]，轴向间距为70 mm时，风机的失速起始扰动为“突尖型”，符合VO等[4]提出的失速起始扰动特征，这明显不同于轴向间距为170 mm的情况。
![]()
图 13 轴向间距为70 mm时不同叶高截面的速度矢量Figure 13. The velocity distribution of different blade height sections under the axial spacing of 70 mm图14—图15为70 mm轴向间距下两级静熵及湍动能分布。由图14可知，在失速发展阶段，两级的叶顶区域产生了整周的失速扰动，随着失速的发展，失速扰动也未能在某几个叶片通道内聚集，而是被冲散在各通道的叶顶区域。原因在于当两级动叶轴向间距较小时，在强烈动−动干涉的作用下，叶根区域的边界层分离被抑制，导致叶根分离区减小。在完全失速阶段，两级叶顶区域的扰动强度增大，扰动范围从叶顶沿径向扩展至70%叶高处。叶根的高熵区并未与叶顶的高熵区汇合，因此失速涡团类型属于多团部分叶高失速，这明显不同于风机在170 mm轴向间距下的失速涡团类型。由图15结合文献[20]可知，在70 mm轴向间距下，失速涡团先后在两级叶顶区域产生，前级产生的失速涡团可传播至前级叶轮的上游区域，导致上游1倍弦长内出现沿整周分布的高湍动能区，而后级产生的失速涡团对叶轮下游的影响范围较小，仅在叶轮尾缘附近存在高湍动能区。另外，受两级叶轮失速涡团的影响，两级叶轮之间也出现了高湍动能区。
![]()
图 14 轴向间距为70 mm时两级叶轮的静熵分布Figure 14. The static entropy distribution of the two-stage impellers under the axial spacing of 70 mm![]()
图 15 轴向间距为70 mm时不同叶高环面的湍动能分布Figure 15. Turbulent kinetic energy distribution of the different blade height sections under the axial spacing of 70 mm4. 结 论
1）2种轴向间距下的失速起始及其发展过程具有显著差异，失速起始的流量也明显不同。与轴向间距为70 mm时相比，当风机轴向间距为170 mm时，在更大的流量下即开始发生失速。
2）失速起始扰动的首发位置、类型及流动特征具有明显不同。当轴向间距为70 mm时，风机的失速起始扰动类型为“突尖型”，其首发于前级叶轮的叶顶区域，并出现前缘溢流和尾缘反流现象；当轴向间距为170 mm时，风机的失速起始扰动类型为“局部喘振型”，其首发于后级叶轮叶根吸力面的尾缘区域，并出现角区分离现象。
3）不同轴向间距是通过改变叶顶泄漏流的轴向动量及叶片吸力面径向涡流的强度来影响失速发展过程的。当轴向间距为70 mm时，失速涡团由叶顶泄漏流发展而来；而当轴向间距为170 mm时，失速涡团则是由叶片吸力面径向涡流发展而来。
4）在完全失速阶段，轴向间距对于失速涡团沿轴向、径向及周向的传播都具有显著影响。当轴向间距为70 mm时，失速涡团先后在两级叶轮内产生，沿周向分散于各叶片通道的叶顶区域，沿轴向向上、下游传播，沿径向表现为部分叶高失速；而当轴向间距为170 mm时，失速涡团由后级叶轮产生的径向涡流沿周向聚集而成，其转速为后级叶轮转速的33.3%，沿轴向传播范围也限于后级叶轮区域，沿径向表现为全叶高失速。
-
![]()
图 7 煤样C1、C2和C3压裂前后的三维重构模型
Figure 7. 3D reconstruction model of C1、C2 and C3 specimen before and after hydraulic fracturing
![]()
图 8 水力压裂前、后煤样的宏观裂隙图像
Figure 8. Image of macroscopic fracture of coal sample before and after hydraulic
![]()
图 9 水力压裂前后煤样的宏观裂隙分形维数线性拟合
Figure 9. Linear fitting of two-dimensional fractures before and after hydraulic
![]()
图 13 二维拓扑网络计算原理图和不同应力条件下二维分形维数变化率与拓扑参数的分布
Figure 13. Fracture networks in two-dimensional and distribution of 2D fractures and topological parameters of fractures under different stress
![]()
图 15 三维拓扑网络计算原理图和不同应力条件下三维分形维数变化率与拓扑参数的分布
Figure 15. Fracture networks in three-dimensional and distribution of 3D fractures and topological parameters of fractures under different stress
表 1 试样的物理力学性质
Table 1 Physical properties of samples
孔隙率/
%天然密度/
(g·m−3)抗拉强度/
MPa弹性模量/
GPa单轴抗压强度/
MPa6.021 1.756 1.743 5.49 10.43 
下载: 导出CSV
表 2 真三轴水力压裂试验参数
Table 2 True triaxial hydraulic fracturing test parameters
试件
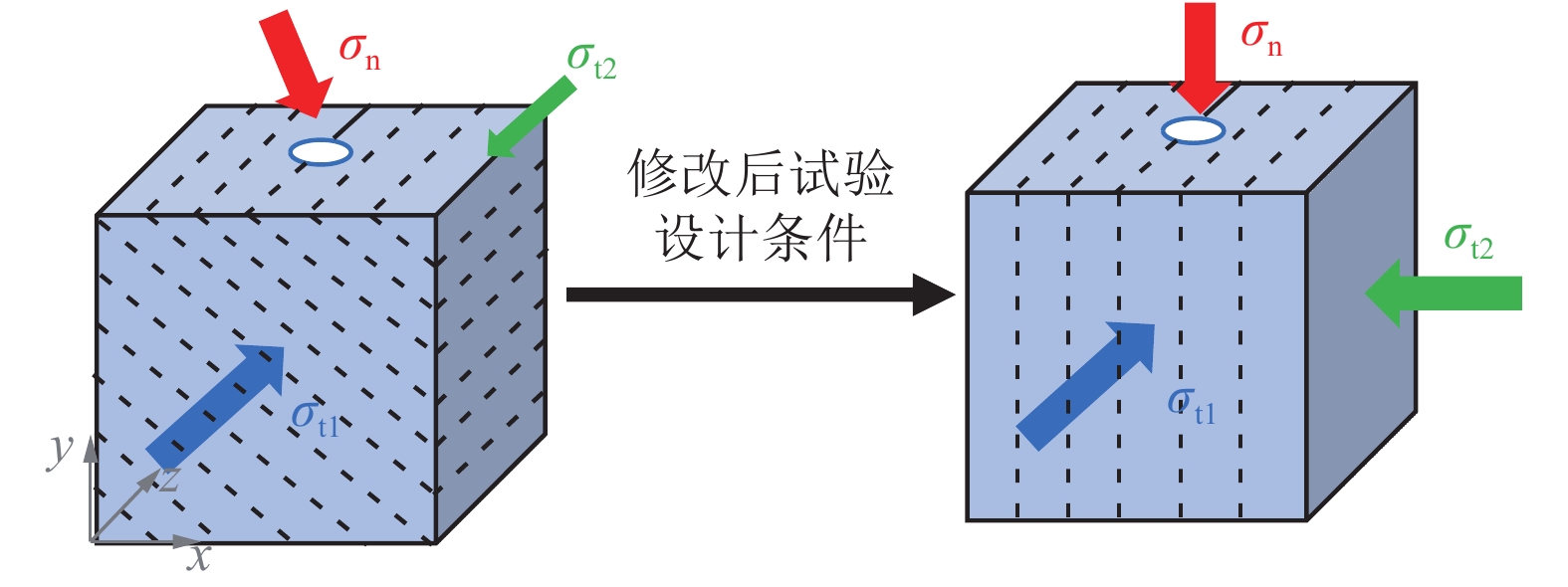
编号($ {\mathrm{\sigma }}_{\mathrm{t}1}/{\mathrm{\sigma }}_{\mathrm{n}}/{\mathrm{\sigma }}_{\mathrm{t}2} $)/
MPa垂向应力
差异系数水平应力
差异系数水平
应力比注入速度/
(mL·min−1)C1 25/40/20 1 0.25 1.25 60 C2 30/40/20 1 0.50 1.50 C3 35/40/20 1 0.75 1.75 注：垂向地应力差异系数$k_{\mathrm{v} }=\left(\sigma_{\rm{n}}-\sigma_{ {\rm{t} } 2}\right) / \sigma_{ {\rm{t} } 2}$；水平应力差异系数$k_{\mathrm{H}}=\left(\sigma_{{\rm{t}} 1}-\sigma_{{\rm{t}} 2}\right) / \sigma_{{\rm{t}} 2}$。
下载: 导出CSV
表 3 煤样裂缝的相关参数
Table 3 Relevant parameters of coal cracks
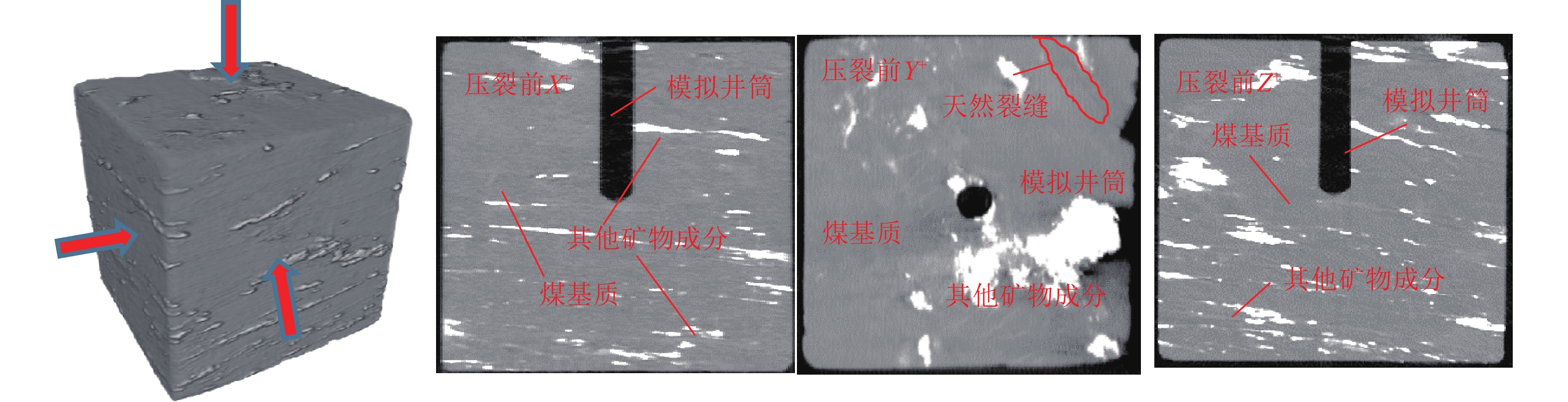
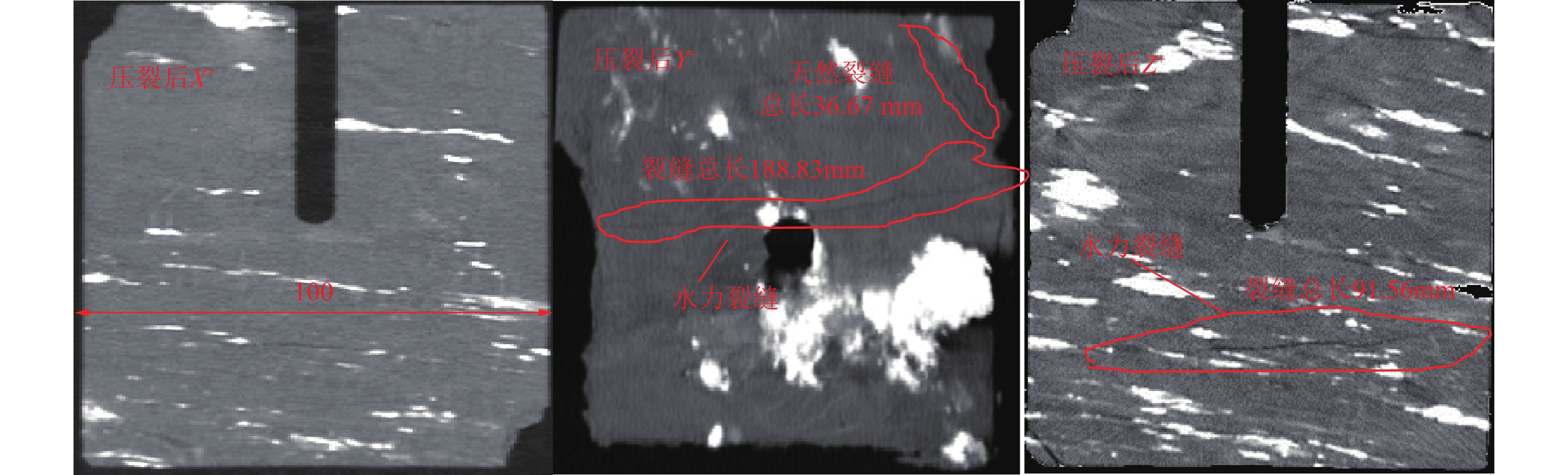
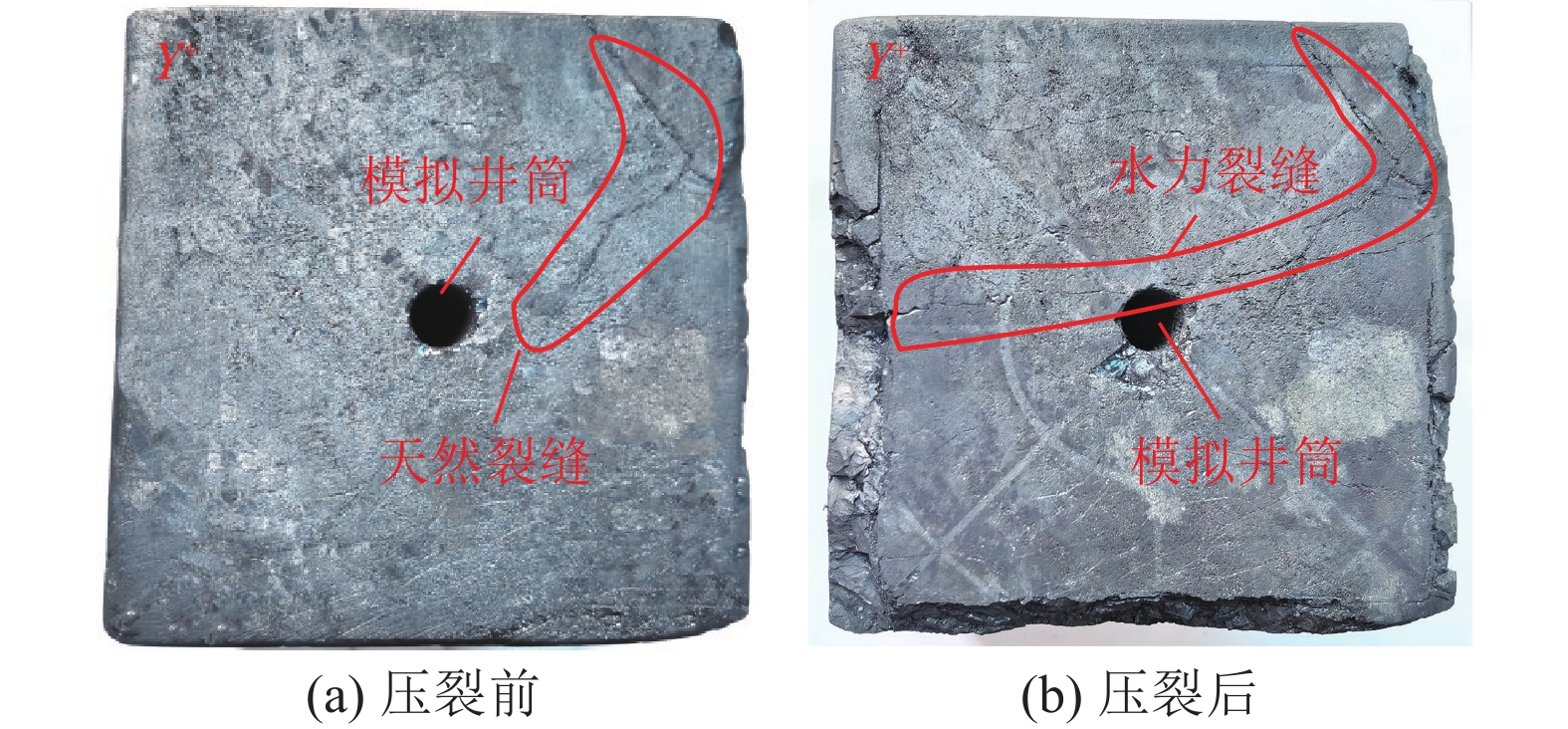
试件
编号压裂前裂缝数量 压裂后裂缝数量 压裂后裂缝形态 C1 0 7 主裂缝为弧形裂缝 C2 1 7 主裂缝为横向贯穿裂缝 C3 0 8 网状裂缝网络
下载: 导出CSV
表 4 压裂前后煤样的三维分形维数
Table 4 Three-dimensional fractal dimension of coal samples before and after hydraulic
试件编号 Da Db K/% 水平应力比 C1 2.167 72 2.24369 3.50 1.25 C2 2.206 16 2.31075 4.74 1.5 C3 2.155 92 2.35386 9.18 1.75
下载: 导出CSV
表 5 压裂前后煤样的二维拓扑参数
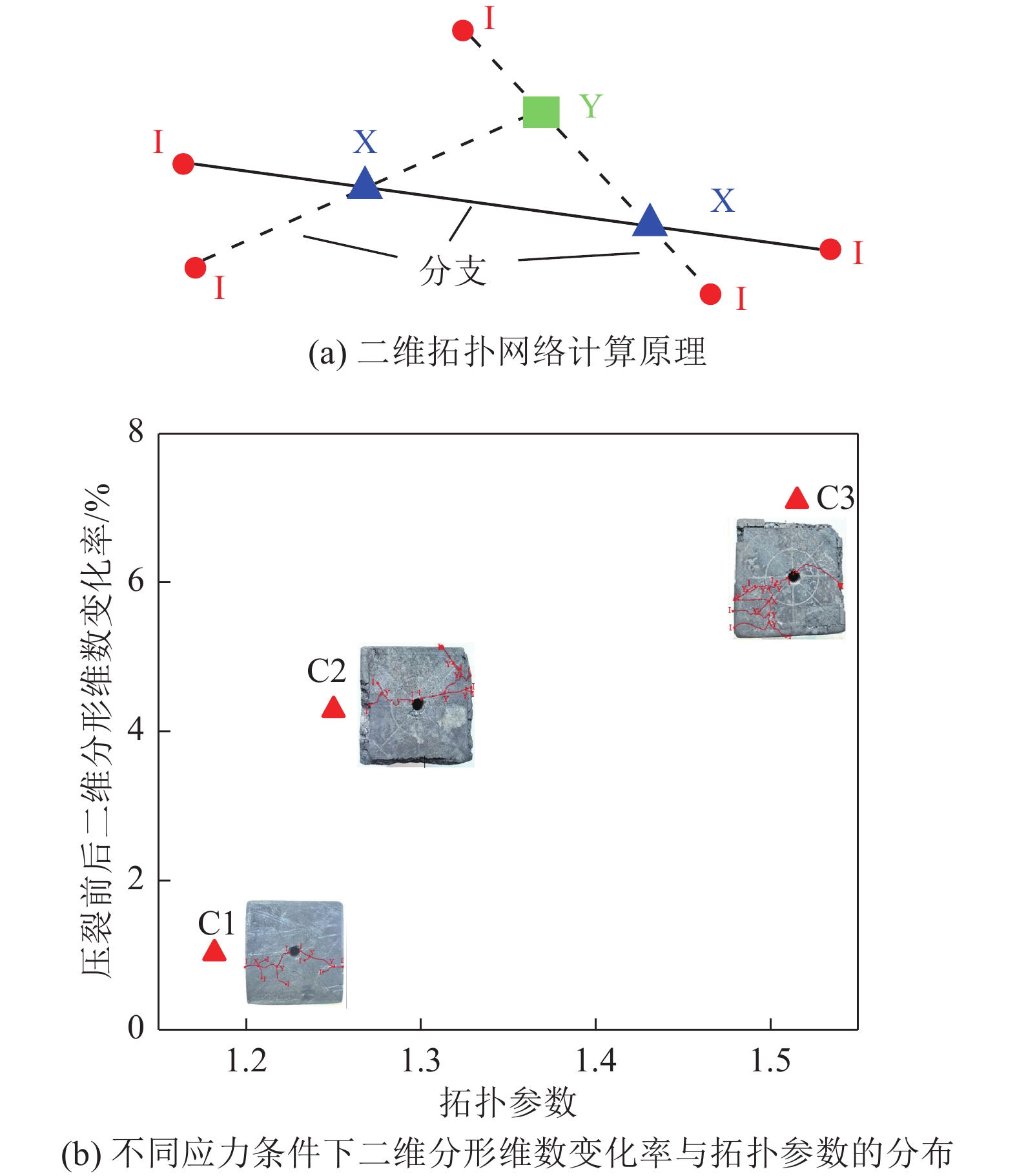
Table 5 Two dimensional topological parameters of coal samples be-fore and after hydraulic fracturing
试件
编号节点数量 Na Nb NI NX NY C1 9 1 3 11 1.18 C2 9 0 5 12 1.25 C3 8 1 7 16.5 1.52
下载: 导出CSV
表 6 压裂前后煤样的三维拓扑参数
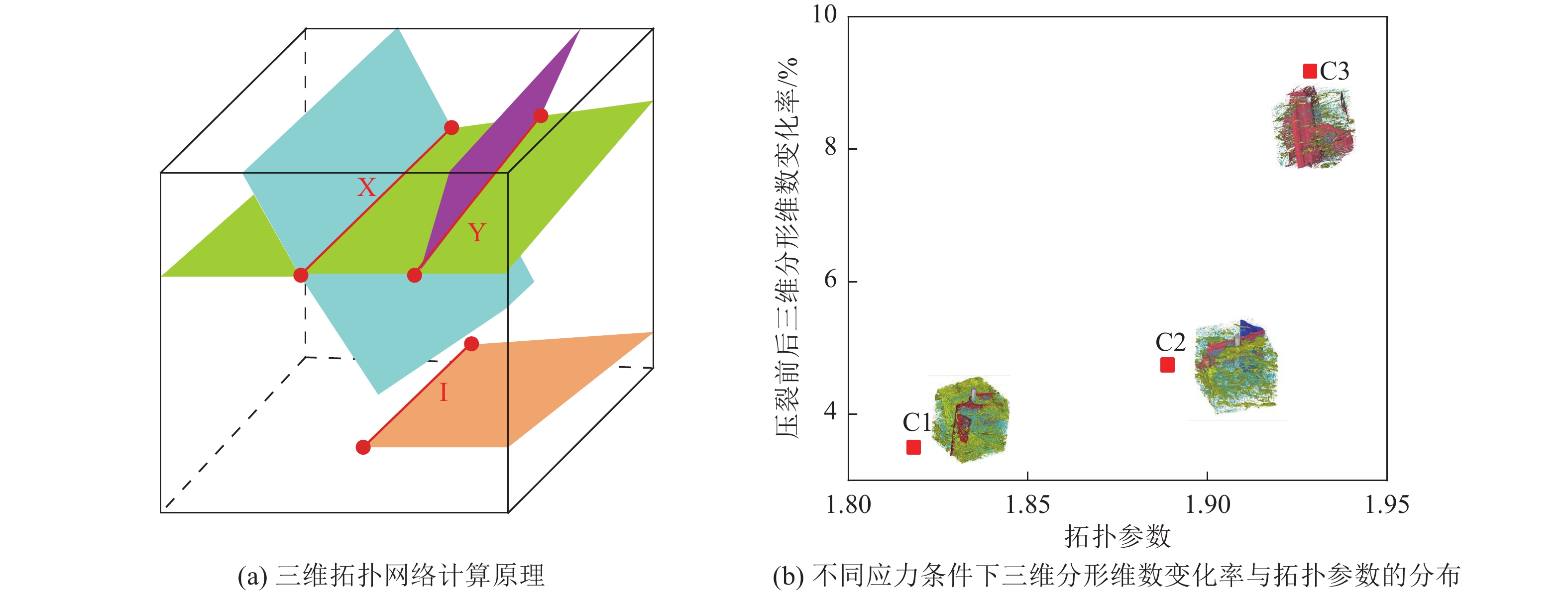
Table 6 Three-dimensional topological parameters of coal samples before and after hydraulic
试件
编号节点数量 Na Nb NI NX NY C1 1 1 2 5.5 1.82 C2 1 1 3 7 1.86 C3 1 3 5 14 1.93
下载: 导出CSV
-
[1] 何利文. 煤矿回采工作面瓦斯涌出非线性特性分析及预测仿真理论研究[D]. 长沙: 中南大学, 2010. HE liwen. Study on nonlinear characteristic analysis and prediction simulation theory of gas emission in coal mining face[D]. Changsha: Central South University, 2010.
[2] 蒋长宝,余 塘,段敏克,等. 瓦斯压力和应力对裂隙影响下的渗透率模型研究[J]. 煤炭科学技术,2021,49(2):115−121. JIANG Changbao,YU Tang,DUAN Minke,et al. Study on permea-bility model under the influence of gas pressure and stress on fracture[J]. Coal Science and Technology,2021,49(2):115−121.
[3] CAO Yunxing,ZHANG Junsheng,ZHAI Hong,et al. CO2 gas fracturing: a novel reservoir stimulation technology in low permeability gassy coal seams[J]. Fuel,2017,203:197−207. doi: 10.1016/j.fuel.2017.04.053
[4] QIN Lei,ZHAI Cheng,XU Jizhao,et al. Evolution of the pore structure in coal subjected to freeze−thaw using liquid nitrogen to enhance coalbed methane extraction[J]. Journal of Petroleum Science and Engineering,2019,175:129−139. doi: 10.1016/j.petrol.2018.12.037
[5] 张凤婕,吴 宇,茅献彪,等. 煤层气注热开采的热-流-固耦合作用分析[J]. 采矿与安全工程学报,2012,29(4):505−510. ZHANG Fengjie,WU Yu,MAO Xianbiao,et al. Coupled ther-mal-hydrological-mechanical analysis of exploiting coal me-thane by heat injection[J]. Journal of Mining & Safety Engineering,2012,29(4):505−510.
[6] LI Quanshu,XING Huilin,LIU Jianjun,et al. A review on hydraulic fracturing of unconventional reservoir[J]. Petroleum,2015,1(1):8−15. doi: 10.1016/j.petlm.2015.03.008
[7] ALEXANDRINE Gesret, MARK Noble, NICOLAS Desassis, et al. Microseismic monitoring-consequences of velocity model uncertainties on event location uncertainties[C]. //Paper presented at the 75th EAGE Conference & Exhibition incorporating SPE EUROPEC, United Kingdom, 2013.
[8] CRAIG L Cipolla, WENG Xiaowei, MARK Gavin Mack, et al. Integrating microseismic mapping and complex fracture modeling to charac-terize fracture complexity [C]. //Paper presented at the SPE Hy-draulic Fracturing Technology Conference, The Woodlands, Texas, USA, 2011: 1-22.
[9] ANISH Singh Chaudhary. Shale oil production performance from a stim-ulated reservoir volume[D]. Texas A&M University, 2011.
[10] PAVEL Zuloaga-Molerol,YU Wei,XU Yifei,et al. Simulation study of CO2-EOR in tight oil reservoirs with complex fracture geome-tries[J]. Scientific Reports,2016,6(1):1−11. doi: 10.1038/s41598-016-0001-8
[11] 高金栋,周立发,冯 乔,等. 储层构造裂缝识别及预测研究进展[J]. 地质科技情报,2018,37(4):158−166. GAO Jindong,ZHOU Lifa,FENG Qiao,et al. Research progress of reservoir structural fracture identification and prediction[J]. Geological Science and Technology Information,2018,37(4):158−166.
[12] DAVID J. Sanderson, CASEY W. Nixon. The use of topology in fracture network characterization[J]. Journal of Structural Geology,2015,72:55−66. doi: 10.1016/j.jsg.2015.01.005
[13] CARDONA R,JENNER E,DAVIS T L. Fracture network characterization from P- and S-wave data at Weyburn field, Canada[J]. Seg Expanded Ab-stracts,2003(1):370.
[14] 赵海燕,宫伟力. 基于图像分割的煤岩割理CT图像各向异性特征[J]. 煤田地质与勘探,2009,37(6):14−18. ZHAO Haiyan,GONG Weili. Anisotropic characteristics of coal and rock cleat CT image based on image segmentation[J]. Coal Geology & Exploration,2009,37(6):14−18.
[15] 康天合,赵阳升,靳钟铭. 煤体裂隙尺度分布的分形研究[J]. 煤炭学报,1995,20(4):393−398. doi: 10.3321/j.issn:0253-9993.1995.04.019 KANG Tianhe,ZHAO Yangsheng,JIN Zhongming. Fractal study on scale distribution of coal fractures[J]. Journal of China Coal Society,1995,20(4):393−398. doi: 10.3321/j.issn:0253-9993.1995.04.019
[16] FU Xuehai,QIN Yong,ZHANG Wanhong,et al. Fractal classification and natural classification of coal pore structure based on migration of coal bed methane[J]. Chinese Science Bulletin,2005,50:66−71.
[17] CAO Taotao,SONG Zhiguang,WANG Sibo,et al. Characterization of pore structure and fractal dimension of Paleozoic shales from the northeastern Sichuan Basin, China[J]. Journal of Natural Gas Science and Engineering,2016,35:882−895. doi: 10.1016/j.jngse.2016.09.022
[18] XIA Yuxuan,CAI Jianchao,WEI Wei,et al. A new method for calculating fractal dimensions of porous media based on pore size distribu-tion[J]. Fractals,2018,26(1):1850006. doi: 10.1142/S0218348X18500068
[19] 李廷芥,王耀辉,张梅英,等. 岩石裂纹的分形特性及岩爆机理研究[J]. 岩石力学与工程学报,2000,19(1):6−10. LI Tingjie,WANG Yaohui,ZHANG Meiying,et al. Fractal properties of crack in rock and mechanism of rock-burst[J]. Chinese Journal of Rock Mechanics and Engineering,2000,19(1):6−10.
[20] LIU Xianfeng,NIE Baisheng. Fractal characteristics of coal samples utilizing image analysis and gas adsorption[J]. Fuel,2016,182:314−322. doi: 10.1016/j.fuel.2016.05.110
[21] JIANG Changbao,NIU Bingwei,YIN Guangzhi,et al. CT-based 3D re-construction of the geometry and propagation of hydraulic frac-turing in shale[J]. Journal of Petroleum Science and Engineering,2019,179:899−911. doi: 10.1016/j.petrol.2019.04.103
[22] HE Qiang,HE Bo,LI Fengxia,et al. Fractal characterization of complex hydraulic fractures in oil shale via topology[J]. Energies,2021,14(4):1123−1141. doi: 10.3390/en14041123
[23] LIU Peng,JU Yang,GAO Feng,et al. CT identification and fractal char-acterization of 3D propagation and distribution of hydrofracturing cracks in low-permeability heterogeneous rocks[J]. Journal of Geophysical Research:Solid Earth,2018,123(3):2156−2173. doi: 10.1002/2017JB015048
[24] LIU Peng,JU Yang,PATHEGAMA G Ranjith,et al. Experimental investi-gation of the effects of heterogeneity and geostress difference on the 3D growth and distribution of hydrofracturing cracks in uncon-ventional reservoir rocks[J]. Journal of Natural Gas Science and Engineering,2016,35:541−554. doi: 10.1016/j.jngse.2016.08.071
[25] JU Yang,HUANG Yaohui,SU Sun,et al. Three-dimensional numerical reconstruction method for irregular structures of granular geo-materials[J]. Geomechanics and Geophysics for Geo-Energy and Geo-Resources,2018,4:327−341. doi: 10.1007/s40948-018-0089-3
[26] PENG Ruidong,YANG Yancong,JU Yang,et al. Computation of fractal dimension of rock pores based on gray CT images[J]. Chinese Science Bulletin,2011,56(31):3346−3357. doi: 10.1007/s11434-011-4683-9
[27] DUAN Minke,JIANG Changbao,GAN Quan,et al. Study on permeability anisotropy of bedded coal under true triaxial stress and its appli-cation[J]. Transport in Porous Media,2020,131:1007−1035. doi: 10.1007/s11242-019-01375-y
[28] JU Yang,ZHENG Jiangtao,MARCELO Epstein,et al. 3D numerical recon-struction of well-connected porous structure of rock using fractal algorithms[J]. Computer Methods in Applied Mechanics & En-gineering,2014,279:212−226.
[29] ANWAR S Al-Kharusi,MARTIN J Blunt. Network extraction from sandstone and carbonate pore space images[J]. Journal of Petroleum Science and Engineering,2007,56(4):219−231. doi: 10.1016/j.petrol.2006.09.003
[30] LI Minghui,YIN Guangzhi,XU Jiang,et al. A novel true triaxial apparatus to study the geomechanical and fluid flow aspects of energy exploitations in geological formations[J]. Rock Me-chanics and Rock Engineering,2016,49:4647−4659. doi: 10.1007/s00603-016-1060-7
[31] 袁 亮. 深部采动响应与灾害防控研究进展[J]. 煤炭学报,2021,46(3):716−725. YUAN Liang. Research progress of deep mining response and disaster prevention and control[J]. Journal of China Coal Society,2021,46(3):716−725.
[32] JANG Changbao,LIU Xiaodong,WANG Wengsong,et al. Three-dimensional visualization of the evolution of pores and fractures in reservoir rocks under triaxial stress[J]. Powder Technology,2021,378:585−592. doi: 10.1016/j.powtec.2020.10.013
[33] AI Ting,ZHANG Ru,ZHOU Hongwei,et al. Box-counting methods to directly estimate the fractal dimension of a rock surface[J]. Applied Surface Science,2014,314:610−621. doi: 10.1016/j.apsusc.2014.06.152
[34] WU Mingyang,WANG Wensong,SHI Di,et al. Improved box-counting methods to directly estimate the fractal dimension of a rough surface[J]. Measurement,2021,177:109303. doi: 10.1016/j.measurement.2021.109303
[35] CHINMAYA Panigrahy,AYAN Seal,NIHAR Kumar Mahato,et al. Differential box counting methods for estimating fractal dimension of gray-scale images: a survey[J]. Chaos, Solitons and Fractals,2019,126:178−202. doi: 10.1016/j.chaos.2019.06.007
[36] 莫海涛,郝世俊,赵江鹏. 煤矿区地面大直径钻孔成孔关键技术与装备[J]. 煤炭科学技术,2021,49(5):190−197. MO Haitao,HAO Shijun,ZHAO Jiangpeng. Key technology and equipment of hole-forming for surface large diameter borehole in coal mine area[J]. Coal Science and Technology,2021,49(5):190−197.
[37] 李波波,王斌,杨康,等. 煤岩孔裂隙结构分形特征及渗透率模型研究[J]. 煤炭科学技术,2021,49(2):226−231. LI Bobo,WANG Bin,YANG Kang,et al. Study on fractal characteristics of coal and rock pore fissure structure and permeability model[J]. Coal Science and Technology,2021,49(2):226−231.
-
期刊类型引用(3)
1. 陈庆光，石晓杰，李凯伦，徐金. 矿用对旋主通风机周向槽机匣处理扩稳机理研究. 矿业安全与环保. 2024(05): 105-111 . 
百度学术
2. 史勋勋. 煤矿FBDNo8.0型对旋风机计算流体力学特性分析. 机械管理开发. 2024(11): 57-59 . 百度学术
3. 陈永平，刘荣华，陈世强，刘东，李洋溢，王潇. 对旋轴流通风机叶轮内能量传递过程与演变特性. 煤炭科学技术. 2024(11): 356-367 . 本站查看
其他类型引用(1)
计量
- 文章访问数: 157
- HTML全文浏览量: 29
- PDF下载量: 89
- 被引次数: 4
